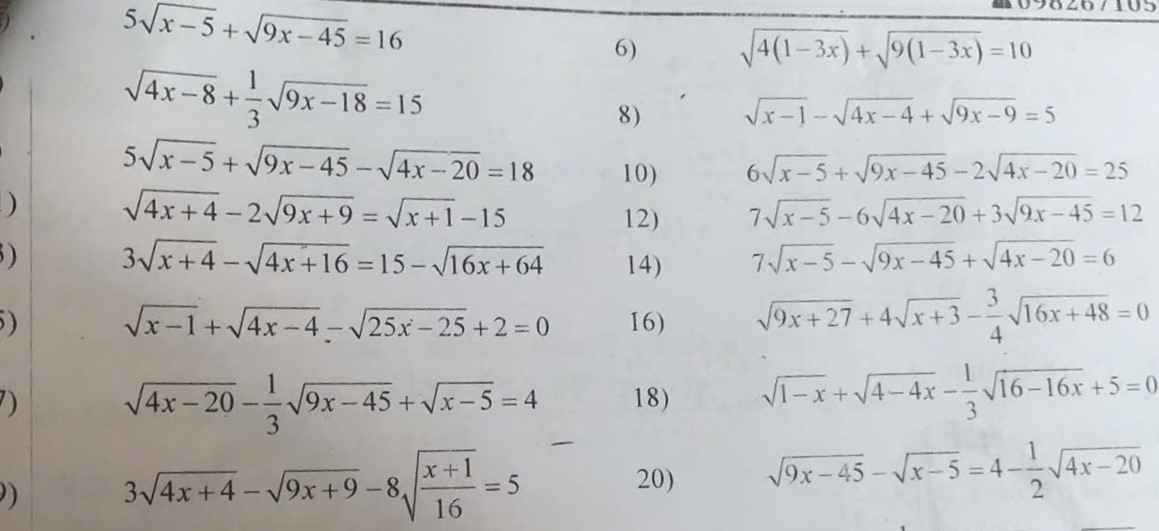5) \(5\sqrt{x-5}+\sqrt{9x-45}=16\Leftrightarrow5\sqrt{x-5}+\sqrt{9\left(x-5\right)}=16\Leftrightarrow5\sqrt{x-5}+3\sqrt{x-5}=16\Leftrightarrow8\sqrt{x-5}=16\Leftrightarrow\sqrt{x-5}=2\Leftrightarrow x-5=4\Leftrightarrow x=9\)
7)\(\sqrt{4x-8}+\dfrac{1}{3}\sqrt{9x-18}=15\Leftrightarrow\sqrt{4\left(x-2\right)}+\dfrac{1}{3}\sqrt{9\left(x-2\right)}=15\Leftrightarrow2\sqrt{x-2}+\sqrt{x-2}=15\Leftrightarrow3\sqrt{x-2}=15\Leftrightarrow\sqrt{x-2}=5\Leftrightarrow x-2=25\Leftrightarrow x=27\)6) \(\sqrt{4\left(1-3x\right)}+\sqrt{9\left(1-3x\right)}=10\Leftrightarrow2\sqrt{1-3x}+3\sqrt{1-3x}=10\Leftrightarrow5\sqrt{1-3x}=10\Leftrightarrow\sqrt{1-3x}=2\Leftrightarrow1-3x=4\Leftrightarrow x=-1\)
8) \(\sqrt{x-1}-\sqrt{4x-4}+\sqrt{9x-9}=5\Leftrightarrow\sqrt{x-1}-\sqrt{4\left(x-1\right)}+\sqrt{9\left(x-1\right)}=5\Leftrightarrow\sqrt{x-1}-2\sqrt{x-1}+3\sqrt{x-1}=5\Leftrightarrow2\sqrt{x-1}=5\Leftrightarrow\sqrt{x-1}=\dfrac{5}{2}\Leftrightarrow x-1=\dfrac{25}{4}\Leftrightarrow x=\dfrac{29}{4}\)
11: ĐKXĐ: x>=-1
\(\sqrt{4x+4}-2\sqrt{9x+9}=\sqrt{x+1}-15\)
=>\(2\sqrt{x+1}-2\cdot3\sqrt{x+1}-\sqrt{x+1}=-15\)
=>\(-5\sqrt{x+1}=-15\)
=>\(\sqrt{x+1}=3\)
=>x+1=9
=>x=9-1=8(nhận)
12: ĐKXĐ: x>=5
\(7\sqrt{x-5}-6\sqrt{4x-20}+3\sqrt{9x-45}=12\)
=>\(7\sqrt{x-5}-6\cdot2\sqrt{x-5}+3\cdot3\sqrt{x-5}=12\)
=>\(4\sqrt{x-5}=12\)
=>\(\sqrt{x-5}=3\)
=>x-5=9
=>x=9+5=14(nhận)
13: ĐKXĐ: x>=-4
\(3\sqrt{x+4}-\sqrt{4x+16}=15-\sqrt{16x+64}\)
=>\(3\sqrt{x+4}-2\sqrt{x+4}+4\sqrt{x+4}=15\)
=>\(5\sqrt{x+4}=15\)
=>\(\sqrt{x+4}=3\)
=>\(x+4=3^2=9\)
=>x=9-4=5(nhận)
14: ĐKXĐ: x>=5
\(7\sqrt{x-5}-\sqrt{9x-45}+\sqrt{4x-20}=6\)
=>\(7\sqrt{x-5}-3\sqrt{x-5}+2\sqrt{x-5}=6\)
=>\(6\sqrt{x-5}=6\)
=>\(\sqrt{x-5}=1\)
=>x-5=1
=>x=6(nhận)
15: ĐKXĐ: x>=1
\(\sqrt{x-1}+\sqrt{4x-4}-\sqrt{25x-25}+2=0\)
=>\(\sqrt{x-1}+2\sqrt{x-1}-5\sqrt{x-1}+2=0\)
=>\(2-2\sqrt{x-1}=0\)
=>\(\sqrt{x-1}=1\)
=>x-1=1
=>x=2(nhận)
16: ĐKXĐ: x>=-3
\(\sqrt{9x+27}+4\sqrt{x+3}-\dfrac{3}{4}\cdot\sqrt{16x+48}=0\)
=>\(3\sqrt{x+3}+4\sqrt{x+3}-\dfrac{3}{4}\cdot4\sqrt{x+3}=0\)
=>\(4\sqrt{x+3}=0\)
=>\(\sqrt{x+3}=0\)
=>x+3=0
=>x=-3(nhận)
17: ĐKXĐ: x>=5
\(\sqrt{4x-20}-\dfrac{1}{3}\cdot\sqrt{9x-45}+\sqrt{x-5}=4\)
=>\(2\sqrt{x-5}-\dfrac{1}{3}\cdot3\sqrt{x-5}+\sqrt{x-5}=4\)
=>\(2\sqrt{x-5}=4\)
=>\(\sqrt{x-5}=2\)
=>\(x-5=2^2=4\)
=>x=4+5=9(nhận)
18: ĐKXĐ: x<=1
\(\sqrt{1-x}+\sqrt{4-4x}-\dfrac{1}{3}\cdot\sqrt{16-16x}+5=0\)
=>\(\sqrt{1-x}+2\sqrt{1-x}-\dfrac{1}{3}\cdot4\sqrt{1-x}+5=0\)
=>\(3\sqrt{1-x}-\dfrac{4}{3}\sqrt{1-x}=-5\)
=>\(\dfrac{5}{3}\sqrt{1-x}=-5\)
=>\(\sqrt{1-x}=-5:\dfrac{5}{3}=-3\)(vô lý)
=>Phương trình vô nghiệm
19: ĐKXĐ: x>=-1
\(3\sqrt{4x+4}-\sqrt{9x+9}-8\sqrt{\dfrac{x+1}{16}}=5\)
=>\(3\cdot2\sqrt{x+1}-3\sqrt{x+1}-8\cdot\dfrac{\sqrt{x+1}}{4}=5\)
=>\(6\sqrt{x+1}-3\sqrt{x+1}-2\sqrt{x+1}=5\)
=>\(\sqrt{x+1}=5\)
=>\(x+1=5^2=25\)
=>x=25-1=24(nhận)
20: ĐKXĐ: x>=5
\(\sqrt{9x-45}-\sqrt{x-5}=4-\dfrac{1}{2}\cdot\sqrt{4x-20}\)
=>\(3\sqrt{x-5}-\sqrt{x-5}=4-\dfrac{1}{2}\cdot2\sqrt{x-5}\)
=>\(3\sqrt{x-5}=4\)
=>\(\sqrt{x-5}=\dfrac{4}{3}\)
=>\(x-5=\left(\dfrac{4}{3}\right)^2=\dfrac{16}{9}\)
=>\(x=\dfrac{16}{9}+5=\dfrac{16}{9}+\dfrac{45}{9}=\dfrac{61}{9}\left(nhận\right)\)