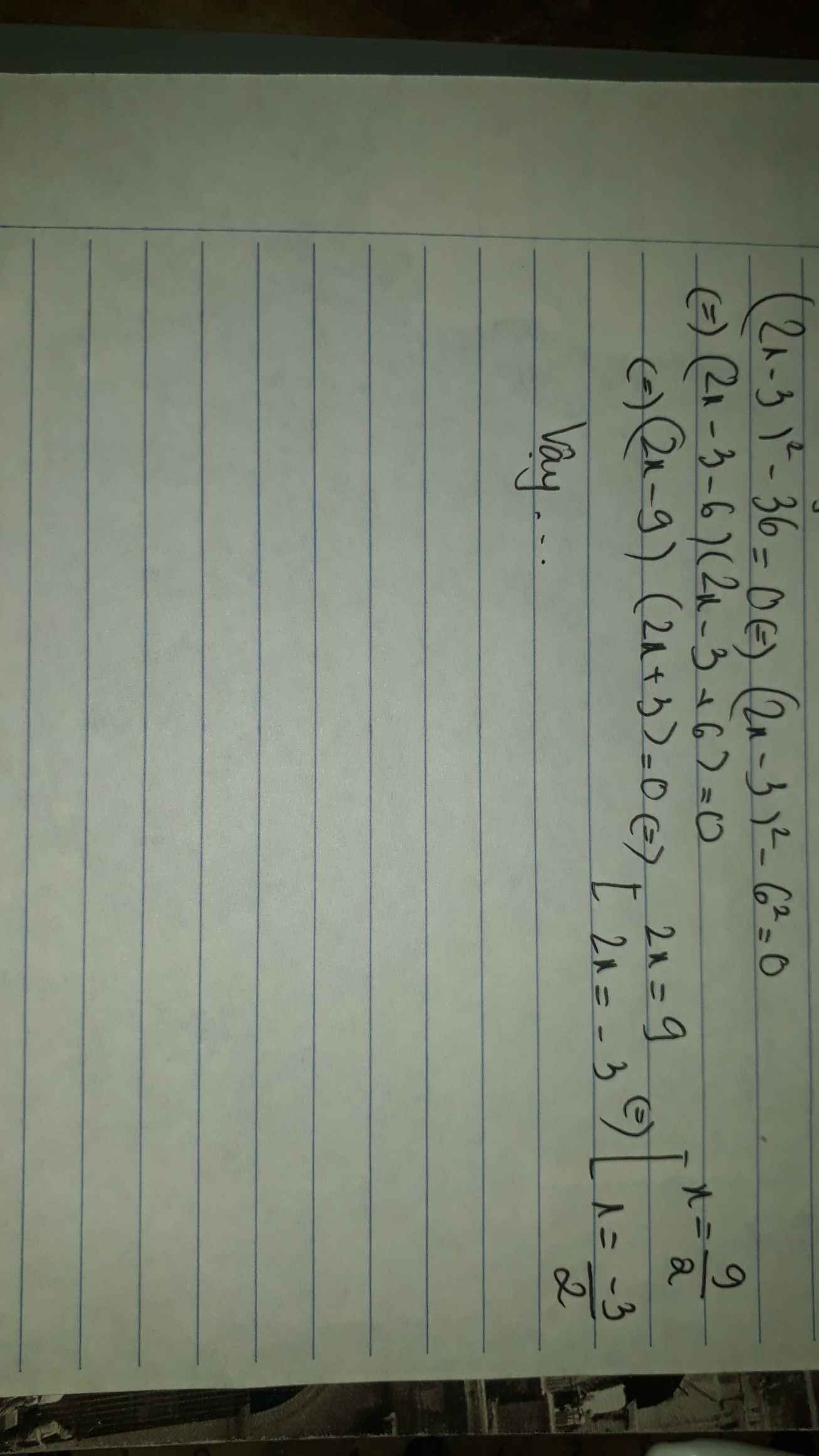\(\left(2x-3\right)^2-36=0\Leftrightarrow\left(2x-3-6\right)\left(2x-3+6\right)=0\Leftrightarrow\left(2x-9\right)\left(2x+3\right)=0\)\(\Leftrightarrow\left[{}\begin{matrix}2x=9\\2x=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{9}{2}\\x=-\dfrac{3}{2}\end{matrix}\right.\)
`(2x-3)^{2}-36=0`
`<=>(2x-3)^{2}-6^{2}=0`
`<=>(2x-3-6)(2x-3+6)=0`
`<=>(2x-9)(2x+3)=0`
`<=>2x-9=0` hay `2x+3=0`
`<=>x=9/2` hay `x=-3/2`
Ta có: \(\left(2x-3\right)^2-36=0\)
\(\Leftrightarrow\left(2x-9\right)\left(2x+3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{9}{2}\\x=-\dfrac{3}{2}\end{matrix}\right.\)
(2x - 3)2 - 36 = 0
<=> (2x - 3)2 - 62 = 0
<=> (2x - 3 + 6)(2x - 3 - 6) = 0
<=> \(\left[{}\begin{matrix}2x-3+6=0\\2x-3-6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1,5\\x=4,5\end{matrix}\right.\)
Vậy nghiệm của PT là S=\(\left\{-1,5;4,5\right\}\)