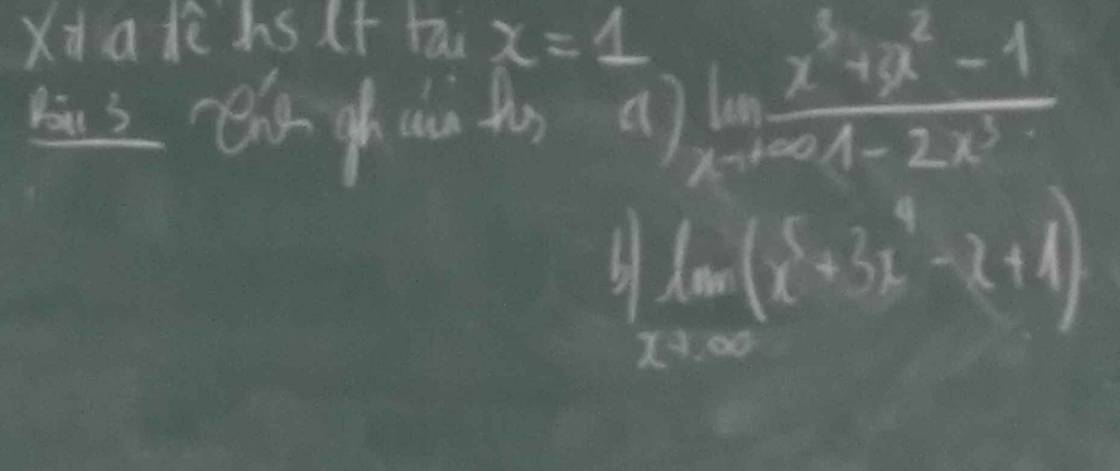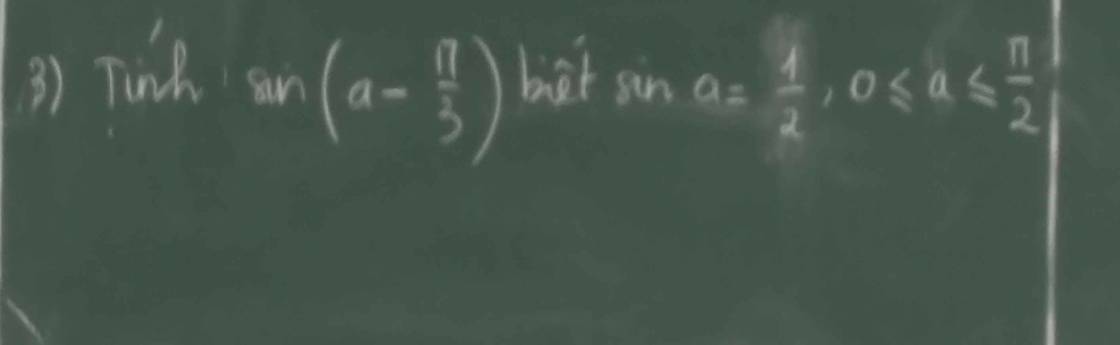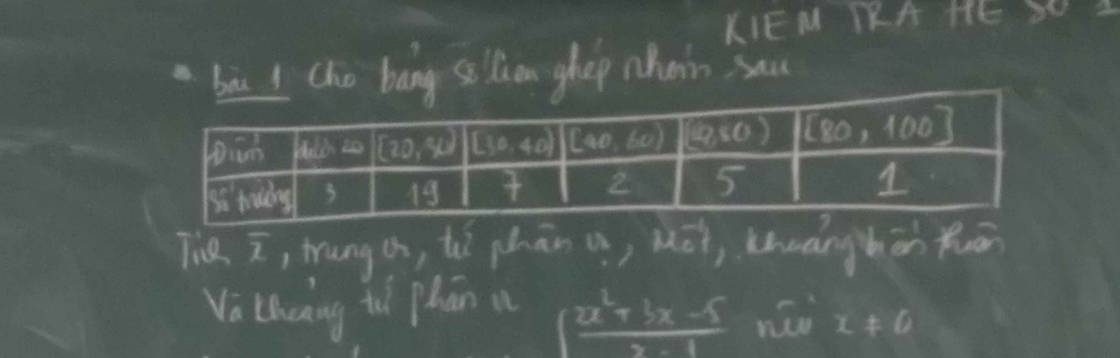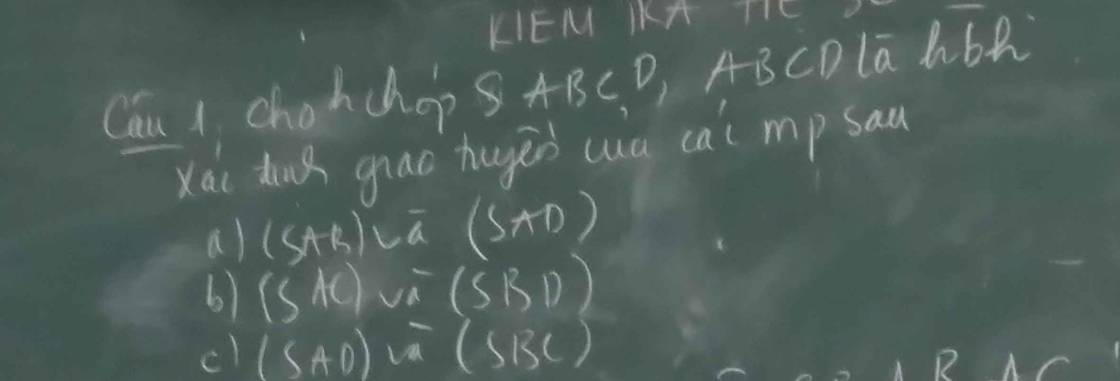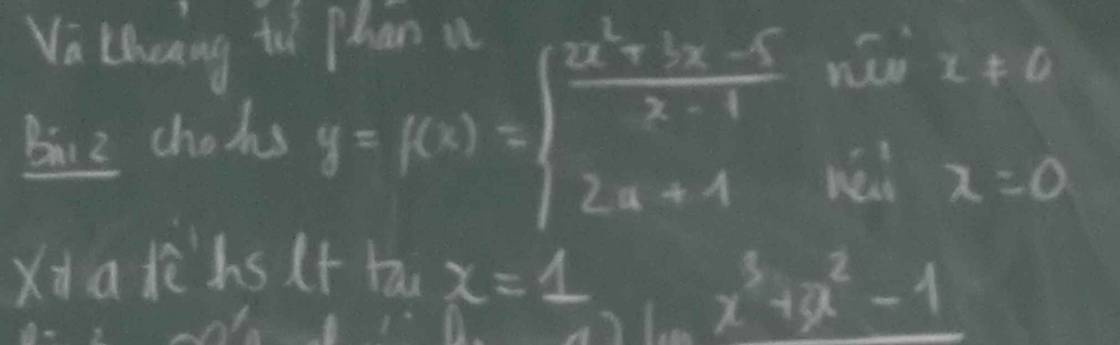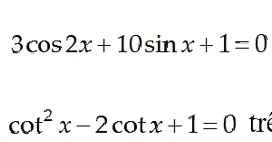a: \(\lim\limits_{x\rightarrow+\infty}\dfrac{x^3+x^2-1}{-2x^3+1}\)
\(=\lim\limits_{x\rightarrow+\infty}\dfrac{1+\dfrac{1}{x}-\dfrac{1}{x^3}}{-2+\dfrac{1}{x^3}}=\dfrac{1}{-2}=-\dfrac{1}{2}\)
b: \(\lim\limits_{x\rightarrow-\infty}\left(x^5+3x^4-x+1\right)\)
\(=\lim\limits_{x\rightarrow-\infty}\left[x^5\left(1+\dfrac{3}{x}-\dfrac{1}{x^4}+\dfrac{1}{x^5}\right)\right]\)
\(=-\infty\) vì \(\left\{{}\begin{matrix}\lim\limits_{x\rightarrow-\infty}x^5=-\infty\\\lim\limits_{x\rightarrow-\infty}1+\dfrac{3}{x}-\dfrac{1}{x^4}+\dfrac{1}{x^5}=1>0\end{matrix}\right.\)