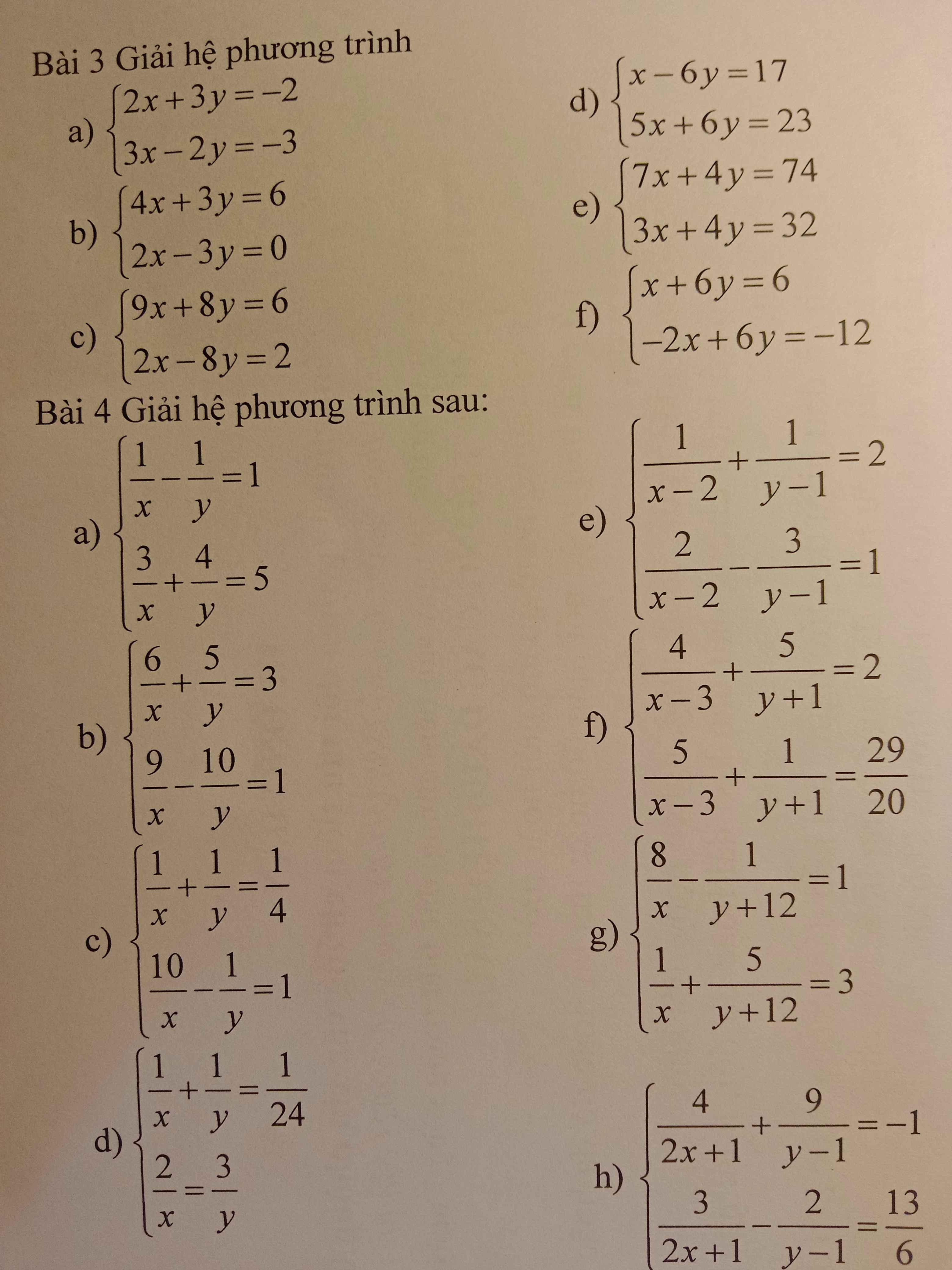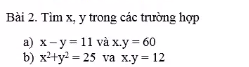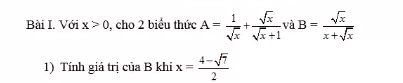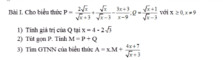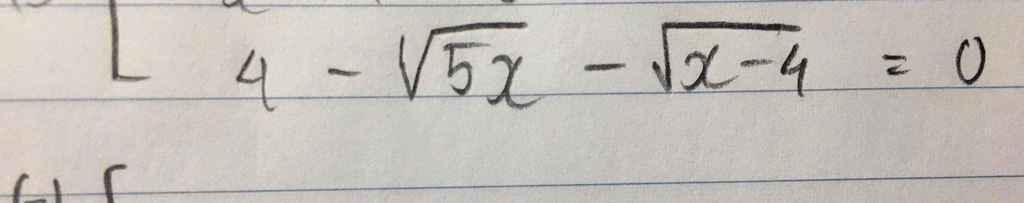Bài 4:
a) \(A=\left(\dfrac{1}{x+2\sqrt{x}}-\dfrac{1}{\sqrt{x}+2}\right):\dfrac{1-\sqrt{x}}{x+4\sqrt{x}+4}\)
\(=\left[\dfrac{1}{\sqrt{x}\left(\sqrt{x}+2\right)}-\dfrac{1}{\sqrt{x}+2}\right]:\dfrac{1-\sqrt{x}}{\left(\sqrt{x}+2\right)^2}\)
\(=\dfrac{1-\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+2\right)}:\dfrac{1-\sqrt{x}}{\left(\sqrt{x}+2\right)^2}\)
\(=\dfrac{1-\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+2\right)}.\dfrac{\left(\sqrt{x}+2\right)^2}{1-\sqrt{x}}\)
\(=\dfrac{\sqrt{x}+2}{\sqrt{x}}\)
b) Yêu cầu bài toán: \(\dfrac{\sqrt{x}+2}{\sqrt{x}}< \dfrac{5}{3}\)
\(\Leftrightarrow\dfrac{\sqrt{x}+2}{\sqrt{x}}-\dfrac{5}{3}< 0\)
\(\Leftrightarrow\dfrac{3\left(\sqrt{x}+2\right)}{3\sqrt{x}}-\dfrac{5\sqrt{x}}{3\sqrt{x}}< 0\)
\(\Leftrightarrow\dfrac{3\sqrt{x}+6-5\sqrt{x}}{3\sqrt{x}}< 0\)
\(\Leftrightarrow\dfrac{6-2\sqrt{x}}{3\sqrt{x}}< 0\)
\(\Leftrightarrow6-2\sqrt{x}< 0\) (vì \(3\sqrt{x}>0,\forall x\))
\(\Leftrightarrow2\sqrt{x}>6\)
\(\Leftrightarrow\sqrt{x}>3\)
\(\Leftrightarrow x>9\)
Vậy với \(x>9\) thì \(A< \dfrac{5}{3}.\)