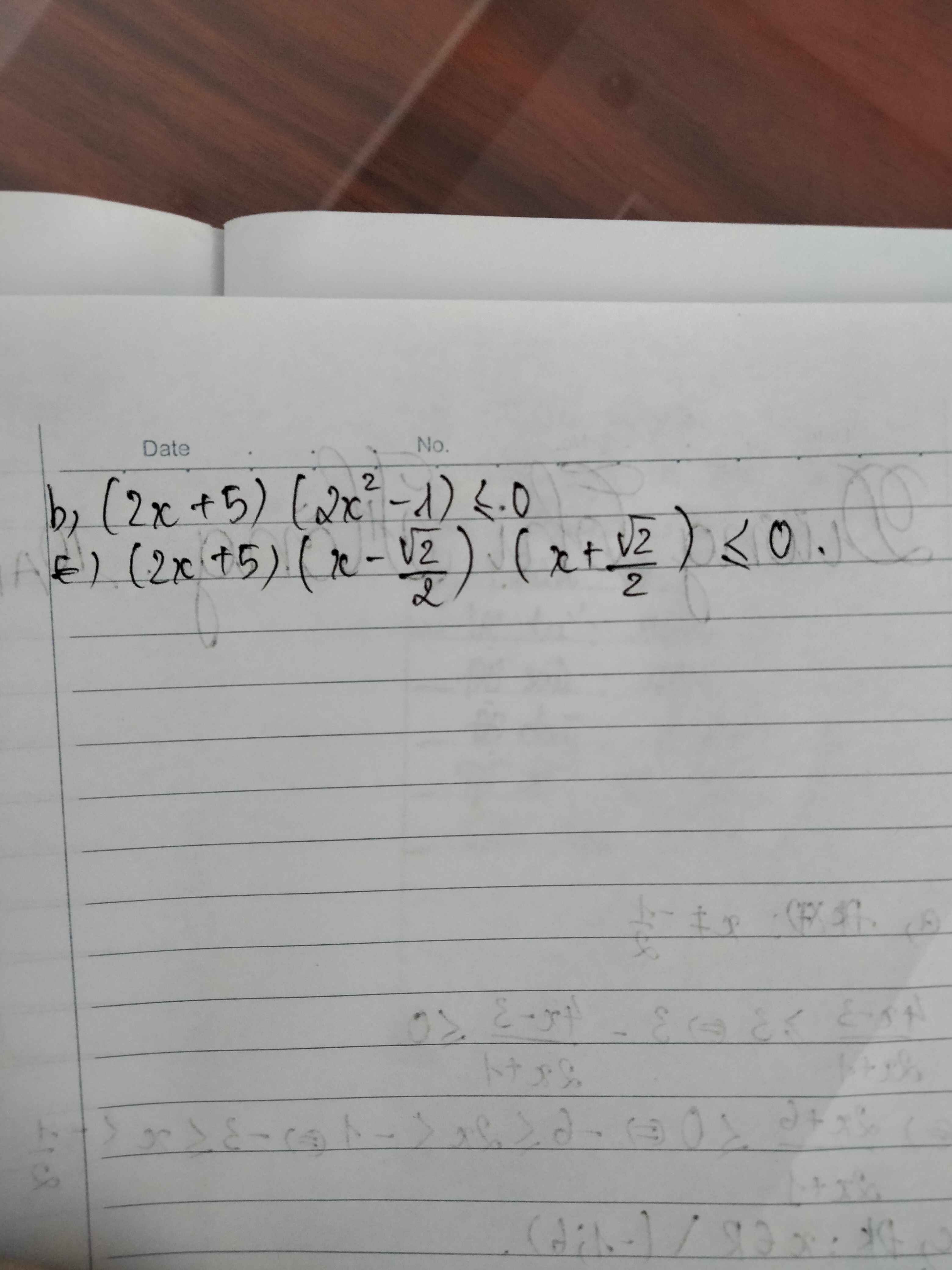a: =>\(\dfrac{x-x+2}{x\left(x-2\right)}-\dfrac{2}{x+2}< =0\)
=>\(\dfrac{2}{x\left(x-2\right)}-\dfrac{2}{x+2}< =0\)
=>\(\dfrac{2x+4-2x^2+4x}{x\left(x-2\right)\left(x+2\right)}< =0\)
=>\(\dfrac{-x^2+3x+4}{x\left(x-2\right)\left(x+2\right)}< =0\)
=>\(\dfrac{\left(x-4\right)\left(x+1\right)}{x\left(x-2\right)\left(x+2\right)}>=0\)
TH1: (x-4)(x+1)>=0 và x(x-2)(x+2)>0
=>(x>=4 hoặc x<=-1) và (-2<x<0 hoặc x>2)
=>x>=4 hoặc -2<x<=-1
TH2: (x-4)(x+1)<=0 và x(x^2-4)<0
=>(-1<=x<=4) và (x<-2 hoặc 0<x<2)
=>0<x<2
b: =>(2x-1)/(x-1)>2 hoặc (2x-1)/(x-1)<-2
=>(2x-1-2x+2)/(x-1)>0 hoặc (2x-1+2x-2)/(x-1)<0
=>1/(x-1)>0 hoặc (4x-3)/(x-1)<0
=>x>1 hoặc 3/4<x<1
c: =>\(\dfrac{x^2-5x+4-x^2+4}{x^2-4}>=0\)
=>\(\dfrac{-5x+8}{x^2-4}>=0\)
=>\(\dfrac{5x-8}{x^2-4}< =0\)
TH1: 5x-8>=0 và x^2-4<0
=>x>=8/5 và -2<x<2
=>Loại
TH2: 5x-8<=0 và x^2-4>0
=>x<=8/5 và (x>2 hoặc x<-2)
=>x<-2