e) \(\left(x^2-4\right)\left(x^2+4\right)=\left(x^2\right)^2-4^2=x^4-16\)
f) \(\left(x+y\right)^2+\left(x-y\right)^2=x^2+2xy+y^2+x^2-2xy+y^2=2x^2+2y^2\)
(x2 - 4)(x2 + 4)
Áp dụng hằng đẳng thức số 3, ta có:
<=> \(\left[\left(x^2\right)^2-4^2\right]\)
<=> (x4 - 16)
Mik làm chi tiết rồi, có gì ko hiểu bảo mik
e: \(\left(x^2-4\right)\left(x^2+4\right)=x^4-16\)
f: \(\left(x+y\right)^2+\left(x-y\right)^2=2x^2+2y^2\)

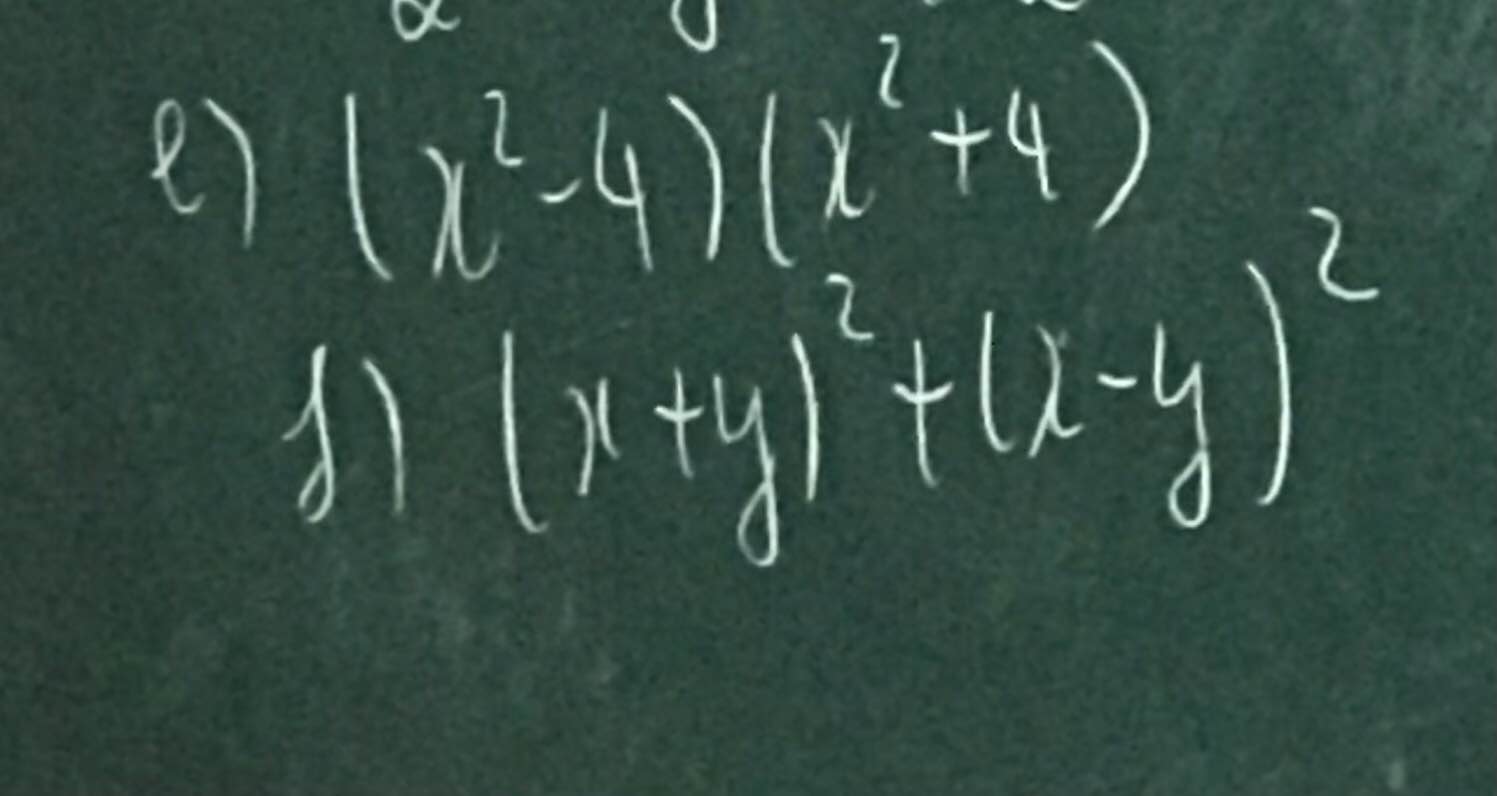







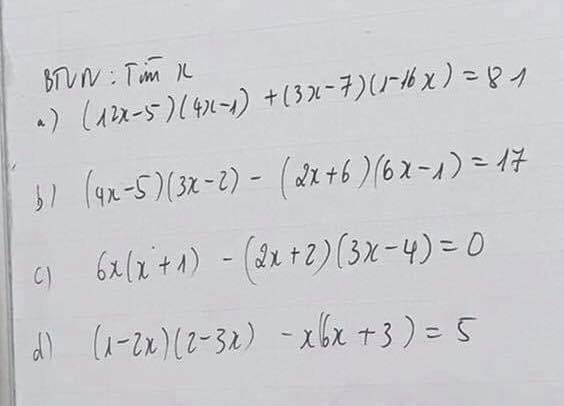

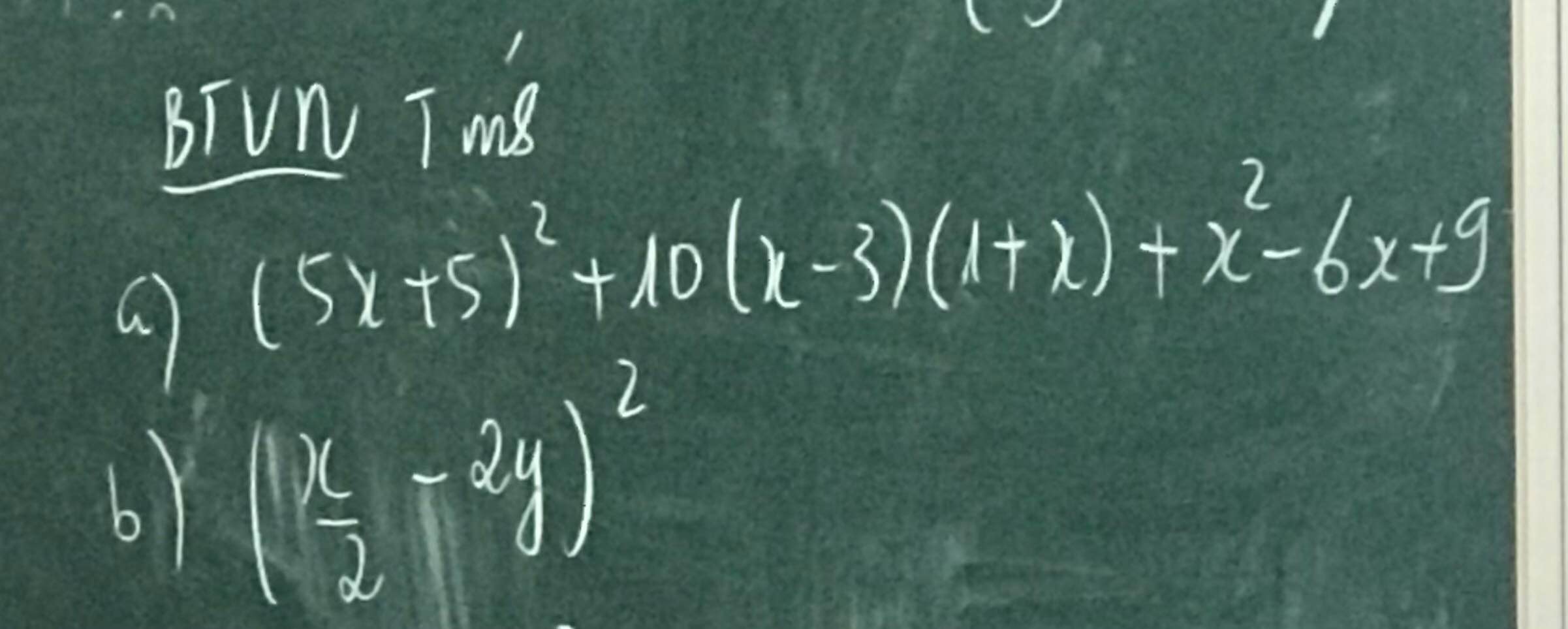

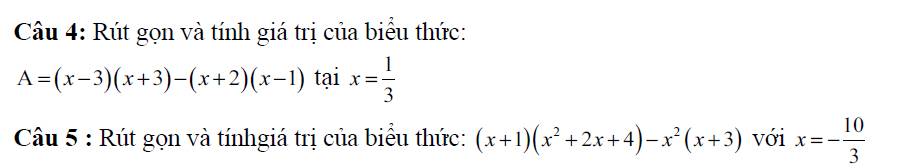 Giải chi tiết 2 bài với ạ. Cần gấp. Cảm ơn ạ.
Giải chi tiết 2 bài với ạ. Cần gấp. Cảm ơn ạ.




 em cần giải gấp bài 3 chi tiết mọi người giúp em với ạ. Làm bài dưới dạng phân số ạ em cần gấp
em cần giải gấp bài 3 chi tiết mọi người giúp em với ạ. Làm bài dưới dạng phân số ạ em cần gấp


