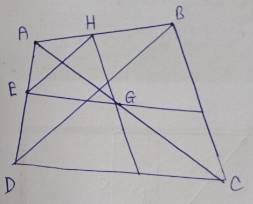
Các bước giải:
a) Vì EG // CD nên theo định lí Thalet ta có: \(\dfrac{AE}{AD}\) = \(\dfrac{AG}{AC}\)
Vì GH // CB nên theo định lí Thalet ta có: \(\dfrac{AG}{AC}\)= \(\dfrac{AH}{AB}\)
⇒ \(\dfrac{AG}{AC}\) = \(\dfrac{AH}{AB}\) ⇒ HE // BD (đpcm) (Thalet đảo)
b) HE // BD ⇒ \(\dfrac{AE}{AD}\) = \(\dfrac{AH}{AB}\)
⇒ \(\dfrac{AE}{AD-AE}\) = \(\dfrac{AH}{AB-AH}\)
⇒ \(\dfrac{AE}{DE}\) = \(\dfrac{AH}{BH}\)
⇒\(AE.BH=AH.DE\left(đpcm\right)\)

Các bước giải:
a) Vì EG // CD nên theo định lí Thalet ta có: \(\dfrac{AG}{AC}\)
Vì GH // CB nên theo định lí Thalet ta có: \(\dfrac{AG}{AC}\) = \(\dfrac{AE}{DE}\) = \(\dfrac{AH}{BH}\)
⇒ AE.BH = AH.DE











