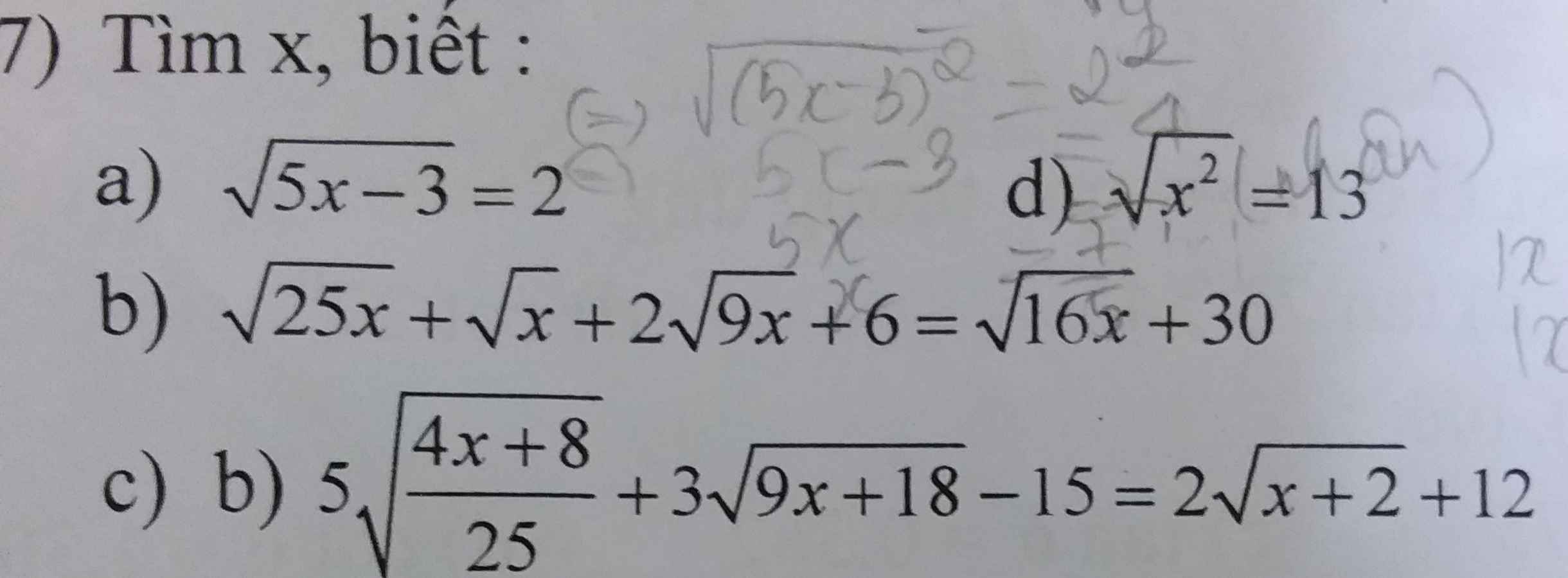\(A=\left(\dfrac{2\sqrt{x}}{\sqrt{x}+3}+\dfrac{\sqrt{x}}{\sqrt{x}-3}-\dfrac{3x+3}{x-9}\right):\left(\dfrac{2\sqrt{x}-2}{\sqrt{x}-3}-1\right)\left(x\ge0,x\ne9\right)\)
\(=\left(\dfrac{2\sqrt{x}}{\sqrt{x}+3}+\dfrac{\sqrt{x}}{\sqrt{x}-3}-\dfrac{3x+3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\right):\dfrac{2\sqrt{x}-2-\sqrt{x}+3}{\sqrt{x}-3}\)
\(=\dfrac{2\sqrt{x}\left(\sqrt{x}-3\right)+\sqrt{x}\left(\sqrt{x}+3\right)-3x-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}:\dfrac{\sqrt{x}+1}{\sqrt{x}-3}\)
\(=\dfrac{-3\sqrt{x}-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}.\dfrac{\sqrt{x}-3}{\sqrt{x}+1}=\dfrac{-3\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}.\dfrac{\sqrt{x}-3}{\sqrt{x}+1}\)
\(=-\dfrac{3}{\sqrt{x}+3}\)
\(A< -\dfrac{1}{2}\Rightarrow-\dfrac{3}{\sqrt{x}+3}< -\dfrac{1}{2}\Rightarrow\dfrac{3}{\sqrt{x}+3}-\dfrac{1}{2}>0\)
\(\Rightarrow\dfrac{6-\sqrt{x}-3}{2\left(\sqrt{x}+3\right)}>0\Rightarrow\dfrac{3-\sqrt{x}}{2\left(\sqrt{x}+3\right)}>0\) mà \(2\left(\sqrt{x}+3\right)>0\)
\(\Rightarrow3-\sqrt{x}>0\Rightarrow\sqrt{x}< 3\Rightarrow x< 9\Rightarrow0\le x< 9\)
đk: \(x\ge0,x\ne9\)
a, rút gọn được \(A=\dfrac{-3}{\sqrt{x}+3}\)
b, để \(A< -\dfrac{1}{2}< =>-\dfrac{3}{\sqrt{x}+3}< -\dfrac{1}{2}\)
\(< =>\dfrac{-3}{\sqrt{x}+3}+\dfrac{1}{2}< 0< =>\dfrac{-6+\sqrt{x}+3}{2\left(\sqrt{x}+3\right)}< 0\)
\(< =>\dfrac{\sqrt{x}-3}{2\left(\sqrt{x}+3\right)}< 0\)
\(< =>\sqrt{x}-3< 0< =>x< 3\)
kết hợp đk : \(=>0\le x< 3\) thì A<-1/2
a) Ta có: \(A=\left(\dfrac{2\sqrt{x}}{\sqrt{x}+3}+\dfrac{\sqrt{x}}{\sqrt{x}-3}-\dfrac{3x+3}{x-9}\right):\left(\dfrac{2\sqrt{x}-2}{\sqrt{x}-3}-1\right)\)
\(=\dfrac{2x-6\sqrt{x}+x+3\sqrt{x}-3x-3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}:\dfrac{2\sqrt{x}-2-\sqrt{x}+3}{\sqrt{x}-3}\)
\(=\dfrac{-3\sqrt{x}-3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\cdot\dfrac{\sqrt{x}-3}{\sqrt{x}+1}\)
\(=\dfrac{-1}{\sqrt{x}+3}\)
b) Để \(A< -\dfrac{1}{2}\) thì \(A+\dfrac{1}{2}< 0\)
\(\Leftrightarrow\dfrac{-1}{\sqrt{x}+3}+\dfrac{1}{2}< 0\)
\(\Leftrightarrow\dfrac{-2+\sqrt{x}+3}{2\sqrt{x}+6}< 0\)
\(\Leftrightarrow\sqrt{x}+1< 0\)(Vô lý)