Câu II:
a) \(\sqrt{2x-1}=\sqrt{5}\left(x\ge\dfrac{1}{2}\right)\)
\(\Leftrightarrow2x-1=5\)
\(\Leftrightarrow2x=5+1\)
\(\Leftrightarrow2x=6\)
\(\Leftrightarrow x=\dfrac{6}{2}\)
\(\Leftrightarrow x=3\left(tm\right)\)
b) \(6\sqrt{x-5}+\sqrt{9x-45}-2\sqrt{4x-20}=25\left(x\ge5\right)\)
\(\Leftrightarrow6\sqrt{x-5}+\sqrt{9\left(x-5\right)}-2\sqrt{4\left(x-5\right)}=25\)
\(\Leftrightarrow6\sqrt{x-5}+3\sqrt{x-5}-2\cdot2\sqrt{x-5}=25\)
\(\Leftrightarrow9\sqrt{x-5}-4\sqrt{x-5}=25\)
\(\Leftrightarrow5\sqrt{x-5}=25\)
\(\Leftrightarrow\sqrt{x-5}=5\)
\(\Leftrightarrow x-5=5^2\)
\(\Leftrightarrow x-5=25\)
\(\Leftrightarrow x=30\left(tm\right)\)
Bài III:
a:
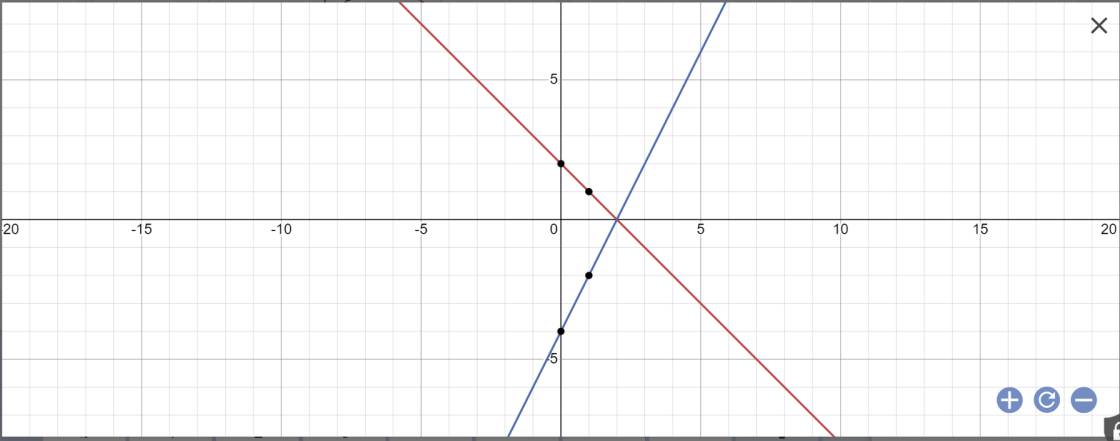
b: phương trình hoành độ giao điểm là:
2x-4=-x+2
=>2x+x=4+2
=>3x=6
=>x=6/3=2
Thay x=2 vào y=-x+2, ta được:
\(y=-2+2=0\)
Vậy: A(2;0)
c: Tọa độ B là:
\(\left\{{}\begin{matrix}x=0\\y=-x+2=-0+2=2\end{matrix}\right.\)
Vậy: B(0;2)
Tọa độ C là:
\(\left\{{}\begin{matrix}x=0\\y=2x-4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=2\cdot0-4=-4\end{matrix}\right.\)
Vậy: C(0;-4)
Ta có: A(2;0); B(0;2); C(0;-4)
\(AB=\sqrt{\left(0-2\right)^2+\left(2-0\right)^2}=2\sqrt{2}\)
\(AC=\sqrt{\left(0-2\right)^2+\left(-4-0\right)^2}=\sqrt{4^2+2^2}=2\sqrt{5}\)
\(BC=\sqrt{\left(0-0\right)^2+\left(-4-2\right)^2}=6\)
Xét ΔABC có \(cosBAC=\dfrac{AB^2+AC^2-BC^2}{2\cdot AB\cdot AC}=\dfrac{8+20-36}{2\cdot2\sqrt{2}\cdot2\sqrt{5}}=\dfrac{-8}{8\sqrt{5}}=-\dfrac{1}{\sqrt{5}}\)
=>\(sinBAC=\sqrt{1-cos^2BAC}=\sqrt{1-\dfrac{1}{5}}=\dfrac{2}{\sqrt{5}}\)
Diện tích tam giác ABC là:
\(S_{ABC}=\dfrac{1}{2}\cdot AB\cdot AC\cdot sinBAC\)
\(=\dfrac{1}{2}\cdot\dfrac{2}{\sqrt{5}}\cdot2\sqrt{2}\cdot2\sqrt{5}=4\sqrt{2}\)












 ai giúp em bài này với ạ 🥺 :)
ai giúp em bài này với ạ 🥺 :)



