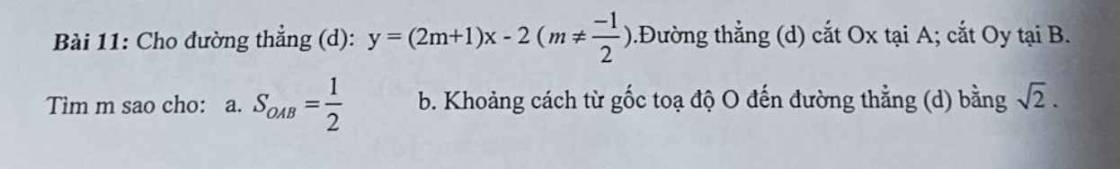a: Tọa độ A là:
\(\left\{{}\begin{matrix}y=0\\\left(2m+1\right)x-2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\x=\dfrac{2}{2m+1}\end{matrix}\right.\)
\(OA=\sqrt{\left(\dfrac{2}{2m+1}-0\right)^2+\left(0-0\right)^2}=\sqrt{\left(\dfrac{2}{2m+1}\right)^2}=\dfrac{2}{\left|2m+1\right|}\)
Tọa độ B là:
\(\left\{{}\begin{matrix}x=0\\y=\left(2m+1\right)\cdot x-2=-2\end{matrix}\right.\)
=>B(0;-2)
\(OB=\sqrt{\left(0-0\right)^2+\left(-2-0\right)^2}=2\)
\(S_{OAB}=\dfrac{1}{2}\)
=>\(\dfrac{1}{2}\cdot OA\cdot OB=\dfrac{1}{2}\)
=>\(OA\cdot OB=1\)
=>\(2\cdot\dfrac{2}{\left|2m+1\right|}=1\)
=>\(\dfrac{4}{\left|2m+1\right|}=1\)
=>|2m+1|=4
=>\(\left[{}\begin{matrix}2m+1=4\\2m+1=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}m=\dfrac{3}{2}\\m=-\dfrac{5}{2}\end{matrix}\right.\)
b: (d): y=(2m+1)x-2
=>(2m+1)x-y-2=0
Khoảng cách từ O đến (d) là:
\(d\left(O;\left(d\right)\right)=\dfrac{\left|0\left(2m+1\right)+0\left(-1\right)-2\right|}{\sqrt{\left(2m+1\right)^2+\left(-1\right)^2}}=\dfrac{2}{\sqrt{\left(2m+1\right)^2+1}}\)
Để \(d\left(O;\left(d\right)\right)=\sqrt{2}\) thì \(\dfrac{2}{\sqrt{\left(2m+1\right)^2+1}}=\sqrt{2}\)
=>\(\sqrt{\left(2m+1\right)^2+1}=\sqrt{2}\)
=>\(\left(2m+1\right)^2+1=2\)
=>\(\left(2m+1\right)^2=1\)
=>\(\left[{}\begin{matrix}2m+1=1\\2m+1=-1\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}m=0\\m=-1\end{matrix}\right.\)