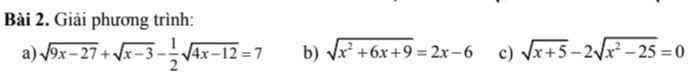a: ĐKXĐ: x>=3
\(PT\Leftrightarrow3\sqrt{x-3}+\sqrt{x-3}-\dfrac{1}{2}\cdot2\sqrt{x-3}=7\)
=>\(3\sqrt{x-3}=7\)
=>\(\sqrt{x-3}=\dfrac{7}{3}\)
=>\(x-3=\dfrac{49}{9}\)
=>\(x=\dfrac{49}{9}+3=\dfrac{76}{9}\)(nhận)
b: \(PT\Leftrightarrow\left|x+3\right|=2x-6\)
=>\(\left\{{}\begin{matrix}2x-6>=0\\\left(x+3\right)^2=\left(2x-6\right)^2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>=3\\\left(2x-6-x-3\right)\left(2x-6+x+3\right)=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=3\\\left(x-9\right)\left(3x-3\right)=0\end{matrix}\right.\Leftrightarrow x=9\)
c: ĐKXĐ: \(\left\{{}\begin{matrix}x+5>=0\\x^2-25>=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-5\\x>=5\end{matrix}\right.\)
\(PT\Leftrightarrow\sqrt{x+5}\left(1-2\sqrt{x-5}\right)=0\)
=>\(\left[{}\begin{matrix}x+5=0\\x-5=\dfrac{1}{4}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-5\left(nhận\right)\\x=\dfrac{21}{4}\left(nhận\right)\end{matrix}\right.\)