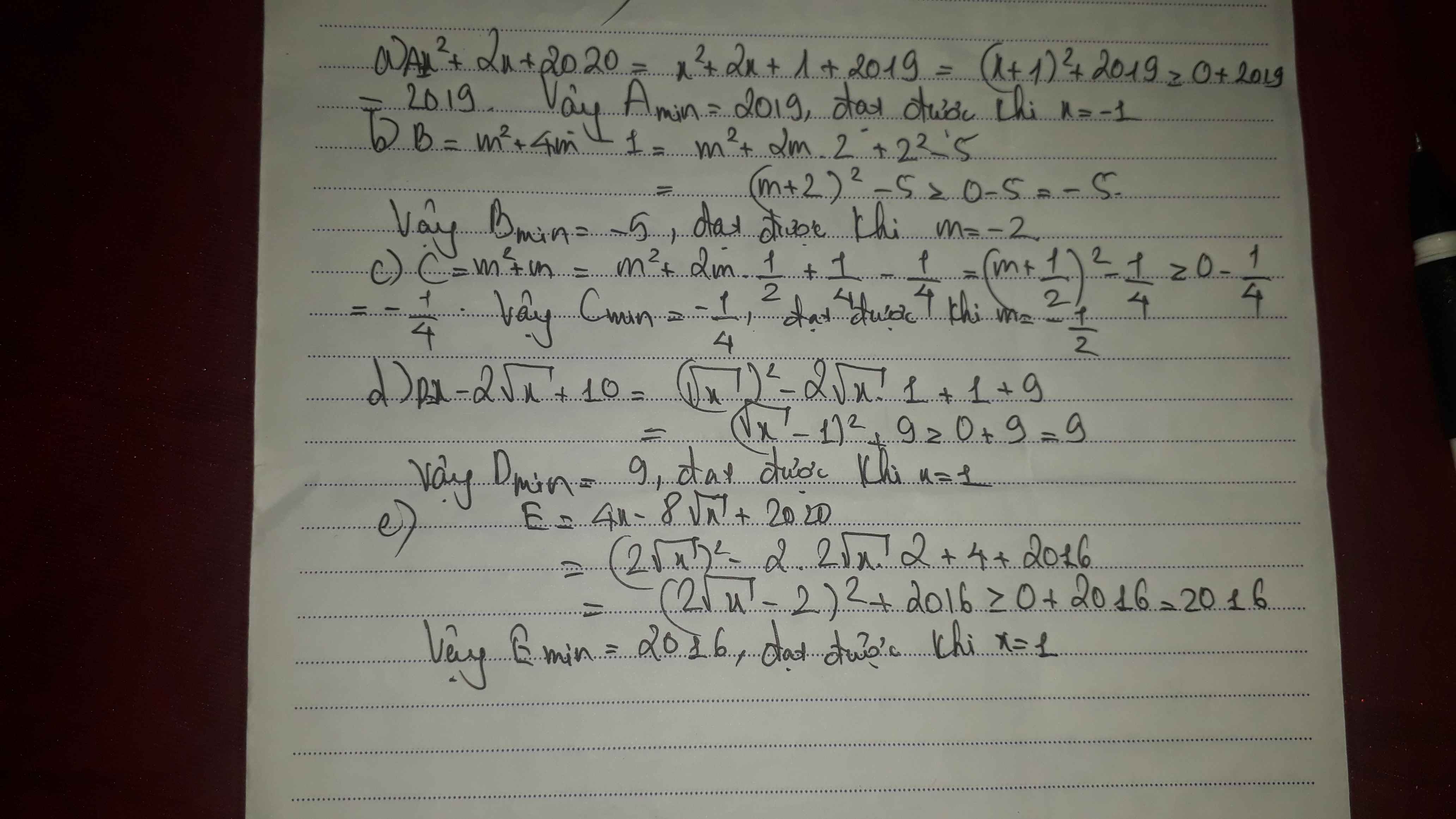\(a,=x^2+2x+1+2019=\left(x+1\right)^2+2019\ge2019\) dấu"=" xảy ra<=>x=-1
b,\(=m^2+2.2m+4-5=\left(m+2\right)^2-5\ge-5\) dấu"=" xảy ra<=>m=-2
c, \(=x-2\sqrt{x}+10=x-2\sqrt{x}+1+9=\left(\sqrt{x}-1\right)^2+9\ge9\)
dấu"=" xảy ra<=>x=1
b, \(4x-8\sqrt{x}+2020=4x-2.2.2\sqrt{x}+4+2016=\left(2\sqrt{x}-2\right)^2+2016\ge2016\)
dấu"=" xảy ra<=>x=1
a) Ta có: \(x^2+2x+2020\)
\(=x^2+2x+1+2019\)
\(=\left(x+1\right)^2+2019\ge2019\forall x\)
Dấu '=' xảy ra khi x=-1
b) Ta có: \(m^2+4m-1\)
\(=m^2+4m+4-5\)
\(=\left(m+2\right)^2-5\ge-5\forall m\)
Dấu '=' xảy ra khi m=-2
c) Ta có: \(m^2+m\)
\(=m^2+2\cdot m\cdot\dfrac{1}{2}+\dfrac{1}{4}-\dfrac{1}{4}\)
\(=\left(m+\dfrac{1}{2}\right)^2-\dfrac{1}{4}\ge-\dfrac{1}{4}\forall m\)
Dấu '=' xảy ra khi \(m=-\dfrac{1}{2}\)