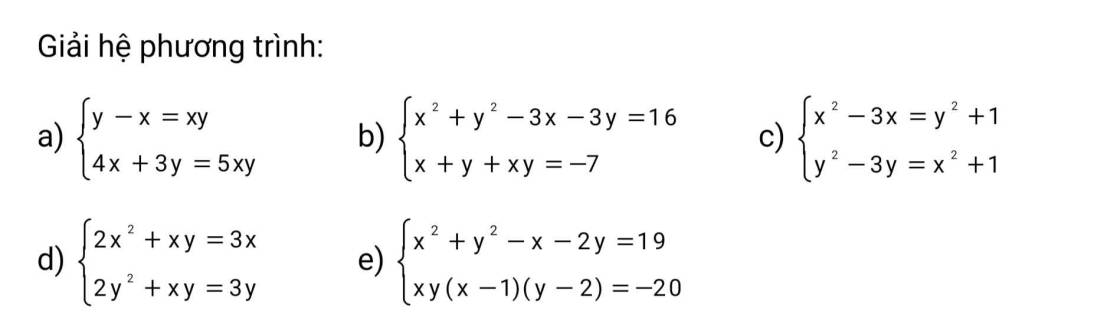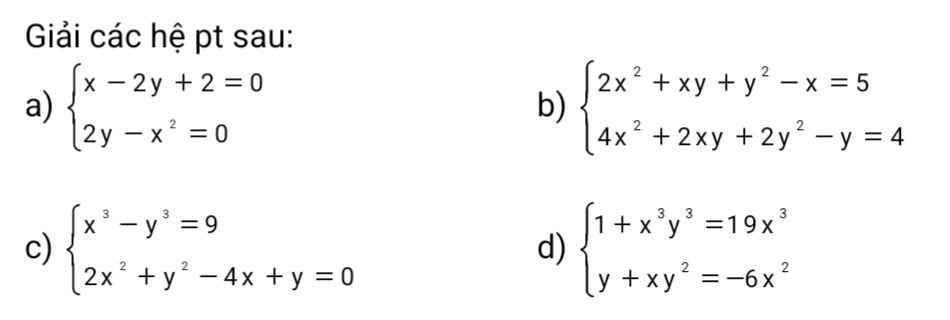a.
Pt có nghiệm khi:
\(\Delta'=\left(m+1\right)^2-\left(2m+3\right)\ge0\)
\(\Leftrightarrow m^2\ge2\)
\(\Rightarrow\left[{}\begin{matrix}m\ge\sqrt{2}\\m\le-\sqrt{2}\end{matrix}\right.\)
b.
\(\left\{{}\begin{matrix}x-my=0\\mx-y=m+1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x-my=0\\m^2x-my=m^2+m\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(m^2-1\right)x=m^2+m\\y=mx-m-1\end{matrix}\right.\)
Hệ có nghiệm duy nhất khi \(m^2-1\ne0\Rightarrow m\ne\pm1\)
Khi đó ta được:
\(\left\{{}\begin{matrix}x=\dfrac{m^2+m}{m^2-1}=\dfrac{m\left(m+1\right)}{\left(m-1\right)\left(m+\right)}=\dfrac{m}{m-1}\\y=m.\dfrac{m}{m-1}-m-1=\dfrac{1}{m-1}\end{matrix}\right.\)
\(y\) nguyên \(\Rightarrow\dfrac{1}{m-1}\) nguyên
\(\Rightarrow m-1=Ư\left(1\right)=\left\{-1;1\right\}\)
\(\Rightarrow\left[{}\begin{matrix}m=0\\m=2\end{matrix}\right.\)
Thay vào x thấy thỏa mãn x nguyên, vậy \(m=\left\{0;2\right\}\)