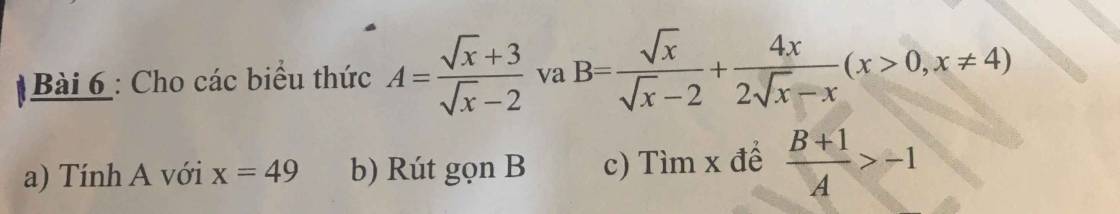\(a,x=49\Rightarrow A=\dfrac{\sqrt{49}+3}{\sqrt{49}-2}=\dfrac{7+3}{7-2}=\dfrac{10}{5}=2\)
\(b,B=\dfrac{\sqrt{x}}{\sqrt{x}-2}+\dfrac{4x}{2\sqrt{x}-x}\left(dk:x>0,x\ne4\right)\)
\(=\dfrac{\sqrt{x}}{\sqrt{x}-2}+\dfrac{4\sqrt{x^2}}{\sqrt{x}\left(2-\sqrt{x}\right)}\)
\(=\dfrac{\sqrt{x}}{\sqrt{x}-2}-\dfrac{4\sqrt{x}}{\sqrt{x}-2}\)
\(=\dfrac{\sqrt{x}-4\sqrt{x}}{\sqrt{x}-2}\)
\(=\dfrac{-3\sqrt{x}}{\sqrt{x}-2}\)
\(c,\dfrac{B+1}{A}>-1\Leftrightarrow\left(\dfrac{-3\sqrt{x}}{\sqrt{x}-2}+1\right):\dfrac{\sqrt{x}+3}{\sqrt{x}-2}>-1\)
\(\Leftrightarrow\dfrac{-3\sqrt{x}+\sqrt{x}-2}{\sqrt{x}-2}.\dfrac{\sqrt{x}-2}{\sqrt{x}+3}>-1\)
\(\Leftrightarrow\dfrac{-2\sqrt{x}-2}{\sqrt{x}+3}+1>0\)
\(\Leftrightarrow\dfrac{-2\sqrt{x}-2+\sqrt{x}+3}{\sqrt{x}+3}>0\)
\(\Leftrightarrow-\sqrt{x}+1>0\\ \Leftrightarrow-\sqrt{x}>-1\\ \Leftrightarrow x< 1\)
So với \(dk:x>0\)
Vậy \(S=\left\{x|0< x< 1\right\}\)