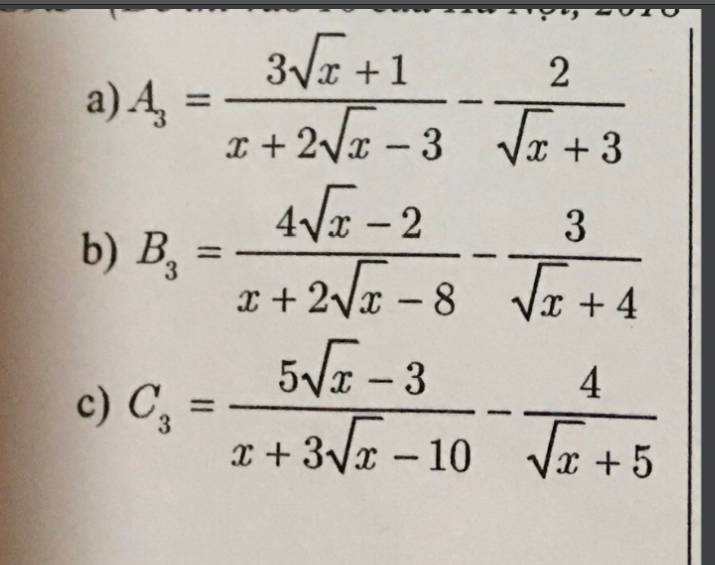a: \(A=\dfrac{3\sqrt{x}+1-2\sqrt{x}+2}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{\sqrt{x}+3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}=\dfrac{1}{\sqrt{x}-1}\)
b: \(=\dfrac{4\sqrt{x}-2-3\sqrt{x}+6}{\left(\sqrt{x}+4\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{\sqrt{x}+4}{\left(\sqrt{x}+4\right)\left(\sqrt{x}-2\right)}=\dfrac{1}{\sqrt{x}-2}\)
c) Điều kiện xác định: \(\left\{{}\begin{matrix}x\ge0\\x+3\sqrt{x}-10\ne\\\sqrt{x}+5\ne0\end{matrix}\right.0\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\x\ne4\end{matrix}\right.\)
\(C_3=\dfrac{5\sqrt{x}-3}{x+3\sqrt{x}-10}-\dfrac{4}{\sqrt{x}+5}\)
\(=\dfrac{5\sqrt{x}-3}{\left(x+5\sqrt{x}\right)-\left(2\sqrt{x}+10\right)}-\dfrac{4}{\sqrt{x}+5}\)
\(=\dfrac{5\sqrt{x}-3}{\sqrt{x}\left(\sqrt{x}+5\right)-2\left(\sqrt{x}+5\right)}-\dfrac{4}{\sqrt{x}+5}\)
\(=\dfrac{5\sqrt{x}-3}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+5\right)}-\dfrac{4\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+5\right)}\)
\(=\dfrac{5\sqrt{x}-3-4\sqrt{x}+8}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+5\right)}\)
\(=\dfrac{\sqrt{x}+5}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+5\right)}\)
\(=\dfrac{1}{\sqrt{x}-2}\)