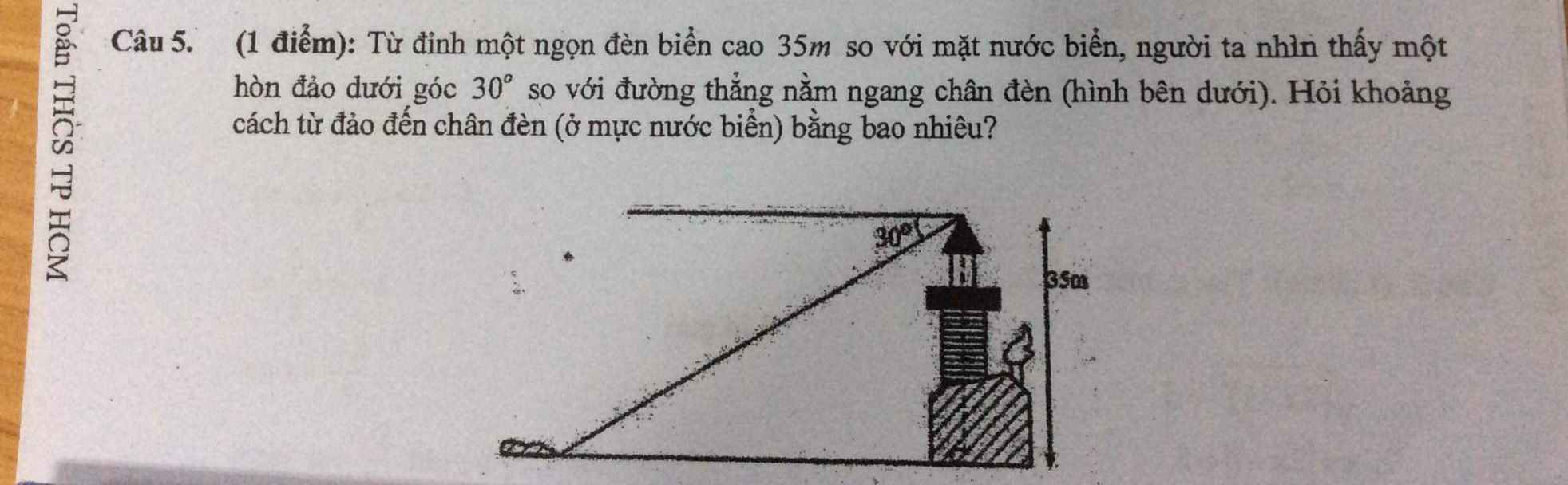
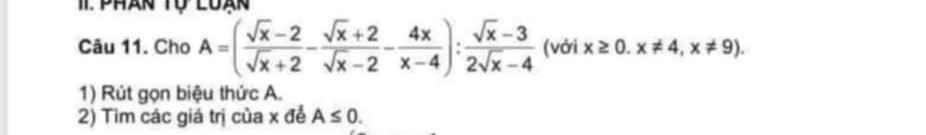1: \(A=\left(\dfrac{\sqrt{x}-2}{\sqrt{x}+2}-\dfrac{\sqrt{x}+2}{\sqrt{x}-2}-\dfrac{4x}{x-4}\right):\dfrac{\sqrt{x}-3}{2\sqrt{x}-4}\)
\(=\left(\dfrac{\sqrt{x}-2}{\sqrt{x}+2}-\dfrac{\sqrt{x}+2}{\sqrt{x}-2}-\dfrac{4x}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\right)\cdot\dfrac{2\left(\sqrt{x}-2\right)}{\sqrt{x}-3}\)
\(=\dfrac{\left(\sqrt{x}-2\right)^2-\left(\sqrt{x}+2\right)^2-4x}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\cdot\dfrac{2\left(\sqrt{x}-2\right)}{\sqrt{x}-3}\)
\(=\dfrac{x-4\sqrt{x}+4-x-4\sqrt{x}-4-4x}{\left(\sqrt{x}+2\right)}\cdot\dfrac{2}{\sqrt{x}-3}\)
\(=\dfrac{-4x-8\sqrt{x}}{\left(\sqrt{x}+2\right)}\cdot\dfrac{2}{\sqrt{x}-3}=\dfrac{-4\sqrt{x}\cdot2}{\sqrt{x}-3}=-\dfrac{8\sqrt{x}}{\sqrt{x}-3}\)
2: Để A<=0 thì \(-\dfrac{8\sqrt{x}}{\sqrt{x}-3}< =0\)
=>\(\dfrac{\sqrt{x}}{\sqrt{x}-3}>=0\)
mà \(\sqrt{x}>=0\forall x\) thỏa mãn ĐKXĐ
nên \(\sqrt{x}-3>0\)
=>\(\sqrt{x}>3\)
=>x>9