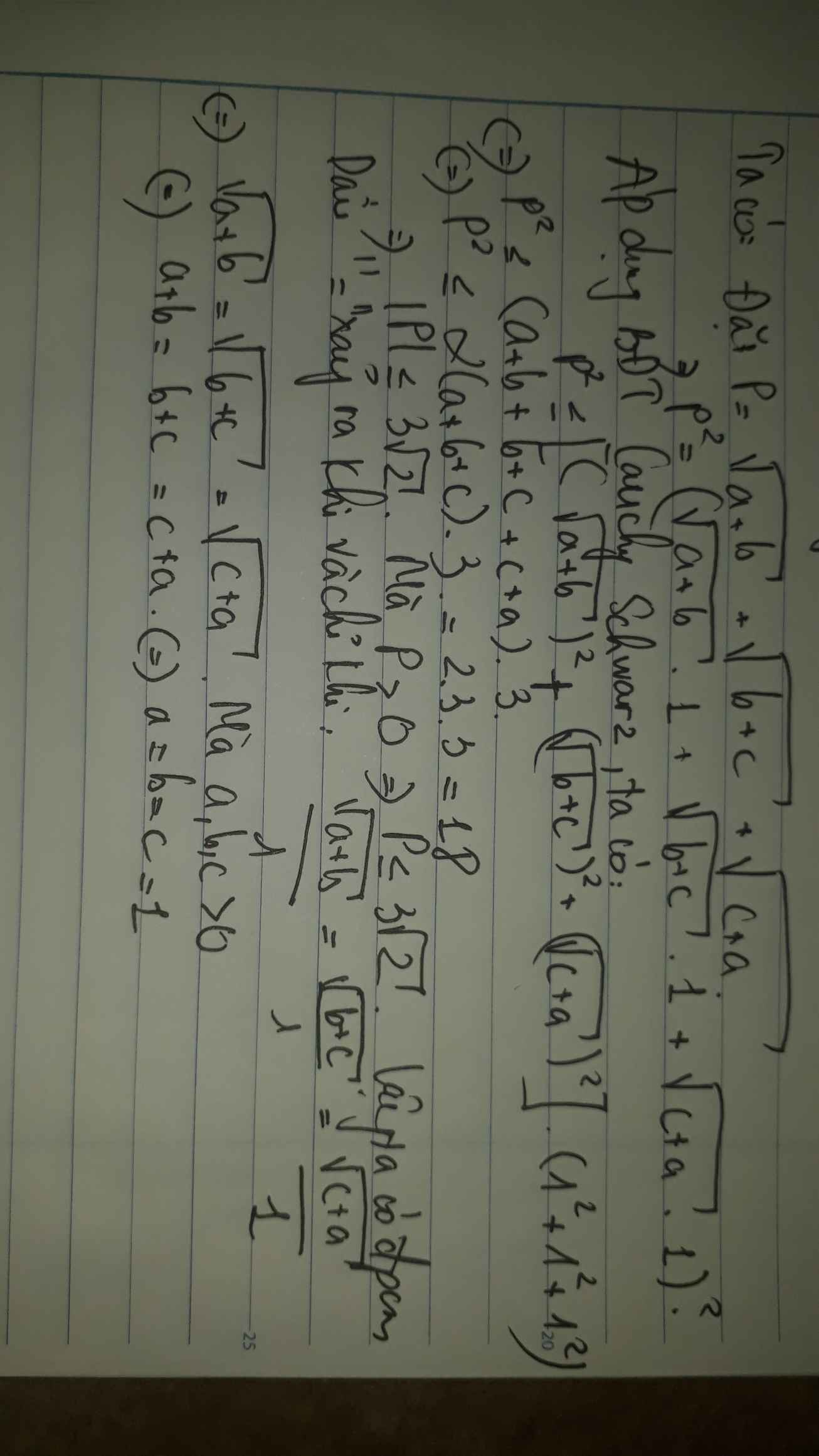Các câu hỏi tương tự
Cho a,b,c>0 thỏa mãn a+b+c=3
\(\sqrt{2a+b}+\sqrt{2b+c}+\sqrt{2c+a}\le3\sqrt{3}\)
\(\sqrt[3]{a+b+c}+\sqrt[3]{b+c+1}+\sqrt[3]{c+a+1}\le3\sqrt[3]{3}\)
Các thiên tài toán học ơi giải hộ mình bài này với ạ!
Cảm ơn !
Bài 1 (1 đ) : Cho ba số không âm \(a,b,c\) thỏa mãn \(a+c\ge b\) và \(\sqrt{a}-\sqrt{b}+\sqrt{c}\text{=}\sqrt{a-b+c}\) . Tính giá trị của biểu thức : \(A\text{=}a^{2021}-b^{2021}+c^{2021}-\left(a+b+c\right)^{2021}\)
Cho a,b,c>0 thỏa mãn \(a^2+b^2+c^2\le3\). Chứng minh rằng: \(\sqrt{5a^2+4bc}+2\sqrt{bc}\le5\)
GIÚP EM BÀI NÀY GẤP ĐƯỢC KHÔNG Ạ!!! HIHI
Cho a,b,c>0
CMR
a/((b+c)*sqrt(a^2+2bc))+b/((a+c)*sqrt(b^2+2ac)) + c/((b+c)*sqrt(c^2+2ab) >= 3/(2*sqrt(a^2+b^2+c^2)). ^_^
1) Cho a, b, c>0 và a+b+c=3. Chứng minh rằng: \(\frac{a}{b^3+ab}+\frac{b}{c^3+bc}+\frac{c}{a^3+ac}\ge\frac{3}{2}\)
2) Cho a, b, c >0 thỏa mãn: ab+ac+bc+abc=4. Chứng minh rằng: \(\sqrt{ab}+\sqrt{ac}+\sqrt{bc}\le3\)
\(\sqrt[3]{a+2b}+\sqrt[3]{b+2c}+\sqrt[3]{c+2a}\le3\sqrt[3]{3}\)Cho a ,b,c > 0 thoả mãn a+b+c =3 CMR :
Dù iêm có sol rồi nhưng vẫn muốn xin tiếp hjhjhj (tham quá)
Cho a,b,c>0 thỏa mãn: \(a^4+b^4+c^4\le3\). CMR:
\(\frac{\sqrt{a^2+b^2}+\sqrt{b^2+c^2}+\sqrt{c^2+a^2}}{\sqrt[3]{\frac{1}{\left(a+b\right)^3}+\frac{1}{\left(b+c\right)^3}+\frac{1}{\left(c+a\right)^3}}}\le\frac{2.\sqrt{2}.\sqrt[3]{27}}{\sqrt[3]{3}}\)
Cho a,b,c là các số thực dương thỏa mãn \(a^2+b^2+c^2=3\)
CMR: \(\frac{a^2+3ab+b^2}{\sqrt{6a^2+8ab+11b^2}}+\frac{b^2+3bc+c^2}{\sqrt{6b^2+8bc+11c^2}}+\frac{c^2+3ca+a^2}{\sqrt{6c^2+8ca+11a^2}}\le3\)
Các bạn giải hộ tớ bài này nhé! Cảm ơn rất nhiều!
1) cho 25 số tự nhiên a1;a2;a3;....;a25 thỏa frac{1}{sqrt{a1}}+frac{1}{sqrt{a2}}+...+frac{1}{sqrt{a25}}9.CM trong 25 số đó có 2 số bằng nhau 2) cho a,b,c là độ dài 3 cạnh tam giác.CMR sqrt{2}left(a+b+cright)lesqrt{a^2+b^2}+sqrt{b^2+c^2}+sqrt{c^2+a^2}le3left(a+b+cright)3) cho a,b,c 0.CMR sqrt{frac{a}{b+c}}+sqrt{frac{b}{a+c}}+sqrt{frac{c}{a+b}}2
Đọc tiếp
1) cho 25 số tự nhiên a1;a2;a3;....;a25 thỏa
\(\frac{1}{\sqrt{a1}}+\frac{1}{\sqrt{a2}}+...+\frac{1}{\sqrt{a25}}=9\).CM trong 25 số đó có 2 số bằng nhau
2) cho a,b,c là độ dài 3 cạnh tam giác.CMR \(\sqrt{2}\left(a+b+c\right)\le\sqrt{a^2+b^2}+\sqrt{b^2+c^2}+\sqrt{c^2+a^2}\le3\left(a+b+c\right)\)
3) cho a,b,c >0.CMR \(\sqrt{\frac{a}{b+c}}+\sqrt{\frac{b}{a+c}}+\sqrt{\frac{c}{a+b}}>2\)