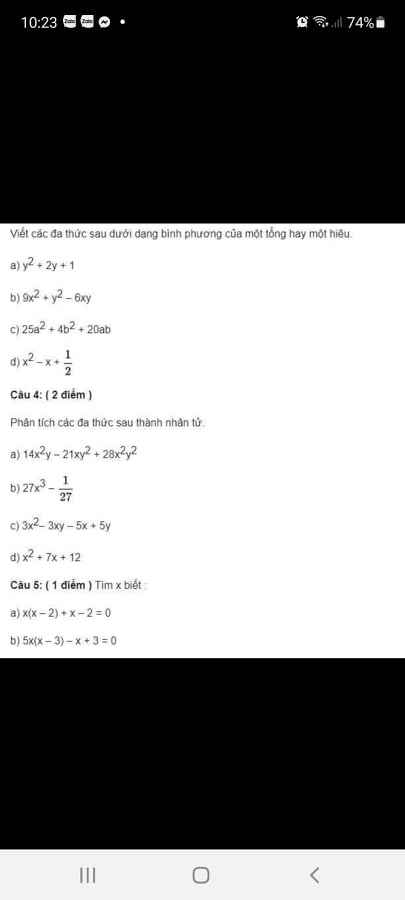Question

giai chi tiet giup mik

 

Answer 1

Câu 3:

a. $y^2+2y+1=(y+1)^2$
b. $9x^2+y^2-6xy=(3x)^2-2.3x.y+y^2=(3x-y)^2$
c. $25a^2+4b^2+20ab=(5a)^2+2.5a.2b+(2b)^2$

$=(5a+2b)^2$
d. Sửa đề:

$x^2-x+\frac{1}{4}=x^2-2.x.\frac{1}{2}+(\frac{1}{2})^2$

$=(x-\frac{1}{2})^2$

 

Answer 2

Câu 5:

a. $x(x-2)+x-2=0$

$\Leftrightarrow x(x-2)+(x-2)=0$

$\Leftrightarrow (x-2)(x+1)=0$

$\Leftrightarrow x-2=0$ hoặc $x+1=0$

$\Leftrightarrow x=2$ hoặc $x=-1$

b. 

$5x(x-3)-x+3=0$

$\Leftrightarrow 5x(x-3)-(x-3)=0$
$\Leftrightarrow (x-3)(5x-1)=0$

$\Leftrightarrow x-3=0$ hoặc $5x-1=0$

$\Leftrightarrow x=3$ hoặc $x=\frac{1}{5}$

Answer 3

Câu 4:

a. $14x^2y-21xy^2+28x^2y^2$

$=7xy(2x-3y+4xy)$

b. $27x^3-\frac{1}{27}=(3x)^3-(\frac{1}{3})^3$

$=(3x-\frac{1}{3})(9x^2+x+\frac{1}{9})$

c. $3x^2-3xy-5x+5y$

$=3x(x-y)-5(x-y)=(x-y)(3x-5)$

d.

$x^2+7x+12=(x^2+3x)+(4x+12)$
$=x(x+3)+4(x+3)=(x+4)(x+3)$

 

Answer 4

Câu 5: 

a: Ta có: \(x\left(x-2\right)+x-2=0\)

\(\Leftrightarrow\left(x-2\right)\left(x+1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-1\end{matrix}\right.\)

b: Ta có: \(5x\left(x-3\right)-x+3=0\)

\(\Leftrightarrow\left(x-3\right)\left(5x-1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=\dfrac{1}{5}\end{matrix}\right.\)