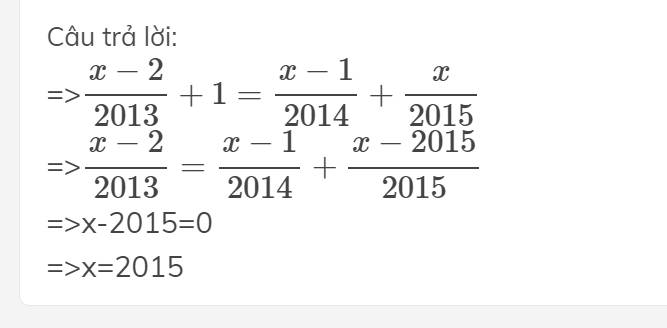Các câu hỏi tương tự
Nghiệm nhỏ nhất của pt\(\frac{1}{2\sqrt{x}-2014}+\frac{1}{3\sqrt{x}+2013}=\frac{1}{2015-4\sqrt{x}}+\frac{1}{9\sqrt{x}-2016}\)
Tìm nghiệm nhỏ nhất của phương trình \(\frac{1}{2\sqrt{x}-2014}+\frac{1}{3\sqrt{x}+2013}=\frac{1}{2015-4\sqrt{x}}+\frac{1}{9\sqrt{x}-2016}\)
Nghiệm nhỏ nhất của phương trình: \(\frac{1}{2\sqrt{x}-2014}+\frac{1}{3\sqrt{x}+2013}=\frac{1}{2015-4\sqrt{x}}+\frac{1}{9\sqrt{x}-2016}\)
là...?
Tính gía trị biểu thức:
\(A=\frac{1}{2\sqrt{1}+1\sqrt{2}}+\frac{1}{3\sqrt{2}+2\sqrt{3}}+....+\frac{1}{2014\sqrt{2013}+2013\sqrt{2014}}+\frac{1}{2015\sqrt{2014}+2014\sqrt{2015}}\)
Giải phương trình: \(\frac{x+4037}{2016}+\frac{x+6050}{2015}+\frac{x+8061}{2014}=\frac{x+18122}{2013}\)
1 giải phương trình
\(\frac{x+2011}{2013}+\frac{x+2012}{2012}=\frac{x+2010}{2014}+\frac{x+2013}{2011}\)
2 . \(\frac{x^2+2x+1}{x^2+2x+2}+\frac{x^2+2x+2}{x^2+2x+3}=\frac{7}{6}\)
Giải pt:
\(\frac{\sqrt{x-2014}-1}{x-2014}+\frac{\sqrt{y-2015}-1}{y-2015}+\frac{\sqrt{z-2016}-1}{z-2016}=\frac{3}{4}\)
Cho x,y,z khác 0 và thỏa mãn:
\(x+y+z=\frac{1}{2}\)
\(\frac{1}{x^2}+\frac{1}{y^2}+\frac{1}{z^2}+\frac{1}{xyz}=4\)
\(\frac{1}{x}+\frac{1}{y}+\frac{1}{z}>0\)
Tính giá trị của biểu thức:\(P=\left(x^{2011}+y^{2011}\right)\left(y^{2013}+z^{2013}\right)\left(z^{2015}+x^{2015}\right)\)
Giải giúp
Cho x,y,z thỏa mãn \(\frac{1}{x}+\frac{1}{y}+\frac{1}{z}=\frac{1}{x+y+z}\), tính giá trị biểu thức: \(M=\frac{19}{4}+\left(x^{2013}+y^{2013}\right)\left(y^{2015}+z^{2015}\right)\left(z^{2017}+x^{2017}\right)\)