Question

  ai help em voi ạ

Answer 1

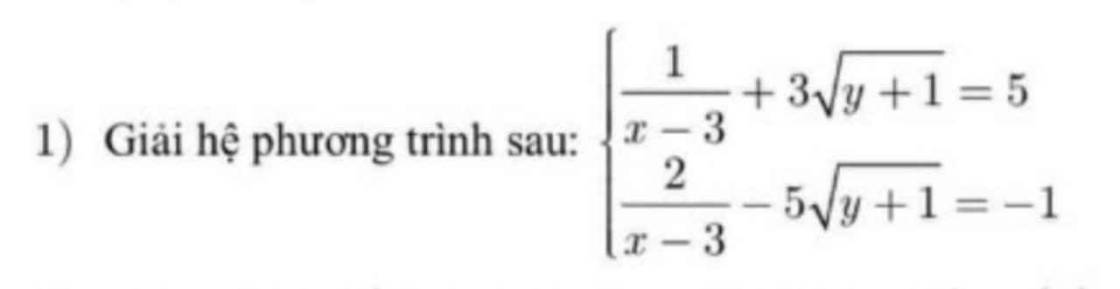
Lời giải:

ĐKXĐ: $x\neq 3; y\geq -1$

Đặt $\frac{1}{x-3}=a; \sqrt{y+1}=b(b\geq 0)$ thì hpt trở thành:

\(\left\{\begin{matrix} a+3b=5\\ 2a-5b=-1\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} 2a+6b=10\\ 2a-5b=-1\end{matrix}\right.\)

$\Rightarrow (2a+6b)-(2a-5b)=11$

$\Leftrightarrow 11b=11$

$\Leftrightarrow b=1$ (tm) 

$a=5-3b=5-3=2$ 

Khi đó: $(a,b)=(2,1)$

$\Leftrightarrow (\frac{1}{x-3}, \sqrt{y+1})=(2,1)$

$\Leftrightarrow (x,y)=(\frac{7}{2}, 0)$