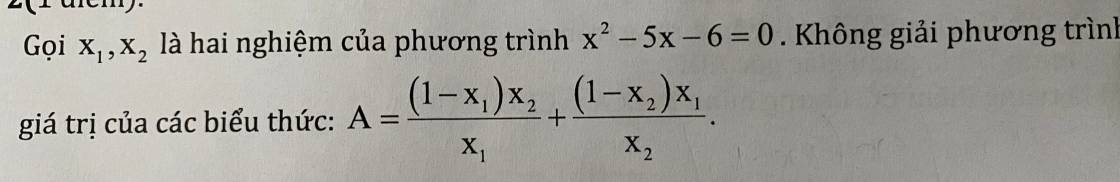Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=5\\x_1x_2=-6\end{matrix}\right.\)
\(A=\dfrac{x_2-x_1x_2}{x_1}+\dfrac{x_1-x_1x_2}{x_2}=\dfrac{x_2+6}{x_1}+\dfrac{x_1+6}{x_2}\)
\(=\dfrac{x_2\left(x_2+6\right)+x_1\left(x_1+6\right)}{x_1x_2}=\dfrac{x_1^2+x_2^2+6\left(x_1+x_2\right)}{x_1x_2}\)
\(=\dfrac{\left(x_1+x_2\right)^2-2x_1x_2+6\left(x_1+x_2\right)}{x_1x_2}=\dfrac{5^2-2.\left(-6\right)+6.5}{-6}=...\)
Đúng 2
Bình luận (0)
