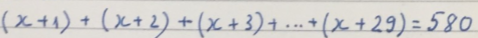Question

Answer 1

\(\text{(x + 1) + (x + 2) + (x + 3) + ... + (x + 29) = 580}\)

\(\Leftrightarrow29x + (1 + 2 + 3 + ... + 29) = 580\)

\(\Leftrightarrow29x+(1+29)\times29\div2=580\)

\(\Leftrightarrow29x+30\times29\div2=580\)

\(\Leftrightarrow29x+435=580\)

\(\Leftrightarrow29x=580-435\)

\(\Leftrightarrow29x=145\Leftrightarrow x=5\)

Answer 2

=>29x+435=580

=>29x=145

hay x=5

Answer 3

(x+1)+(x+2)+(x+3)+...+(x+29)=580
\(\Leftrightarrow\)29x+\(\dfrac{\left(29+1\right)29}{2}\)=580
\(\Rightarrow\)29x+435=580
\(\Rightarrow\)29x=145
\(\Rightarrow\)x=5

Answer 4

X=5