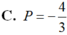Question

Answer 1

\(\left|\overrightarrow{BM}+\overrightarrow{BC}\right|=2\cdot BD=\dfrac{\sqrt{a^2+12a}}{4}\)

Answer 2

Do tam giác ABC đều \(\Rightarrow\left\{{}\begin{matrix}BM=\dfrac{a\sqrt{3}}{2}\\\widehat{CBM}=30^0\end{matrix}\right.\)

\(\left|\overrightarrow{BM}+\overrightarrow{BC}\right|^2=BM^2+BC^2+2\overrightarrow{BM}.\overrightarrow{BC}\)

\(=BM^2+BC^2+2BM.BC.cos\widehat{CBM}\)

\(=\left(\dfrac{a\sqrt{3}}{2}\right)^2+a^2+2.\dfrac{a\sqrt{3}}{2}.a.cos30^0=\dfrac{13a^2}{4}\)

\(\Rightarrow\left|\overrightarrow{BM}+\overrightarrow{BC}\right|=\dfrac{a\sqrt{13}}{2}\)