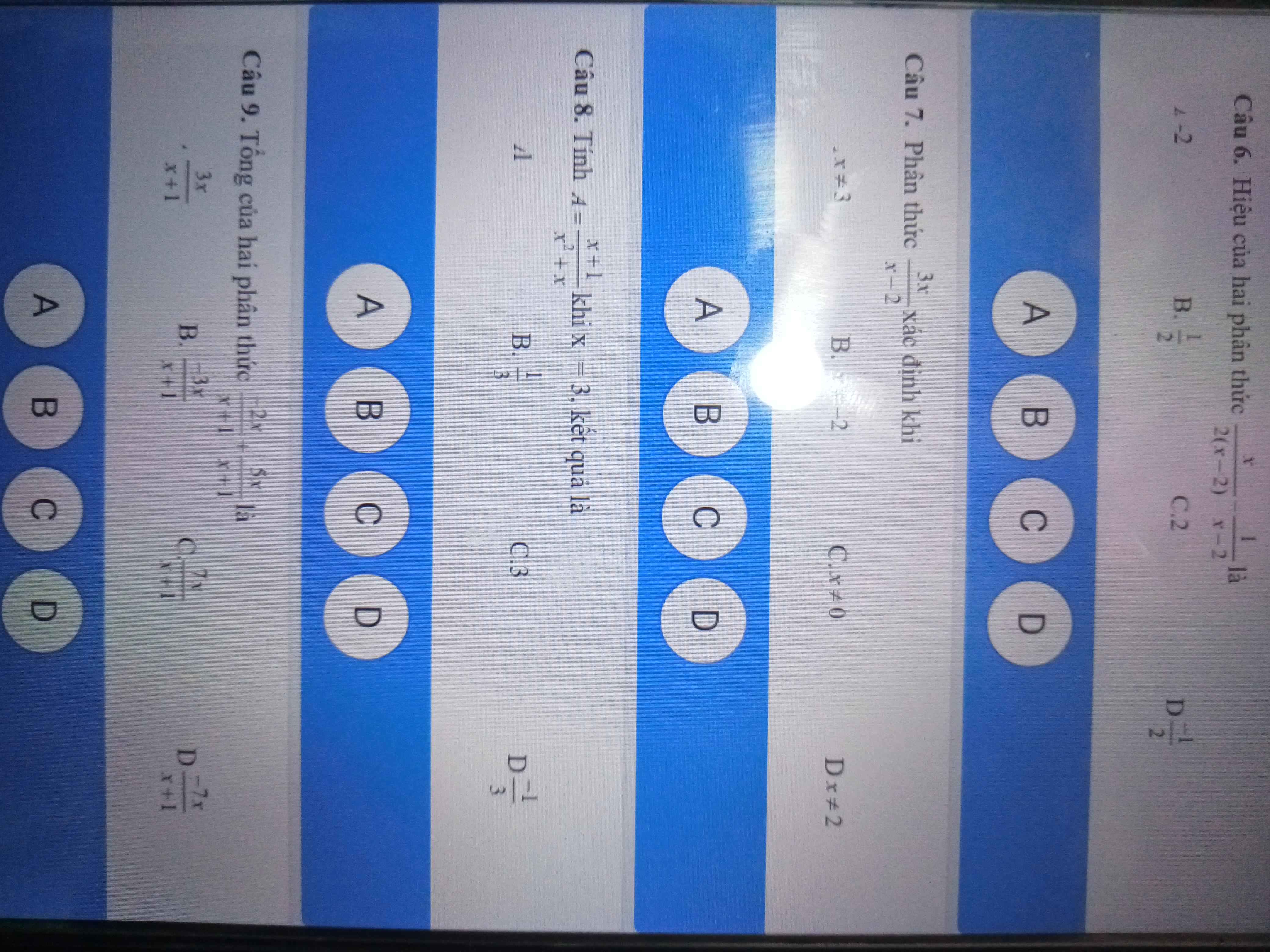
Tại x = 2022 suy ra : 2023 = x + 1
Khi đó ta có : \(P\left(2022\right)\) \(=x^{x+1}-\left(x+1\right)x^x+\left(x+1\right)x^{x-1}-\left(x+1\right)x^{x-2}+...+\left(x+1\right)x-\left(x+1\right)\)
\(=x^{x+1}-x^{x+1}-x^x+x^x+x^{x-1}-x^{x-1}-x^{x-2}+...+x^2+x-x-1\)
\(=-1\)
Vậy ...
Mng ơi, giúp mình bài này với ạ! Mình đang cần gấp, cảm ơn mng nhiều lắm ạ!!
Mng giúp em giải từng bước 4 câu này với ạ, em cảm ơn ạ
Mng giúp em với, em cảm ơn nhiều ạ
tìm giá trị lớn nhất của biểu thức A= 4x^2+9999/x^2+98999998
mk làm ko ra vì đề bài sai ạ mng giúp mk với cảm ơn nhiều ạ :)))))
Giúp mk mấy câu này với cảm ơn nhiều !
Tìm x
a) 3*(x-3)+4x-4=1
b) 6x*(3x+5)-2x*(9x-2)+(17-x)*(x-1)+x*(x-18)=0
Mng giúp mk bài này nka ạ, cảm ơn nhiều
Giúp mk mấy câu hỏi này với cảm ơn nhiều
Giải giúp mình hai câu này với ạ cảm ơn mn nhiều
Anh chị giúp e giải câu này với ạ! Em cảm ơn rất nhiều ạ<3
6x^2-73x+123=0
Mng ơi, giúp mình với ạ. Cảm ơn nhiều nhaa=''))
