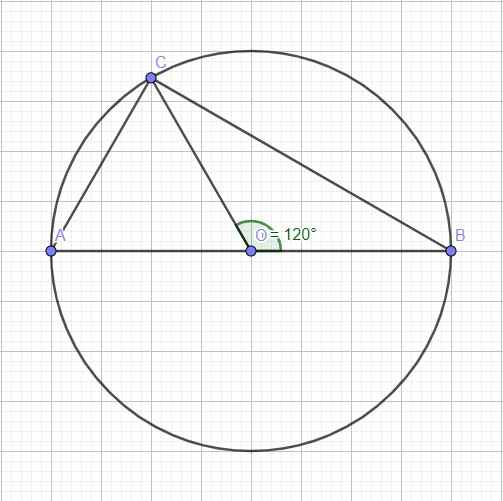
Do AB là đường kính \(\Rightarrow\widehat{ACB}\) là góc nội tiếp chắn nửa đường tròn
\(\Rightarrow\) Tam giác ABC vuông tại C
Mặt khác \(OA=OC=R\Rightarrow\Delta OAC\) cân tại O (1)
\(\widehat{AOC}=180^0-\widehat{BOC}=60^0\) (2)
(1);(2) \(\Rightarrow\Delta AOC\) đều \(\Rightarrow AC=OA=R\)
Áp dụng Pitago:
\(BC=\sqrt{AB^2-AC^2}=\sqrt{\left(2R\right)^2-R^2}=R\sqrt{3}\)