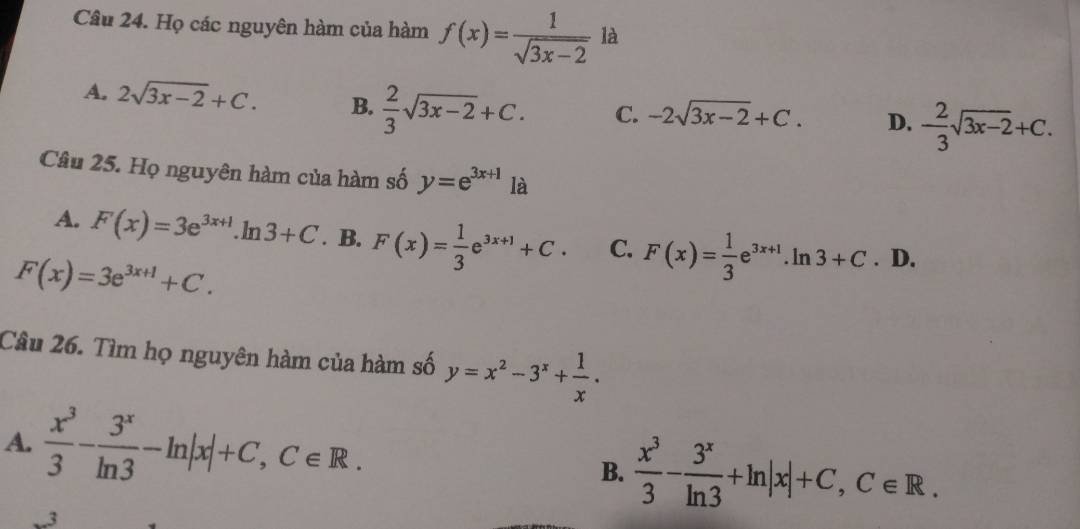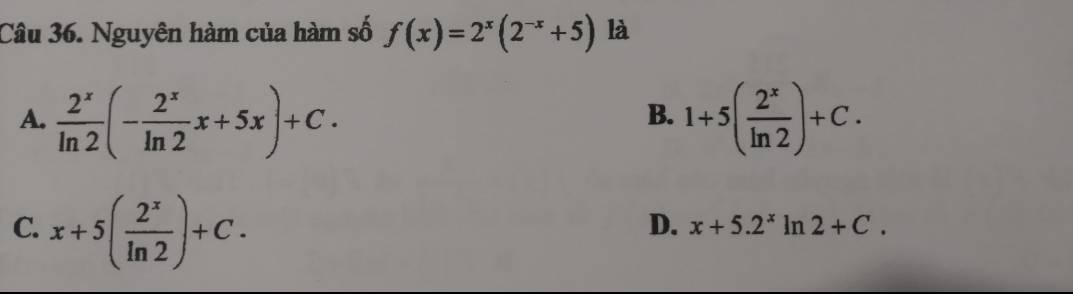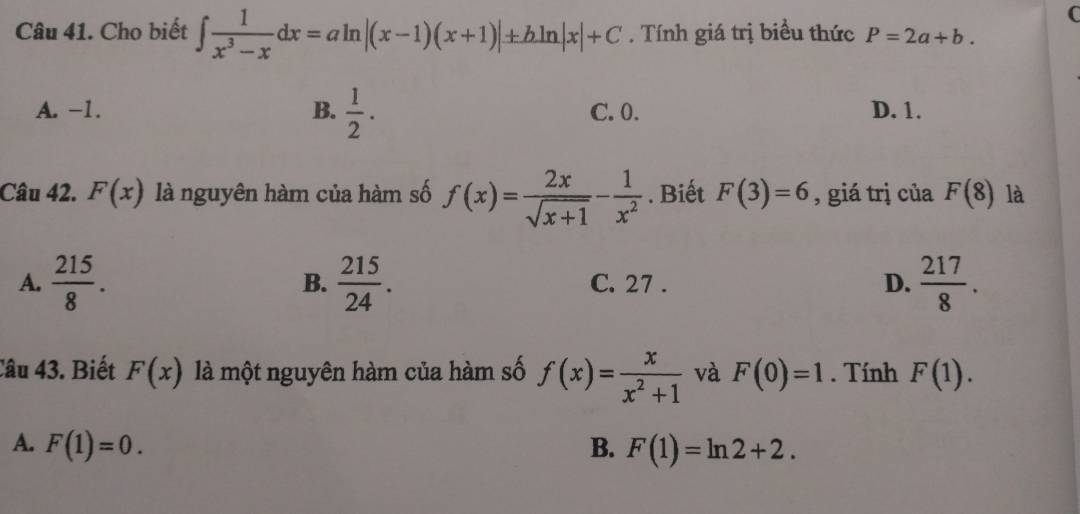17.
\(log_a\dfrac{a}{bc}=log_aa-log_a\left(bc\right)=log_aa-\left(log_ab+lo_ac\right)\)
\(=1-\left(2+3\right)=-4\)
18.
\(log_a\left(a^3b^2\sqrt{c}\right)=log_aa^3+log_ab^2+log_a\sqrt{c}\)
\(=3log_aa+2log_ab+\dfrac{1}{2}log_ac=3+2.3+\dfrac{1}{2}.\left(-1\right)=8\)
19.
\(log_{27}\left(\dfrac{\sqrt{x}}{y}\right)^3=3log_{3^3}\left(\dfrac{\sqrt{x}}{y}\right)=3.\dfrac{1}{3}log_3\left(\dfrac{\sqrt{x}}{y}\right)\)
\(=log_3\sqrt{x}-log_3y=\dfrac{1}{2}log_3x-log_3y=\dfrac{\alpha}{2}-\beta\)
20.
\(\log_{\sqrt{a}}(a^2\sqrt{b})=\log_{a^\frac{1}{2}}(a^2\sqrt{b})=2\log_a(a^2\sqrt{b})\)
\(=2\log_aa^2+2\log_a\sqrt{b}=4\log_aa+\log_ab=4+\log_ab\)
21.
\(y'=\dfrac{\left(2+2019^x\right)'}{\left(2+2019^x\right).\ln2019}=\dfrac{2019^x.\ln2019}{\left(2+2019^x\right).\ln2019}=\dfrac{2019^x}{2+2019^x}\)
22.
Do \(\dfrac{\sqrt{3}}{3}< \dfrac{\sqrt{2}}{2}\) mà \(a^{\frac{\sqrt{3}}{3}}>a^{\frac{\sqrt{2}}{2}}\) \(\Rightarrow0< a< 1\)
Do \(\dfrac{3}{4}< \dfrac{4}{5}\) mà \(\log_b\dfrac{3}{4}< \log_b\dfrac{4}{5}\Rightarrow b>1\)
Vậy \(0< a< 1,b>1\)
23.
Hàm đồng biến trên R khi:
\(a^2-3a+3>1\Leftrightarrow a^2-3a+2>0\Rightarrow\left[{}\begin{matrix}a< 1\\a>2\end{matrix}\right.\)