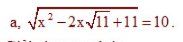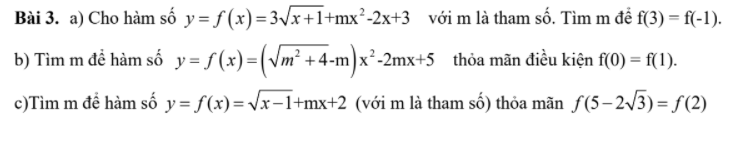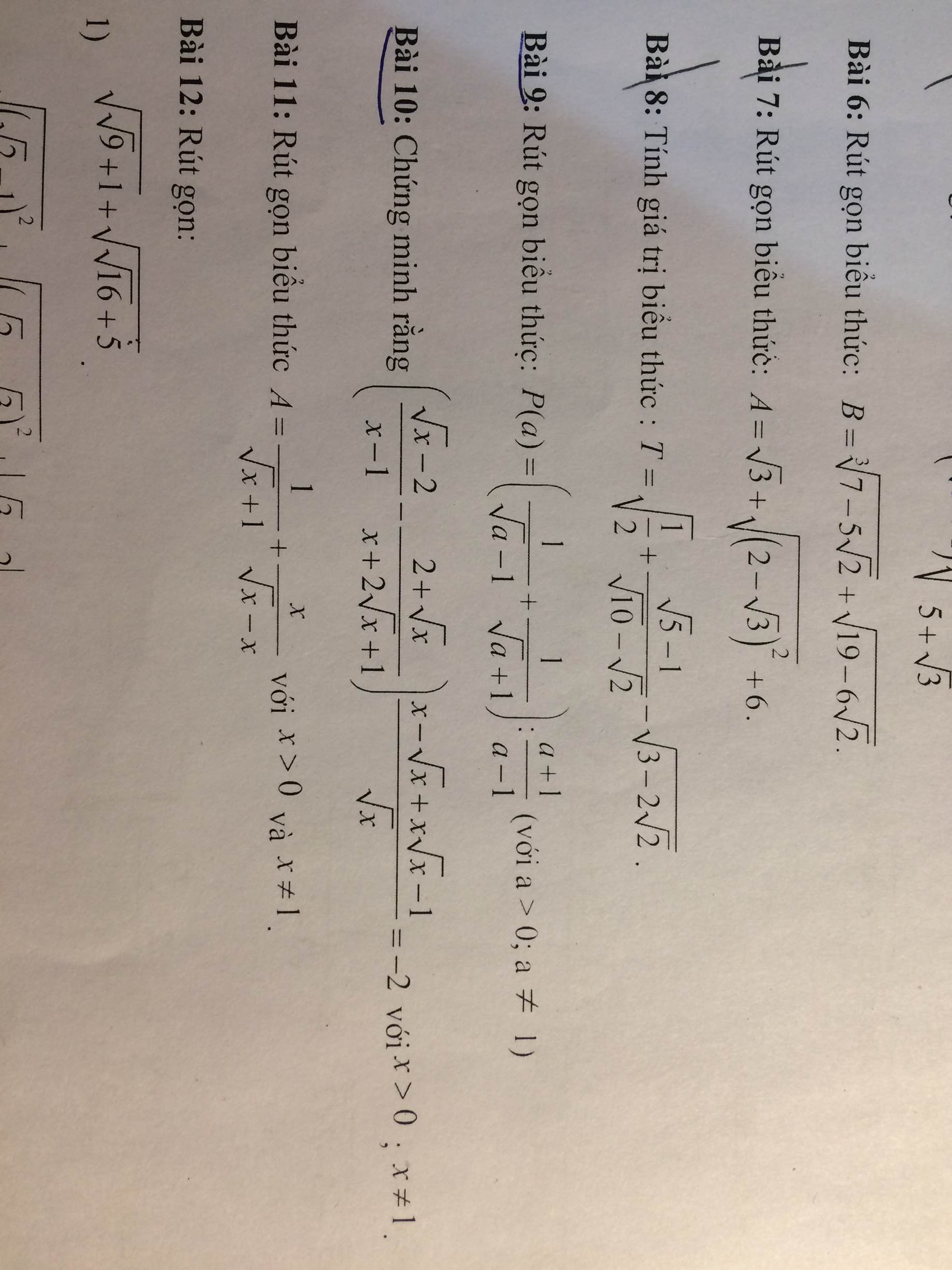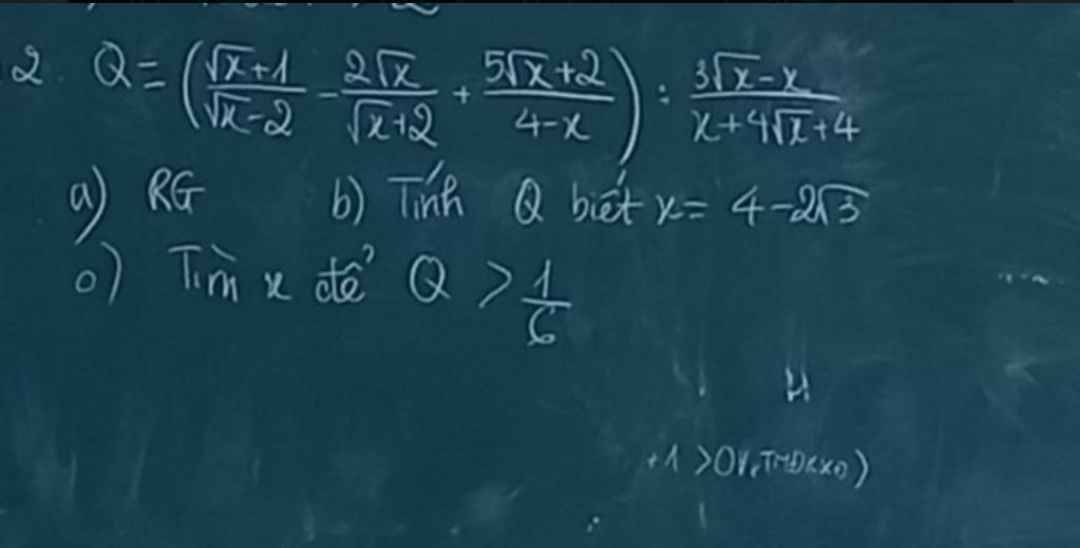1. ĐKXĐ: $x\geq 1$
PT $\Leftrightarrow \sqrt{x-1}=13-x$
\(\Rightarrow \left\{\begin{matrix} 13-x\geq 0\\ x-1=(13-x)^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\leq 13\\ x^2-27x+170=0\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\leq 13\\ (x-17)(x-10)=0\end{matrix}\right.\)
\(\Rightarrow x=10\) (tm)
2. ĐKXĐ: $x\geq 3$
\(3\sqrt{x+34}-3\sqrt{x-3}=1\)
\(\Leftrightarrow 3\sqrt{x+34}=3\sqrt{x-3}+1\)
\(\Rightarrow 9(x+34)=9x+6\sqrt{x-3}-26\)
\(\Leftrightarrow \frac{166}{3}=\sqrt{x-3}\)
$\Leftrightarrow x-3=\frac{27556}{9}$
$\Leftrightarrow x=\frac{27583}{9}$ (tm)
3.
ĐKXĐ: $x\ge \frac{5}{3}$
\(\sqrt{2x+5}-\sqrt{3x-5}=2\)
$\Leftrightarrow \sqrt{2x+5}=2+\sqrt{3x-5}$
$\Leftrightarrow 2x+5=3x+4\sqrt{3x-5}-1$
$\Leftrightarrow 6-x=4\sqrt{3x-5}$
\(\Rightarrow \left\{\begin{matrix}
6-x\geq 0\\
(6-x)^2=16(3x-5)\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix}
x\leq 6\\
x^2-60x+116=0\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\leq 6\\ (x-58)(x-2)=0\end{matrix}\right.\Rightarrow x=2\)
Thử lại thấy tm
4. ĐKXĐ: $7\leq x\leq 12$
\(\sqrt{x+1}-\sqrt{x-7}=\sqrt{12-x}\)
\(\Rightarrow 2x-6-2\sqrt{(x+1)(x-7)}=12-x\) (bình phương 2 vế)
\(\Leftrightarrow 3x-18=2\sqrt{(x+1)(x-7)}\)
\(\Rightarrow 9(x-6)^2=4(x+1)(x-7)\) (bình phương 2 vế)
\(\Leftrightarrow 5x^2-84x+352=0\)
\(\Leftrightarrow (5x-44)(x-8)=0\)
$\Leftrightarrow x=\frac{44}{5}$ hoặc $x=8$ (đều tm)