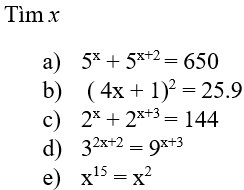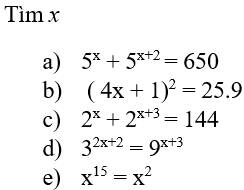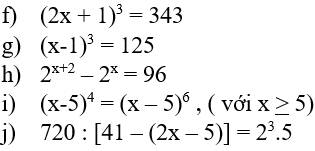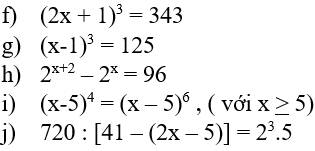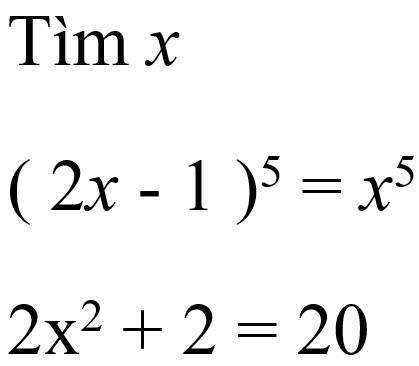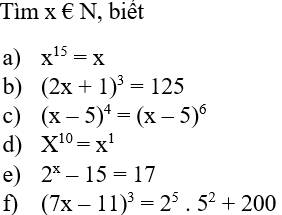`@` `\text {Ans}`
`\downarrow`
`a)`
\(5^x+5^{x+2}=650\)
`\Rightarrow 5^x + 5^x . 5^2 = 650`
`\Rightarrow 5^x . (1 + 5^2) = 650`
`\Rightarrow 5^x . 26 = 650`
`\Rightarrow 5^x = 650 \div 26`
`\Rightarrow 5^x = 25`
`\Rightarrow 5^x = 5^2`
`\Rightarrow x = 2`
Vậy, `x = 2`
`b)`
`(4x + 1)^2 = 25.9`
`\Rightarrow (4x + 1)^2 = 225`
`\Rightarrow (4x + 1)^2 = (+-15^2)`
`\Rightarrow`\(\left[{}\begin{matrix}4x-1=15\\4x-1=-15\end{matrix}\right.\)
`\Rightarrow `\(\left[{}\begin{matrix}4x=16\\4x=-14\end{matrix}\right.\)
`\Rightarrow `\(\left[{}\begin{matrix}x=4\\x=-\dfrac{7}{2}\end{matrix}\right.\)
Vậy, `x \in`\(\left\{-\dfrac{7}{2};4\right\}\)
`c)`
\(2^x+2^{x+3}=144\)
`\Rightarrow 2^x + 2^x . 2^3 = 144`
`\Rightarrow 2^x . (1 + 2^3) = 144`
`\Rightarrow 2^x . 9 = 144`
`\Rightarrow 2^x = 144 \div 9`
`\Rightarrow 2^x = 16`
`\Rightarrow 2^x = 2^4`
`\Rightarrow x = 4`
Vậy, `x = 4`
`d)`
\(3^{2x+2}=9^{x+3}\)
`\Rightarrow `\(3^{2x+2}=\left(3^2\right)^{x+3}\)
`\Rightarrow `\(3^{2x+2}=3^{2x+6}\)
`\Rightarrow 2x + 2 = 2x + 6`
`\Rightarrow 2x - 2x = 6 - 2`
`\Rightarrow 0 = 4 (\text {vô lý})`
Vậy, `x` không có giá trị nào thỏa mãn.
`e)`
\(x^{15}=x^2\)
`\Rightarrow `\(x^{15}-x^2=0\)
`\Rightarrow `\(x^2\cdot\left(x^{13}-1\right)=0\)
`\Rightarrow `\(\left[{}\begin{matrix}x^2=0\\x^{13}-1=0\end{matrix}\right.\)
`\Rightarrow `\(\left[{}\begin{matrix}x=0\\x^{13}=1\end{matrix}\right.\)
`\Rightarrow `\(\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)
Vậy, `x \in`\(\left\{0;1\right\}.\)