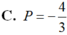a) \(ĐK:x\ge0,x\ne9\)
\(P=\dfrac{x+2\sqrt{x}-10-\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)-\left(\sqrt{x}-3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{x+2\sqrt{x}-10-x+4-\sqrt{x}+3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+2\right)}=\dfrac{\sqrt{x}-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+2\right)}=\dfrac{1}{\sqrt{x}+2}\)
b) \(x^2-13x+36=0\)
\(\Leftrightarrow\left(x-4\right)\left(x-9\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=9\left(ktm\right)\\x=4\left(tm\right)\end{matrix}\right.\)
\(\Leftrightarrow P=\dfrac{1}{\sqrt{x}+2}=\dfrac{1}{\sqrt{4}+2}=\dfrac{1}{4}\)
c) \(P=\dfrac{1}{\sqrt{x}+2}=\dfrac{1}{3}\)
\(\Leftrightarrow\sqrt{x}+2=3\Leftrightarrow\sqrt{x}=1\Leftrightarrow x=1\left(tm\right)\)
d) \(P=\dfrac{1}{\sqrt{x}+2}>\dfrac{1}{9}\)
\(\Leftrightarrow\sqrt{x}+2< 9\Leftrightarrow\sqrt{x}< 7\)
Kết hợp đk:
\(\Rightarrow0\le x< 49\) và \(x\ne0\)
\(a,ĐK:x\ge0;x\ne9\\ b,P=\dfrac{x+2\sqrt{x}-10}{x-\sqrt{x}-6}-\dfrac{\sqrt{x}-2}{\sqrt{x}-3}-\dfrac{1}{\sqrt{x}+2}\\ P=\dfrac{x+2\sqrt{x}-10-\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)-\left(\sqrt{x}-3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+2\right)}\\ P=\dfrac{x+2\sqrt{x}-10-x+4-\sqrt{x}+3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+2\right)}\\ P=\dfrac{\sqrt{x}-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+2\right)}=\dfrac{1}{\sqrt{x}+2}\)
\(x^2-13x+36=0\Leftrightarrow\left[{}\begin{matrix}x=9\\x=4\end{matrix}\right.\\ x=9\Leftrightarrow P=\dfrac{1}{\sqrt{9}+2}=\dfrac{1}{5}\\ x=4\Leftrightarrow P=\dfrac{1}{\sqrt{4}+2}=\dfrac{1}{4}\)
\(c,P=\dfrac{1}{3}\Leftrightarrow\dfrac{1}{\sqrt{x}+2}=\dfrac{1}{3}\Leftrightarrow\sqrt{x}+2=3\Leftrightarrow\sqrt{x}=1\Leftrightarrow x=1\\ d,P>\dfrac{1}{9}\Leftrightarrow\dfrac{1}{\sqrt{x}-2}-\dfrac{1}{9}>0\Leftrightarrow\dfrac{9-\sqrt{x}+2}{9\left(\sqrt{x}-2\right)}>0\\ \Leftrightarrow\dfrac{11-\sqrt{x}}{9\left(\sqrt{x}-2\right)}>0\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}11-\sqrt{x}>0\\\sqrt{x}-2>0\end{matrix}\right.\\\left\{{}\begin{matrix}11-\sqrt{x}< 0\\\sqrt{x}-2< 0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}\sqrt{x}< 11\\\sqrt{x}>2\end{matrix}\right.\\\left\{{}\begin{matrix}\sqrt{x}>11\\\sqrt{x}< 2\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2< \sqrt{x}< 11\\x\in\varnothing\left(\sqrt{x}\in\varnothing\right)\end{matrix}\right.\\ \Leftrightarrow4< x< 121\)