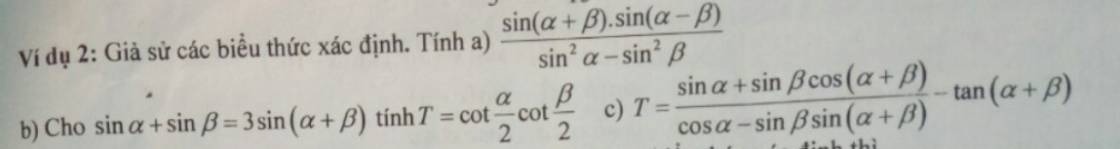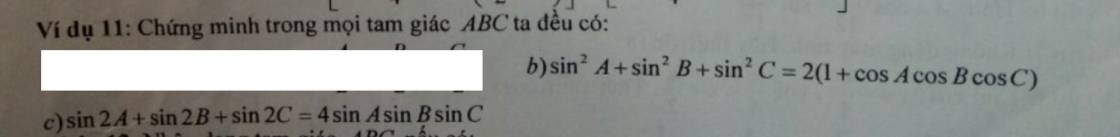Tất cả k dưới đây đều là \(k\in Z\)
6.
\(\Leftrightarrow\sqrt{3}cot\left(3x-\dfrac{\pi}{3}\right)=1\)
\(\Leftrightarrow cot\left(3x-\dfrac{\pi}{3}\right)=\dfrac{1}{\sqrt{3}}\)
\(\Leftrightarrow cot\left(3x-\dfrac{\pi}{3}\right)=cot\left(\dfrac{\pi}{3}\right)\)
\(\Leftrightarrow3x-\dfrac{\pi}{3}=\dfrac{\pi}{3}+k\pi\)
\(\Leftrightarrow3x=\dfrac{2\pi}{3}+k\pi\)
\(\Leftrightarrow x=\dfrac{2\pi}{9}+\dfrac{k\pi}{3}\)
7.
\(\Leftrightarrow\sqrt{3}tan\left(3x-15^0\right)=-1\)
\(\Leftrightarrow tan\left(3x-15^0\right)=-\dfrac{1}{\sqrt{3}}\)
\(\Leftrightarrow tan\left(3x-15^0\right)=tan\left(-30^0\right)\)
\(\Leftrightarrow3x-15^0=-30^0+k180^0\)
\(\Leftrightarrow3x=-15^0+k180^0\)
\(\Leftrightarrow x=-3^0+k60^0\)
8.
\(\Leftrightarrow\sqrt{3}cot\left(3x-30^0\right)=1\)
\(\Leftrightarrow cot\left(3x-30^0\right)=\dfrac{1}{\sqrt{3}}\)
\(\Leftrightarrow cot\left(3x-30^0\right)=cot\left(60^0\right)\)
\(\Leftrightarrow3x-30^0=60^0+k180^0\)
\(\Leftrightarrow3x=90^0+k180^0\)
\(\Leftrightarrow x=30^0+k60^0\)
9.
\(\Leftrightarrow sin\left(3x-\dfrac{\pi}{4}\right)=sin\left(\dfrac{\pi}{2}\right)\)
\(\Leftrightarrow3x-\dfrac{\pi}{4}=\dfrac{\pi}{2}+k2\pi\)
\(\Leftrightarrow3x=\dfrac{3\pi}{4}+k2\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{4}+\dfrac{k2\pi}{3}\)
10.
\(\Leftrightarrow sin\left(2x-\dfrac{\pi}{3}\right)=sin\left(-\dfrac{\pi}{2}\right)\)
\(\Leftrightarrow2x-\dfrac{\pi}{3}=-\dfrac{\pi}{2}+k2\pi\)
\(\Leftrightarrow2x=-\dfrac{\pi}{6}+k2\pi\)
\(\Leftrightarrow x=-\dfrac{\pi}{12}+k\pi\)
11.
\(\Leftrightarrow sin\left(3x-\dfrac{\pi}{4}\right)=sin\left(0\right)\)
\(\Leftrightarrow3x-\dfrac{\pi}{4}=k\pi\)
\(\Leftrightarrow3x=\dfrac{\pi}{4}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{12}+\dfrac{k\pi}{3}\)
12.
\(\Leftrightarrow cos\left(2x-\dfrac{\pi}{3}\right)=cos\left(0\right)\)
\(\Leftrightarrow2x-\dfrac{\pi}{3}=k2\pi\)
\(\Leftrightarrow2x=\dfrac{\pi}{3}+k2\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+k\pi\)
13.
\(\Leftrightarrow cos\left(3x-\dfrac{\pi}{4}\right)=cos\left(\pi\right)\)
\(\Leftrightarrow3x-\dfrac{\pi}{4}=\pi+k2\pi\)
\(\Leftrightarrow3x=\dfrac{5\pi}{4}+k2\pi\)
\(\Leftrightarrow x=\dfrac{5\pi}{12}+\dfrac{k2\pi}{3}\)
14.
\(\Leftrightarrow3x-\dfrac{\pi}{4}=\dfrac{\pi}{2}+k\pi\)
\(\Leftrightarrow3x=\dfrac{3\pi}{4}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{4}+\dfrac{k\pi}{3}\)
15.
\(\Leftrightarrow3x-\dfrac{\pi}{4}=\dfrac{\pi}{4}+k\pi\)
\(\Leftrightarrow3x=\dfrac{\pi}{2}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+\dfrac{k\pi}{3}\)
16.
\(\Leftrightarrow2x-\dfrac{\pi}{3}=-\dfrac{\pi}{4}+k\pi\)
\(\Leftrightarrow2x=\dfrac{\pi}{12}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{24}+\dfrac{k\pi}{2}\)
17.
\(\Leftrightarrow3x-\dfrac{\pi}{4}=k\pi\)
\(\Leftrightarrow3x=\dfrac{\pi}{4}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{12}+\dfrac{k\pi}{3}\)
18.
\(\Leftrightarrow3x-\dfrac{\pi}{4}=\dfrac{\pi}{2}+k\pi\)
\(\Leftrightarrow3x=\dfrac{3\pi}{4}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{4}+\dfrac{k\pi}{3}\)
19.
\(\Leftrightarrow\left[{}\begin{matrix}2sin\left(4x-\dfrac{\pi}{12}\right)-1=0\\cos\left(5x-\dfrac{\pi}{3}\right)-1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}sin\left(4x-\dfrac{\pi}{12}\right)=\dfrac{1}{2}\\cos\left(5x-\dfrac{\pi}{3}\right)=1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x-\dfrac{\pi}{12}=\dfrac{\pi}{6}+k2\pi\\4x-\dfrac{\pi}{12}=\dfrac{5\pi}{6}+k2\pi\\5x-\dfrac{\pi}{3}=k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{16}+\dfrac{k\pi}{2}\\x=\dfrac{11\pi}{48}+\dfrac{k\pi}{2}\\x=\dfrac{\pi}{15}+\dfrac{k2\pi}{5}\end{matrix}\right.\)
20.
ĐKXĐ: \(cos\left(4x-\dfrac{\pi}{12}\right)\ne0\Leftrightarrow x\ne\dfrac{7\pi}{48}+\dfrac{k\pi}{4}\)
Pt tương đương:
\(\left[{}\begin{matrix}\sqrt{3}tan\left(4x-\dfrac{\pi}{12}\right)-1=0\\\sqrt{2}cos\left(2x-\dfrac{\pi}{6}\right)-1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}tan\left(4x-\dfrac{\pi}{12}\right)=\dfrac{1}{\sqrt{3}}\\cos\left(2x-\dfrac{\pi}{6}\right)=\dfrac{\sqrt{2}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x-\dfrac{\pi}{12}=\dfrac{\pi}{6}+k\pi\\2x-\dfrac{\pi}{6}=\dfrac{\pi}{4}+k2\pi\\2x-\dfrac{\pi}{6}=-\dfrac{\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{16}+\dfrac{k\pi}{2}\\x=\dfrac{5\pi}{24}+k\pi\\x=-\dfrac{\pi}{24}+k\pi\end{matrix}\right.\)
21.
\(\Leftrightarrow\left[{}\begin{matrix}sin\left(4x-\dfrac{\pi}{12}\right)=0\\cos\left(5x-\dfrac{\pi}{3}\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x-\dfrac{\pi}{12}=k\pi\\5x-\dfrac{\pi}{3}=\dfrac{\pi}{2}+k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x=\dfrac{\pi}{12}+k\pi\\5x=\dfrac{5\pi}{6}+k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{48}+\dfrac{k\pi}{4}\\x=\dfrac{\pi}{6}+\dfrac{k\pi}{5}\end{matrix}\right.\)
22.
\(\Leftrightarrow\left[{}\begin{matrix}tan\left(4x-\dfrac{\pi}{12}\right)=0\\cot\left(2x-\dfrac{\pi}{6}\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x-\dfrac{\pi}{12}=k\pi\\2x-\dfrac{\pi}{6}=\dfrac{\pi}{2}+k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x=\dfrac{\pi}{12}+k\pi\\2x=\dfrac{2\pi}{3}+k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{48}+\dfrac{k\pi}{4}\\x=\dfrac{\pi}{3}+\dfrac{k\pi}{2}\end{matrix}\right.\)