\(0,1^{2x-1}=100\)
\(\Leftrightarrow0,1^{2x-1}=0,1^{log_{0,1}100}\)
\(\Leftrightarrow2x-1=log_{0,1}100\)
\(\Leftrightarrow2x-1=-2\)
\(\Leftrightarrow2x=-1\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
Chọn A
\(0,1^{2x-1}=100\)
\(\Leftrightarrow0,1^{2x-1}=0,1^{log_{0,1}100}\)
\(\Leftrightarrow2x-1=log_{0,1}100\)
\(\Leftrightarrow2x-1=-2\)
\(\Leftrightarrow2x=-1\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
Chọn A
Tập nghiệm của bất phương trình \(0,{5^{3{\rm{x}} - 1}} > 0,25\) là
A. \(\left( { - \infty ;1} \right)\).
B. \(\left( {1; + \infty } \right)\).
C. \(\left( {0;1} \right)\).
D. \(\left( { - \infty ; - \frac{1}{3}} \right)\).
Giải các phương trình sau:
a) \({\left( {\frac{1}{4}} \right)^{x - 2}} = \sqrt 8 \);
b) \({9^{2x - 1}} = {81.27^x}\);
c) \(2{\log _5}\left( {x - 2} \right) = {\log _5}9\);
d) \({\log _2}\left( {3{\rm{x}} + 1} \right) = 2 - {\log _2}\left( {x - 1} \right)\).
Nếu \({2^\alpha } = 9\) thì \({\left( {\frac{1}{{16}}} \right)^{\frac{\alpha }{8}}}\) có giá trị bằng
A. \(\frac{1}{3}\).
B. 3.
C. \(\frac{1}{9}\).
D. \(\frac{1}{{\sqrt 3 }}\).
Nếu \({a^{\frac{1}{2}}} = b\left( {a > 0,a \ne 1} \right)\) thì
A. \({\log _{\frac{1}{2}}}a = b\).
B. \(2{\log _a}b = 1\).
C. \({\log _a}\frac{1}{2} = b\).
D. \({\log _{\frac{1}{2}}}b = a\).
Rút gọn biểu thức \({\left[ {{{\left( {\frac{1}{3}} \right)}^2}} \right]^{\frac{1}{4}}}.{\left( {\sqrt 3 } \right)^5}\), ta được
A. \(\sqrt 3 \).
B. \(3\sqrt 3 \).
C. \(\frac{1}{{\sqrt 3 }}\).
D. 9.
Viết công thức biểu thị \(y\) theo \(x\), biết \(2{\log _2}y = 2 + \frac{1}{2}{\log _2}x\).
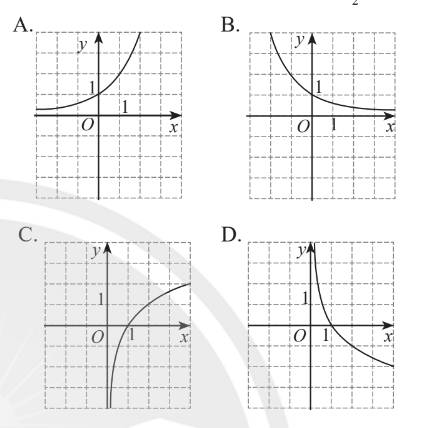
Hình nào vẽ đồ thị của hàm số \(y = {\log _{\frac{1}{2}}}x\)?
a) \(\left(\dfrac{1}{9}\right)^{x+1}>\dfrac{1}{81}\);
b) \(\left(\sqrt[4]{3}\right)^x\le27.3^x\);
c) \(log_2\left(x+1\right)\le log_2\left(2-4x\right)\).
Số nguyên \(x\) nhỏ nhất thoả mãn \({\log _{0,1}}\left( {1 - 2x} \right) > - 1\) là
A. \(x = 0\).
B. \(x = 1\).
C. \(x = - 5\).
D. \(x = - 4\).