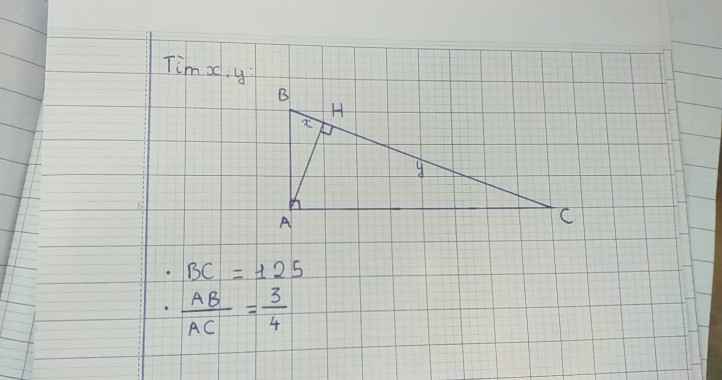
\(\dfrac{AB}{AC}=\dfrac{3}{4}\) (1)
\(\Rightarrow AB=\dfrac{3}{4}AC\)
Áp dụng định lý pytago, ta có:
\(BC^2=AB^2+AC^2\)
\(\Leftrightarrow125^2=\left(\dfrac{3}{4}AC\right)^2+AC^2\)
\(\Leftrightarrow125^2=\dfrac{25}{16}AC^2\)
\(\Leftrightarrow AC^2=10000\)
\(\Leftrightarrow AC=100\left(đ.v.đ.d\right)\)
(1)\(\Rightarrow\dfrac{AB}{100}=\dfrac{3}{4}\)
\(\Leftrightarrow AB=75\left(đ.v.đ.d\right)\)
Áp dụng hệ thức lượng, ta có:
\(AB^2=BH.BC\)
\(\Leftrightarrow75^2=125x\)
\(\Leftrightarrow x=45\left(đ.v.đ.d\right)\)
Ta có: \(x+y=125\)
\(\Rightarrow y=125-x=125-45=80\left(đ.v.đ.d\right)\)