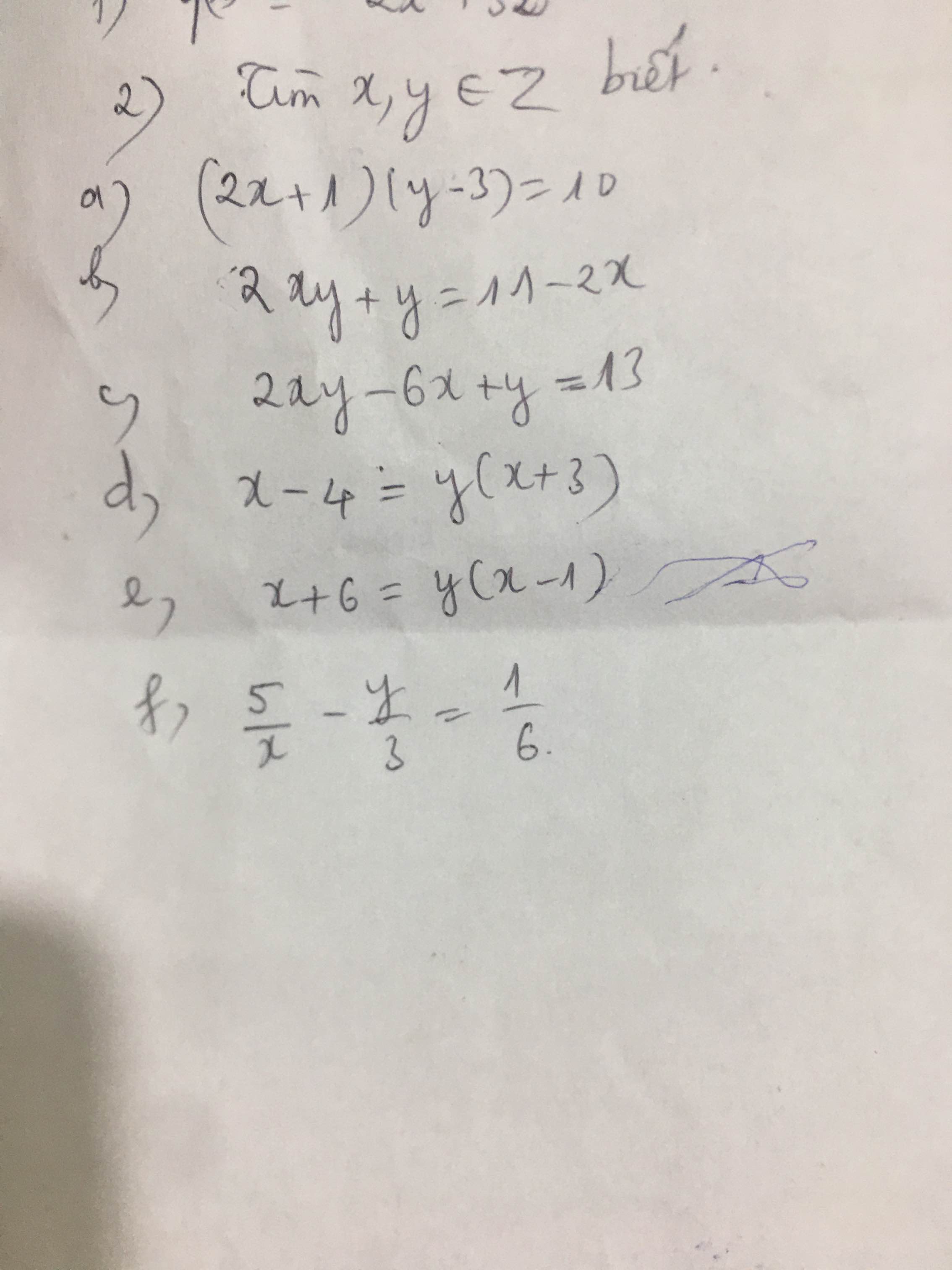Question

Answer 1

\(a,x=0\Leftrightarrow A=\dfrac{-4}{1}=-4\\ x=3\Leftrightarrow A=\dfrac{-1}{4}\\ b,A\in Z\Leftrightarrow\dfrac{x-4}{x-1}\in Z\Leftrightarrow1-\dfrac{3}{x-1}\in Z\\ \Leftrightarrow3⋮x-1\Leftrightarrow x-1\inƯ\left(3\right)=\left\{-3;-1;1;3\right\}\\ \Leftrightarrow x\in\left\{-2;0;2;4\right\}\\ c,A\in Q\Leftrightarrow\left\{{}\begin{matrix}x-4\in Z\\x+1\in Z\\x+1\ne0\end{matrix}\right.\Leftrightarrow x\in Z\backslash\left\{-1\right\}\)

\(d,A>0\Leftrightarrow\dfrac{x-4}{x+1}>0\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-4>0\\x+1>0\end{matrix}\right.\\\left\{{}\begin{matrix}x-4< 0\\x+1< 0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x>4\\x< -1\end{matrix}\right.\\ A< 0\Leftrightarrow\dfrac{x-4}{x+1}< 0\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-4>0\\x+1< 0\end{matrix}\right.\\\left\{{}\begin{matrix}x-4< 0\\x+1>0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\in\varnothing\\-1< x< 4\end{matrix}\right.\)

 

Answer 2

a) Khi x=0:

\(A=\dfrac{x-4}{x+1}\left(đk:x\ne-1\right)=\dfrac{0-4}{0+1}=\dfrac{-4}{1}=-4\)

Khi x=3:

\(A=\dfrac{x-4}{x+1}=\dfrac{3-4}{3+1}=-\dfrac{1}{4}\)

b) \(A=\dfrac{x-4}{x+1}=\dfrac{x+1}{x+1}-\dfrac{5}{x+1}=1-\dfrac{5}{x+1}\in Z\)

\(\Rightarrow x+1\inƯ\left(5\right)=\left\{-5;-1;1;5\right\}\)

\(\Rightarrow x\in\left\{-6;-2;0;4\right\}\)

c) Để A là số hữu tỉ: \(x\ne-1\) và \(x\in Z\)

d) \(A=\dfrac{x-4}{x+1}>0\Leftrightarrow\) \(\left[{}\begin{matrix}\left\{{}\begin{matrix}x-4>0\\x+1>0\end{matrix}\right.\\\left\{{}\begin{matrix}x-4< 0\\x+1< 0\end{matrix}\right.\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x>4\\x< -1\end{matrix}\right.\)

\(A=\dfrac{x-4}{x+1}< 0\Leftrightarrow\)\(\left\{{}\begin{matrix}x-4< 0\\x+1>0\end{matrix}\right.\)\(\Leftrightarrow-1< x< 4\)

g) \(A=\dfrac{x-4}{x+1}=3\) 

\(\Leftrightarrow x-4=3x+3\Leftrightarrow2x=-7\Leftrightarrow x=-\dfrac{7}{2}\left(tm\right)\)

Answer 3

a)
thay x=0 vào A ta có
\(A=\dfrac{0-4}{0+1}=-4\)
thay x=3 vào biểu thức A ta có
\(A=\dfrac{3-4}{3+1}=-\dfrac{1}{4}\)
A>0
=>\(\dfrac{x-4}{x+1}>0\)
trường hợp 1 
x-4>0 và x+1 > 0
x>4     và  x>-1
trường hợp 2
x-4<0  và  x+1<0
x<4      và  x<-1
\(\dfrac{x+4}{x-1}< 0\)
TH1
x+4<0 và x-1>0
x<-4 và x>1
TH2
x+4>0 và x-1<0
x>-4 và x<1
\(\dfrac{x+4}{x-1}=3\left(x\ne1\right)< =>\dfrac{x+4}{x-1}-3=0< =>\dfrac{x+4-3\left(x-1\right)}{x-1}=0< =>\dfrac{x+4-3x+1}{x-1}=0< =>\dfrac{-2x+5}{x-1}=0 < =>-2x+5=0< =>-2x=-5< =>x=\dfrac{5}{2}\)