Học tại trường
Chưa có thông tin
Đến từ
Chưa có thông tin , Chưa có thông tin
Số lượng câu hỏi
6
Số lượng câu trả lời
11102
Điểm GP
4169
Điểm SP
8392











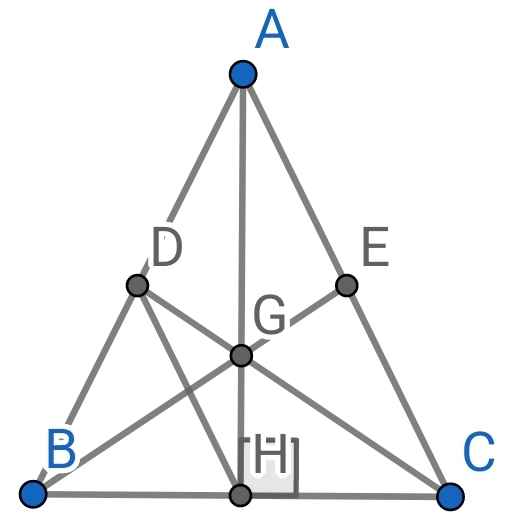 a) Do ∆ABC cân tại A (gt)
a) Do ∆ABC cân tại A (gt)