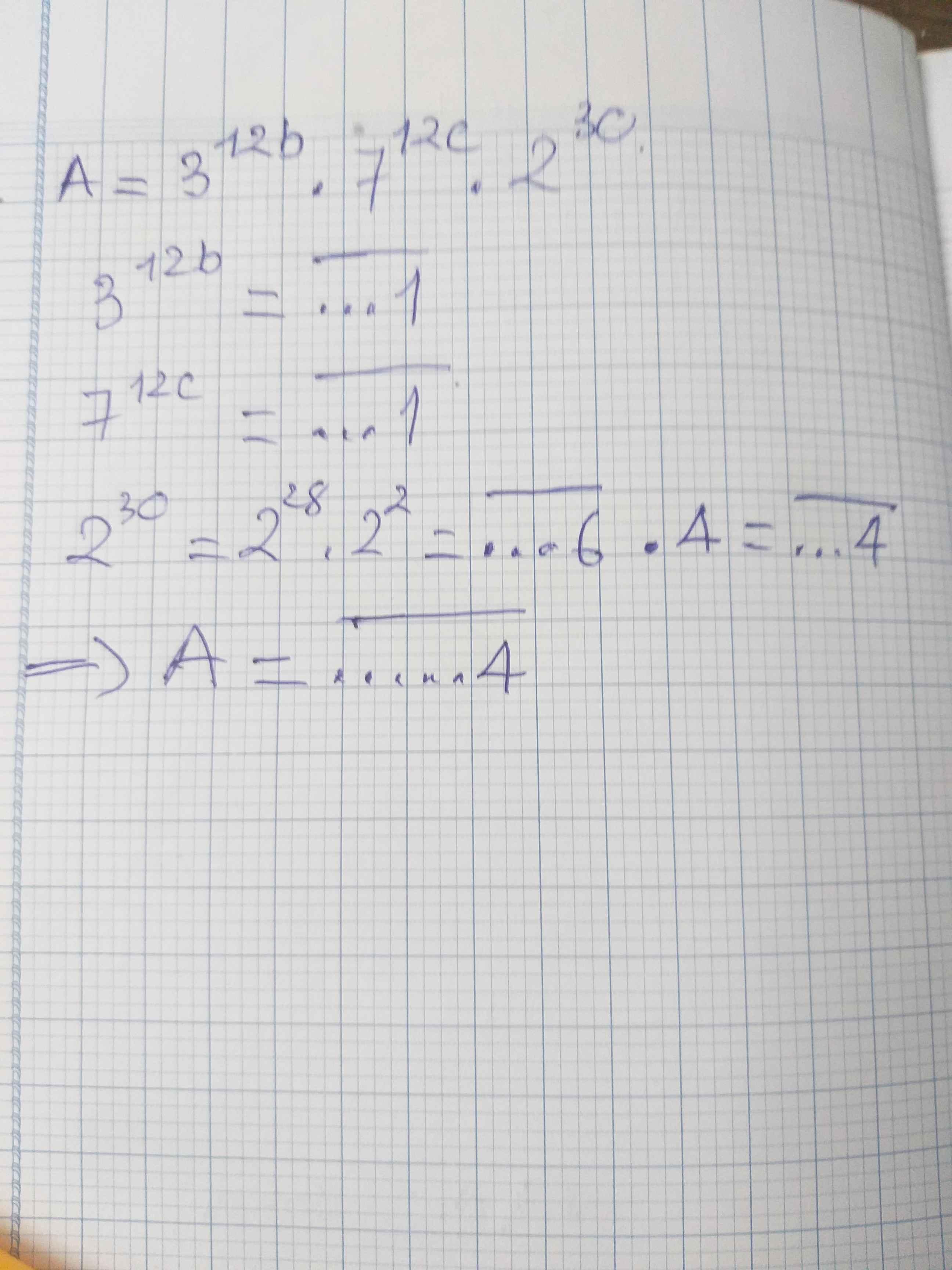(4 x\(^2\))\(^{^8}\) : (4 x\(^2\))\(^{^4}\) : (4 x\(^2\))\(^{^2}\)
Bài 6. Lũy thừa với số mũ tự nhiên
\(\left(4x^2\right)^8:\left(4x^2\right)^4:\left(4x^2\right)^2\\ =\left(4x^2\right)^{8-4-2}\\ =\left(4x^2\right)^2\\ =4^2\cdot\left(x^2\right)^2\\ =16x^4\)
Đúng 2
Bình luận (0)
(4 x22)88 : (4 x22)44 : (4 x22)2
Đọc tiếp
(4 x) : (4 x) : (4 x)
Sửa đề: (4 . 2)8 : (4 . 2)4 : (4 . 2)2
= 88 : 84 : 82
= 84 : 82
=82
Đúng 1
Bình luận (1)
\(\left(4\times22\right)^{88}:\left(4\times22\right)^{44}:\left(4\times22\right)^2\)
\(=\left(4\times22\right)^{88-44-2}\)
\(=\left(4\times22\right)^{42}\)
Đúng 2
Bình luận (0)
tìm số tận cùng của
a] 3^12 b] 7 ^12 c] 2^ 30
a) Ta có:
`3^12=3^(3*4)`
Mà: \(3^{4n}=\overline{....1}\)
`=>3^12` có chữ số tận cùng là 1
b) Ta có:
`7^12=7^(4*3)`
Mà: \(7^{4n}=\overline{....1}\)
`=>7^12` có chữ số tận cùng là 1
c) Ta có:
`2^30=(2^2)^15`
`=4^15=4^14*4=4^(2*7)*4`
Mà: \(4^{2n}=\overline{...6}\)
`=>\(4^{14}=\overline{...6}\Rightarrow4^{14}\cdot4=\overline{...6}\cdot4=\overline{....4}\)
Vậy: ...
Đúng 3
Bình luận (0)