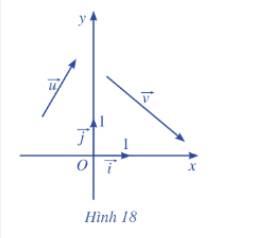
Trong mặt phẳng toạ độ Oxy, cho \(\overrightarrow i \)và \(\overrightarrow j \) là vectơ đơn vị trên trục hoành Ox và ở trên trục tung Oy
a) Tính \({\overrightarrow i ^2};{\overrightarrow j ^2};\overrightarrow i .\overrightarrow j .\)
b) Cho \(\overrightarrow u = \left( {{x_1},{y_1}} \right)\), \(\overrightarrow v = \left( {{x_2},{y_2}} \right)\). Tính tích vô hướng \(\overrightarrow u .\overrightarrow v \).
a) Ta có: \({\overrightarrow i ^2} = {\left| {\overrightarrow i } \right|^2} = 1;{\overrightarrow j ^2} = {\left| {\overrightarrow j } \right|^2};\overrightarrow i .\overrightarrow j = 0\)(vì \(\overrightarrow i \bot \overrightarrow j \) )
b) Ta có: \(\overrightarrow u .\overrightarrow v = \left( {{x_1}\overrightarrow i + {y_1}\overrightarrow j } \right).\left( {{x_2}\overrightarrow i + {y_2}\overrightarrow j } \right) = {x_1}{x_2}.{\overrightarrow i ^2} + {x_1}{y_2}.\left( {\overrightarrow i .\overrightarrow j } \right) + {y_1}{x_2}.\left( {\overrightarrow j .\overrightarrow i } \right) + {y_1}{y_2}.{\overrightarrow j ^2} = {x_1}{x_2} + {y_1}{y_2}\)