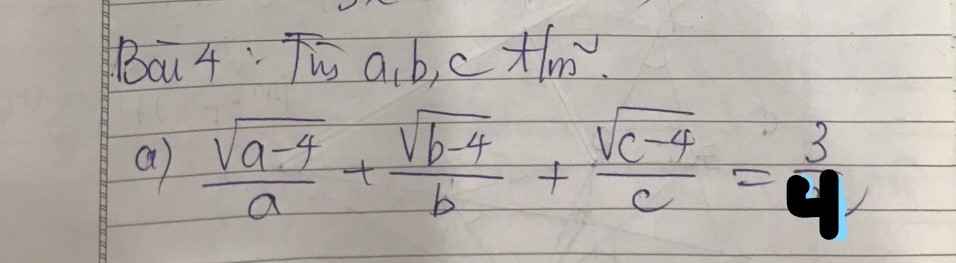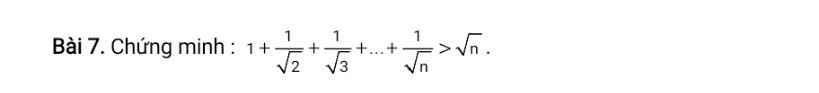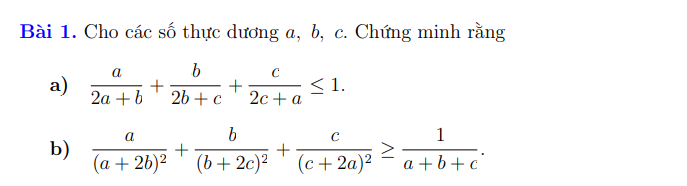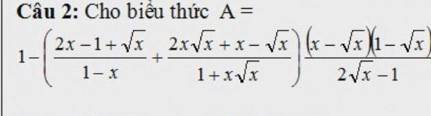\(\dfrac{\sqrt{a-4}}{a}+\dfrac{\sqrt{b-4}}{b}+\dfrac{\sqrt{c-4}}{c}=\dfrac{3}{4}\) (ĐK: \(a\ge4;b\ge4;c\ge4\))
Áp dụng AM-GM có:
\(2\sqrt{4\left(a-4\right)}\le4+a-4=a\)
\(\Rightarrow\dfrac{\sqrt{a-4}}{a}\le\dfrac{1}{4}\)
Tương tự cũng có: \(\dfrac{\sqrt{b-4}}{b}\le\dfrac{1}{4}\);\(\dfrac{\sqrt{c-4}}{c}\le\dfrac{1}{4}\)
Cộng vế với vế \(\Rightarrow VT\le\dfrac{3}{4}\)
Dấu "=" xảy ra khi \(\left\{{}\begin{matrix}4=a-4\\4=b-4\\4=c-4\end{matrix}\right.\)\(\Rightarrow a=b=c=8\) (tm)
Vậy...