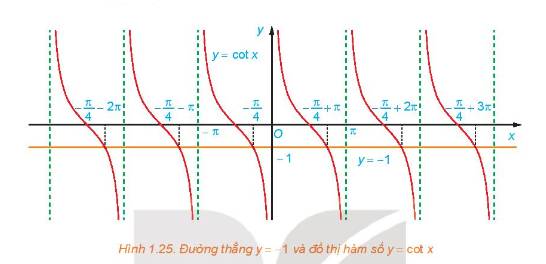
a) \(\cot x = 1\; \Leftrightarrow \cot x = \cot \frac{\pi }{4}\;\;\; \Leftrightarrow x = \frac{\pi }{4} + k\pi \;\left( {k \in \mathbb{Z}} \right)\)
b) \(\sqrt 3 \cot x + 1 = 0\;\;\; \Leftrightarrow \sqrt 3 \cot x = - 1\; \Leftrightarrow \cot x = - \frac{{\sqrt 3 }}{3}\;\; \Leftrightarrow \cot x = \cot \left( { - \frac{\pi }{3}} \right)\)
\( \Leftrightarrow x = - \frac{\pi }{3} + k\pi \;\left( {k \in \mathbb{Z}} \right)\)