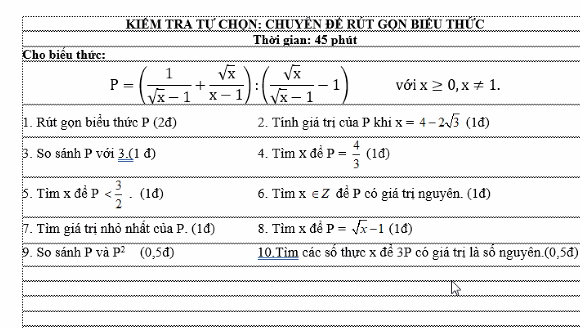
a: Khi x=49 thì \(A=\dfrac{\sqrt{49}-5}{\sqrt{49}}=\dfrac{7-5}{7}=\dfrac{2}{7}\)
b: P=A*B
\(=\dfrac{\sqrt{x}-5}{\sqrt{x}}\left(\dfrac{\sqrt{x}}{\sqrt{x}-5}-\dfrac{3\sqrt{x}}{x-25}\right)\)
\(=\dfrac{\sqrt{x}-5}{\sqrt{x}}\cdot\left(\dfrac{\sqrt{x}}{\sqrt{x}-5}-\dfrac{3\sqrt{x}}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}\right)\)
\(=\dfrac{\sqrt{x}-5}{\sqrt{x}}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}+5\right)-3\sqrt{x}}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x}+5-3\right)}{\sqrt{x}\left(\sqrt{x}+5\right)}=\dfrac{\sqrt{x}+2}{\sqrt{x}+5}\)
c: Vì \(\sqrt{x}+2>=2>0\forall x\) thỏa mãn ĐKXĐ
và \(\sqrt{x}+5>=5>0\forall x\) thỏa mãn ĐKXĐ
nên \(P=\dfrac{\sqrt{x}+2}{\sqrt{x}+5}>0\) với mọi x thỏa mãn ĐKXĐ
Vì \(\sqrt{x}+2< \sqrt{x}+5\)
nên \(P=\dfrac{\sqrt{x}+2}{\sqrt{x}+5}< 1\)
=>\(0< P< 1\)
=>P không thể là số nguyên