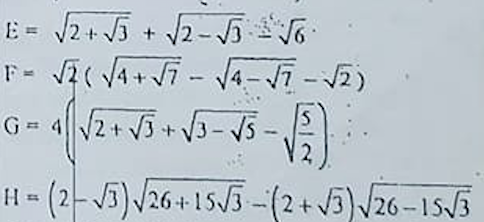4:
\(A=\sqrt{\left(x+1\right)^2}+\sqrt{\left(x-1\right)^2}\)
=>\(A=\left|x+1\right|+\left|x-1\right|=\left|x+1\right|+\left|1-x\right|>=\left|x+1+1-x\right|=2\)
Dấu = xảy ra khi -1<=x<=1
3:
a: \(P=\dfrac{\sqrt{x}-\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{x-1-x+4}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}-1\right)}{3}=\dfrac{\sqrt{x}-1}{3\sqrt{x}}\)
b: P=1/4
=>\(\dfrac{\sqrt{x}-1}{3\sqrt{x}}=\dfrac{1}{4}\)
=>\(4\sqrt{x}-4=3\sqrt{x}\)
=>căn x=4
=>x=16
c: Khi x=4+2căn 3 thì \(P=\dfrac{\sqrt{3}+1-1}{3\left(\sqrt{3}+1\right)}=\dfrac{\sqrt{3}}{3\left(\sqrt{3}+1\right)}=\dfrac{1}{3+\sqrt{3}}=\dfrac{3-\sqrt{3}}{6}\)