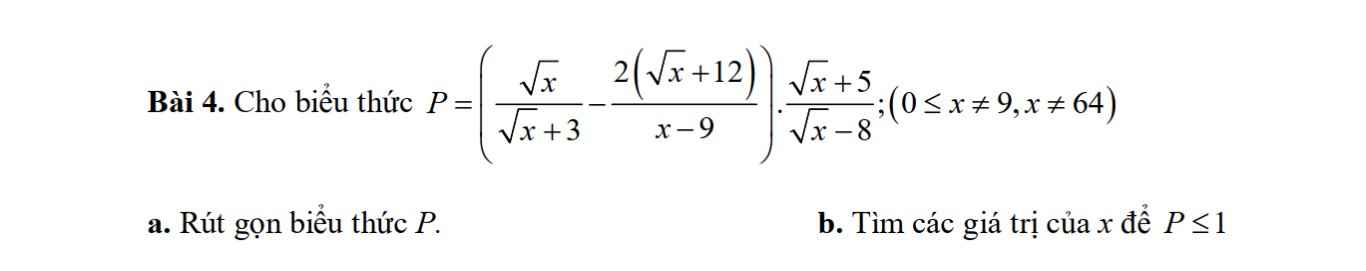\(a,P=\left(\dfrac{\sqrt{x}}{\sqrt{x}+3}-\dfrac{2\left(\sqrt{x}+12\right)}{x-9}\right)\cdot\dfrac{\sqrt{x}+5}{\sqrt{x}-8}\left(0\le x\ne9;x\ne64\right)\\ P=\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)-2\sqrt{x}-24}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\cdot\dfrac{\sqrt{x}+5}{\sqrt{x}-8}\\ P=\dfrac{x-5\sqrt{x}-24}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\cdot\dfrac{\sqrt{x}-5}{\sqrt{x}+8}\\ P=\dfrac{\left(\sqrt{x}-8\right)\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\cdot\dfrac{\sqrt{x}-5}{\sqrt{x}-8}=\dfrac{\sqrt{x}-5}{\sqrt{x}-3}\)
\(b,P\le1\Leftrightarrow P-1\le0\Leftrightarrow\dfrac{\sqrt{x}-5}{\sqrt{x}-3}\le0\\ \Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}\sqrt{x}-5\ge0\\\sqrt{x}-3\le0\end{matrix}\right.\\\left\{{}\begin{matrix}\sqrt{x}-5\le0\\\sqrt{x}-3\ge0\end{matrix}\right.\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge25\\x\le9\end{matrix}\right.\\\left\{{}\begin{matrix}x\le25\\x\ge9\end{matrix}\right.\end{matrix}\right.\Leftrightarrow9\le x\le25\)
a: Ta có: \(P=\left(\dfrac{\sqrt{x}}{\sqrt{x}+3}-\dfrac{2\left(\sqrt{x}+12\right)}{x-9}\right)\cdot\dfrac{\sqrt{x}+5}{\sqrt{x}-8}\)
\(=\dfrac{x-5\sqrt{x}-24}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\cdot\dfrac{\sqrt{x}+5}{\sqrt{x}-8}\)
\(=\dfrac{\left(\sqrt{x}-8\right)\left(\sqrt{x}+3\right)\left(\sqrt{x}+5\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)\left(\sqrt{x}-8\right)}\)
\(=\dfrac{\sqrt{x}+5}{\sqrt{x}-3}\)
b: Để \(P\le1\) thì \(\dfrac{\sqrt{x}+5-\sqrt{x}+3}{\sqrt{x}-3}\le0\)
hay x<9
Kết hợp ĐKXĐ, ta được: \(0\le x< 9\)