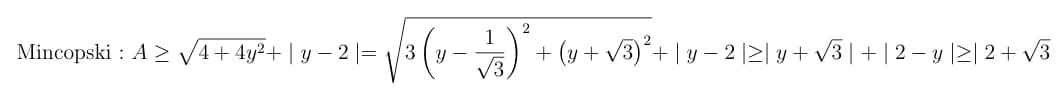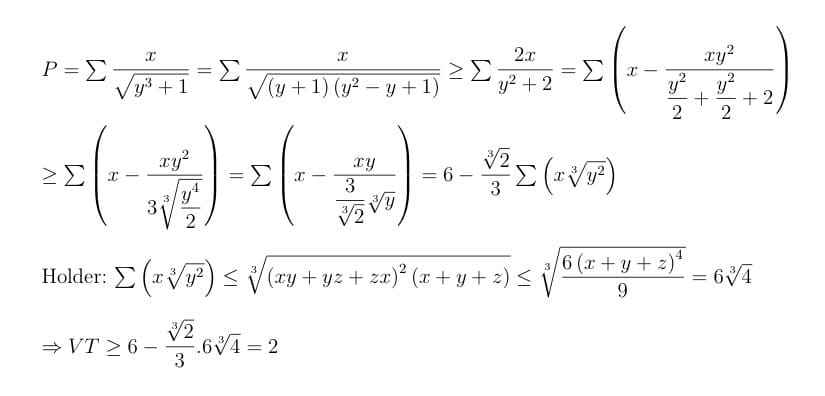Tại anh Đạt chỉ bảo là tìm quy luật của dãy chứ không phải tìm đáp án đúng nên em góp 1 cái quy luật dễ nhất nha:D

Quy luật của dãy đề cũ là: Lập phương của dãy số nguyên tố không chia hết cho 3.

C49.3: Câu dễ nhất ạ:((

Ta có: \(\dfrac{1}{a^2+1}+\dfrac{1}{b^2+1}+\dfrac{1}{c^2+1}\le\dfrac{1}{2a}+\dfrac{1}{2b}+\dfrac{1}{2c}=\dfrac{ab+bc+ca}{2abc}=\dfrac{3abc}{2abc}=\dfrac{3}{2}\)

Dấu ''='' xảy ra khi a=b=c=1

C43.4:

Ủa em đánh lộn ạ.

Đoạn dòng 2 trở đi là như thế này ạ:

\(\left(\Sigma\dfrac{a\sqrt{a}}{\sqrt{c+3}}\right)^3\left(\Sigma\left(c+3\right)\right)\ge\left(a+b+c\right)^3\Leftrightarrow\Sigma\dfrac{a\sqrt{a}}{\sqrt{c+3}}\ge\sqrt{\dfrac{\left(a+b+c\right)^3}{a+3+b+3+c+3}}=\dfrac{3}{2}\)

\(P=\dfrac{a\sqrt{a}}{\sqrt{c+3}}+\dfrac{b\sqrt{b}}{\sqrt{a+3}}+\dfrac{c\sqrt{c}}{\sqrt{b+3}}\)

Ta có: Theo Holder:

\(\left(\Sigma\dfrac{a\sqrt{a}}{\sqrt{a+3}}\right)^2\left(\Sigma\left(a+3\right)\right)\ge\left(a+b+c\right)^3\)

\(\Leftrightarrow\Sigma\dfrac{a\sqrt{a}}{\sqrt{a+3}}\ge\sqrt{\dfrac{\left(a+b+c\right)^3}{a+3+b+3+c+3}}=\dfrac{3}{2}\)

Dấu ''='' xảy ra khi và chỉ khi a=b=c=1

Nhìn cách đánh tấu hài quá má :"((

Đọc nhầm đề nên ngồi nãy giờ không ra ai dè nó dễ:"(((

C41.2:

Ta có: \(2\Sigma\left(\dfrac{x^2}{2}+\dfrac{x}{yz}\right)=\Sigma\left(x^2+\dfrac{x}{yz}+\dfrac{x}{yz}\right)=\Sigma\left(x^2+\dfrac{y}{xz}+\dfrac{z}{xy}\right)\ge\left(3+3+3\right)=9\)

\(\Rightarrow\Sigma\left(\dfrac{x^2}{2}+\dfrac{x}{yz}\right)\ge\dfrac{9}{2}\)

Dấu ''='' xảy ra khi x=y=z=1.

Lúc bình phương lên em không có ghi đk bình phương tại nhìn rối quá nhưng sau đó em đã thay ngược lại để loại nghiệm ngoại lai nha.

Làm câu hệ vậy+((

C33.4:

Ta có: \(\left\{{}\begin{matrix}x^2+y^2=1\left(1\right)\\21x+3y+48x^2-48y^2+28xy=69\left(2\right)\end{matrix}\right.\)

Từ pt (1) ta có:  \(x^2+y^2=1\Rightarrow y^2=1-x^2\)

Thay vào pt (2) ta được: \(21x+3\sqrt{1-x^2}+48x^2-48\left(1-x^2\right)+28x\sqrt{1-x^2}-69=0\)

\(\Leftrightarrow3\sqrt{\left(1-x\right)\left(1+x\right)}+28x\sqrt{\left(1-x\right)\left(1+x\right)}-21\sqrt{\left(1-x\right)\left(1-x\right)}-48\left(1-x^2\right)-48\left(1-x^2\right)=0\)

\(\Leftrightarrow\sqrt{1-x}\left(3\sqrt{1+x}+28x\sqrt{1+x}-21\sqrt{1-x}-96\left(1+x\right)\sqrt{1-x}\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{1-x}=0\\3\sqrt{1+x}+28x\sqrt{1+x}-21\sqrt{1-x}-96\left(1+x\right)\sqrt{1-x}=0\end{matrix}\right.\)

+ Nếu \(\sqrt{1-x}=0\Leftrightarrow1-x=0\Leftrightarrow x=1\Rightarrow y=0\)

+Nếu \(3\sqrt{1+x}+28x\sqrt{1+x}-21\sqrt{1-x}-96\left(1+x\right)\sqrt{1-x}=0\)

\(\Leftrightarrow3\sqrt{1+x}+28x\sqrt{1+x}=21\sqrt{1-x}+96\left(1+x\right)\sqrt{1-x}\)

\(\Leftrightarrow784x^3+952x^2+177x+9=-9216x^3-13248x^2+8775x+13689\)

\(\Leftrightarrow10000x^3+14200x^2-8598x-13680=0\)

\(\Leftrightarrow x=\dfrac{24}{25}\Rightarrow y=\dfrac{7}{25}\)

Thay \(x=\dfrac{24}{24};y=\dfrac{7}{25}\) vào hệ pt ta thấy thoả mãn

\(x=\dfrac{24}{25};y=\dfrac{7}{25}\) là 1 cặp nghiệm của hệ pt

Vậy hệ pt có nghiệm: \(\left(x;y\right)\in\left\{\left(\dfrac{24}{25};\dfrac{7}{25}\right),\left(1;0\right)\right\}\)