Question

Tìm ba số \(x,y,z\) biết rằng :

                       \(\dfrac{x}{2}=\dfrac{y}{3};\dfrac{y}{4}=\dfrac{z}{5};x+y-z=10\)

Answer 1

Do đó: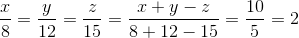

Nên x = 2.8 = 16

y = 2.12 = 24

z= 2. 15 = 30

Answer 2

Ta có \(\dfrac{x}{2}=\dfrac{y}{3}\Rightarrow\dfrac{x}{2.4}=\dfrac{y}{3.4}\Rightarrow\dfrac{x}{8}=\dfrac{y}{12}\)

\(\dfrac{y}{4}=\dfrac{z}{5}\Rightarrow\dfrac{y}{4.3}=\dfrac{z}{3.5}\Rightarrow\dfrac{y}{12}=\dfrac{z}{15}\)

Do đó \(\dfrac{x}{8}=\dfrac{y}{12}=\dfrac{z}{15}và\) \(x+y-z=10\)

Áp dụng tính chất của dãy tỉ số bằng nhau,ta được:

\(\dfrac{x}{8}=\dfrac{y}{12}=\dfrac{z}{15}=\dfrac{x+y-z}{8+12-15}=\dfrac{10}{5}=2\)

Với\(\dfrac{x}{8}=2\Rightarrow x=16\)

\(\dfrac{y}{12}=2\Rightarrow y=24\)

\(\dfrac{z}{15}=2\Rightarrow z=30\)

Vậy x=16, y=24 và z=30

Answer 3

Ta có:\(\dfrac{x}{2}=\dfrac{y}{3}\Rightarrow\dfrac{x}{2}\div4=\dfrac{y}{3}\div4\)hay \(\dfrac{x}{8}=\dfrac{y}{12}\) (1)

Ta có:\(\dfrac{y}{4}=\dfrac{z}{5}\Rightarrow\dfrac{y}{4}\div3=\dfrac{z}{5}\div3\) hay \(\dfrac{y}{12}=\dfrac{z}{15}\) (2)

Từ (1) và (2), ta có:\(\dfrac{x}{8}=\dfrac{y}{12}=\dfrac{z}{15}=\dfrac{x+y-z}{8+12-15}=\dfrac{10}{5}=2\)

Vậy: \(\dfrac{x}{8}=2\Rightarrow x=2\times8=16\)

\(\dfrac{y}{12}=2\Rightarrow y=2\times12=24\)

\(\dfrac{z}{15}=2\Rightarrow z=2\times15=30\)

Answer 4

Ta có: \(\dfrac{x}{2}=\dfrac{y}{3}\)

=> \(\dfrac{x}{8}=\dfrac{y}{12}\left(1\right)\)

Ta có: \(\dfrac{y}{4}=\dfrac{z}{5}\)

=> \(\dfrac{y}{12}=\dfrac{z}{15}\left(2\right)\)

Từ (1) và (2) => \(\dfrac{x}{8}=\dfrac{y}{12}=\dfrac{z}{15}\)

Áp dụng tính chất của dãy tỉ số bằng nhau ta được:

\(\dfrac{x}{8}=\dfrac{y}{12}=\dfrac{z}{15}=\dfrac{x+y-z}{8+12-15_{ }}=\dfrac{10}{5}=2\) (Vì x+y-z = 10)

=> \(\dfrac{x}{8}=2\)

\(\dfrac{y}{12}=2\)

\(\dfrac{z}{15}=2\)

=> x = 2 . 8 = 16

y = 2 . 12 = 24

z = 2 . 15 = 30

Vậy x = 16; y = 24 và z = 30