\(P=x+y+\dfrac{10}{x+y}=2\sqrt{10}\)
Dấu "=" xảy ra khi: \(\left\{{}\begin{matrix}x+y=\sqrt{10}\\x^2+y=8\end{matrix}\right.\)
\(\Rightarrow\left(x;y\right)=\left(\dfrac{1+\sqrt{33-4\sqrt{10}}}{2};\dfrac{2\sqrt{10}-1-\sqrt{33-4\sqrt{10}}}{2}\right)\)
1) cho các số thực dương a,b thỏa mãn \(3a+b\le1\). Tìm Min của \(P=\dfrac{1}{a}+\dfrac{1}{\sqrt{ab}}\)
2) Với hai số thực a,b không âm thỏa mãn \(a^2+b^2=4\). Tìm Max \(M=\dfrac{ab}{a+b+2}\)
3) Cho x,y khác 0 thỏa mãn \(\left(x+y\right)xy=x^2+y^2-xy\). Tìm Max \(A=\dfrac{1}{x^3}+\dfrac{1}{y^3}\)
Cho x,y>0 thỏa mãn (x+\(\sqrt{1+x^2}\))(y+\(\sqrt{1+y^2}\))=2018. Tìm GTNN của P=x+yGiúp mk với ạ, please
cho \(x,y,z\ge0\) thỏa mãn \(x+y+z=6\). tìm GTLN và GTNN của biểu thức \(A=x^2+y^2+z^2\)
Cho : x,y,z là các số dương thỏa mãn \(\sqrt{x+2}-x^3=\sqrt{x+2}-y^3\)
tìm GTNN của \(x^2+2xy-y^2+2y+2020\)
cho x,y thỏa mãn 1≤y≤2 và xy+2≥2y. tìm GTNN của \(M=\dfrac{x^2+4}{y^2+1}\)
Cho 3 số thực không âm x ,y ,z thỏa mãn x + y + z = 2 . Chứng minh rằng : x + 2y + z >= (2 - x)(2 - y)(2 - z)
Tìm nghiệm nguyên không âm thỏa mãn phương trình: x^2=y^2 + căn y+1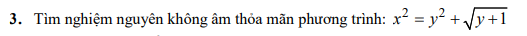
cho x,y>0 thỏa mãn \(x+y\le1\). tìm GTNN của \(T=\left(1+x+\dfrac{1}{x}\right)^3+\left(1+y+\dfrac{1}{y}\right)^3\)
