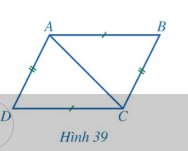
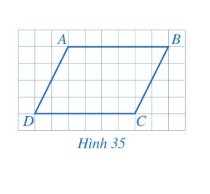
a, Tứ giác ABCD có:
\(\widehat {ABC} + \widehat {BCD} + \widehat {CDA} + \widehat {DAB} = {360^0}\)
\(\widehat {ABC} + \widehat {DAB} + \widehat {ABC} + \widehat {DAB} = {360^0}\)(do \(\widehat {DAB} = \widehat {BCD};\widehat {ABC} = \widehat {CDA}\))
\(\begin{array}{l}2\widehat {ABC} + 2\widehat {DAB} = {360^0}\\\widehat {ABC} + \widehat {DAB} = \dfrac{{{{360}^0}}}{2} = {180^0}\end{array}\)
b, Ta có: \(\widehat {xAD} + \widehat {DAB} = {180^0}\)(do tia Ax là tia đối của tia AB)
Nên
\(\begin{array}{l}\widehat {xAD} + \widehat {DAB} = \widehat {ABC} + \widehat {DAB}\\ \Rightarrow \widehat {xAD} = \widehat {ABC}\end{array}\)
Suy ra AD//BC (hai góc đồng vị bằng nhau)
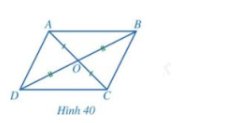
c, Vì AD//BC \( \Rightarrow \widehat {ADB} = \widehat {DBC}\) (2 góc so le trong)
Xét \(\Delta A{\rm{D}}B\) có \(\widehat {ABD} = {180^0} - \widehat {ADB} - \widehat {DAB} = {180^0} - \widehat {DBC} - \widehat {BCD}\left( 1 \right)\)
( vì \(\widehat {ADB} = \widehat {DBC};\widehat {DAB} = \widehat {BCD})\)
Xét \(\Delta CDB\) có: \(\widehat {BDC} = {180^0} - \widehat {DBC} - \widehat {BCD}\left( 2 \right)\)
Từ (1), (2) suy ra \(\widehat {ABD} =\widehat {BDC}\)
Xét \(\Delta ADB\) và \(\Delta BCD\)có:
\(\left. \begin{array}{l}DBchung\\\widehat {ABD} = \widehat {BDC}\\\widehat {ABD} = \widehat {DBC}\end{array} \right\} \Rightarrow \Delta A{\rm{D}}B = \Delta C{\rm{D}}B \Rightarrow A{\rm{D}} = BC,AB = CB\)
Suy ra tứ giác ABCD có cặp cạnh đối bằng nhau nên ABCD là hình bình hành.