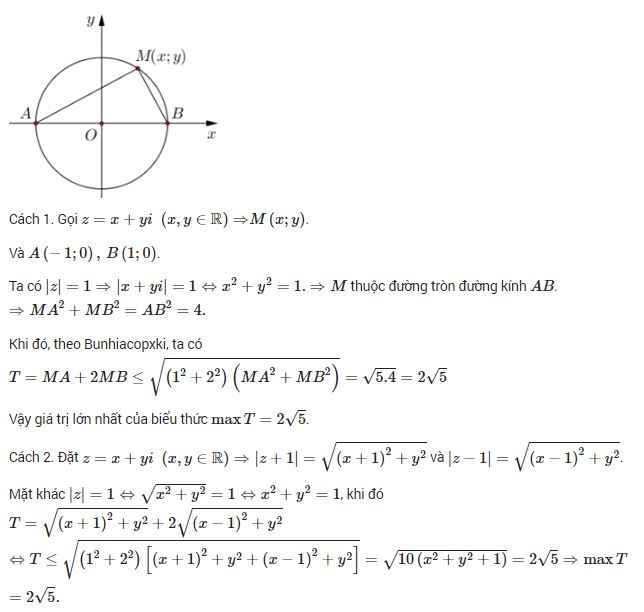
Đặt \(z=x+yi\Rightarrow x^2+y^2=1\)
\(P=\left|x+1+yi\right|+2\left|1-x-yi\right|=\sqrt{\left(x+1\right)^2+y^2}+2\sqrt{\left(1-x\right)^2+y^2}\)
\(=\sqrt{x^2+y^2+2x+1}+2\sqrt{x^2+y^2-2x+1}\)
\(=\sqrt{2x+2}+2\sqrt{-2x+2}\le\sqrt{\left(1^2+2^2\right)\left(2x+2-2x+2\right)}=2\sqrt{5}\)
\(\Rightarrow P_{max}=2\sqrt{5}\) khi \(2x+2=\frac{-2x+2}{4}\Rightarrow x=-\frac{3}{5}\)
Cho số phức z thoả mãn |\(z+\overline{z}+2\)| + \(2\left|z-\overline{z}-2i\right|\le12\). Gọi M, m lần lượt là giá trị lớn nhất, nhỏ nhất của biểu thức \(P=\left|z-4-4i\right|\). Tính M+ m
Cho số phức \(z\) thỏa mãn \(\left|z-3-4i\right|=\sqrt{5}\). Gọi \(M\) và \(m\) lần lượt là giá trị lớn nhất và giá trị nhỏ nhất của biểu thức \(P=\left|z+2\right|^2-\left|z-i\right|^2\). Môđun của số phức \(w=M+mi\) là?
Giải thích cho mình dòng bôi vàng ở dưới ạ, mình cảm ơn nhiều ♥
Cho số phức \(z\) thỏa mãn \(\left|z\right|=1\). Giá trị lớn nhất của biểu thức \(P=\left|1+z\right|+2\left|1-z\right|\) bằng?
Có cách nào chứng minh không cần dùng bất đẳng thức Bunyakovsky không ạ, mình cảm ơn nhiều♥
Cho N là điểm biểu diễn số phức z thỏa mãn \(\dfrac{z+2-3i}{z-3}=1-i\) và M là điểm biểu diễn số phức z' thoả mãn \(\left|z'-2-i\right|+\left|z'+3-3i\right|=\sqrt{29}\). Tìm giá trị nhỏ nhất của MN
Cho số phức z = a − 2 + (b + 1)i với a, b ∈ Z và |z| = 2. Tìm giá trị lớn nhất của biểu thức S = a + 2b.
Cho các số phức \(z_1\), \(z_2\) thoả mãn \(\left|z-2\right|=\left|z\right|\) và \(\left|z_2-z_1\right|=4\). Số phức \(w\) thoả mãn \(\left|w-3-5i\right|=1\), số phức \(u\) thoả mãn \(\left|u-4+4i\right|=2\). Giá trị nhỏ nhất của \(T=\left|w-z_2\right|+\left|u-z_1\right|\) là
A. \(5\sqrt{3}-3\) B. \(5\sqrt{2}-3\) C. \(2\sqrt{5}-3\) D. \(5\sqrt{3}-2\)
Cho \(z_1,z_2\) là hai số phức thoả mãn \(\left|z-4-3i\right|=2\) và \(\left|z_1-z_2\right|=3\). Giá trị lớn nhất của biểu thức \(M=\left|z_1+z_2-2+2i\right|\) là
Cho hai số phức \(z_1,z_2\) thỏa mãn \(\left|z_1+3+2i\right|=1\) và \(\left|z_2+2-i\right|=1\). Xét các số phức \(z=a+bi\), (\(a,b\in R\)) thỏa mãn \(2a-b=0\). Khi biểu thức \(T=\left|z-z_1\right|+\left|z-2z_2\right|\) đạt giá trị nhỏ nhất thì giá trị biểu thức \(P=a^2+b^2\) bằng?
Cho hai số phức \(z\) và \(w\) thay đổi thỏa mãn các điều kiện \(\left|z+1+i\right|=\left|z\right|\) và \(\left|w-3-4i\right|=1\). Tìm giá trị nhỏ nhất của biểu thức \(P=\left|z-w-1-i\right|\)
A.\(minP=5\sqrt{2}\) B. \(minP=5\sqrt{2}-1\) C. \(minP=3\sqrt{2}\) D. \(minP=3\sqrt{2}-1\)
Mình cần bài giải ạ, mình cảm ơn nhiều♥
