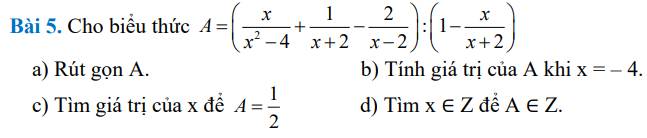Bài 5:
\(a,A=\left(\dfrac{x}{x^2-4}+\dfrac{1}{x+2}-\dfrac{2}{x-2}\right):\left(1-\dfrac{x}{x+2}\right)\left(ĐKXĐ:x\ne\pm2\right)\)
\(A=\left(\dfrac{x}{\left(x-2\right)\left(x+2\right)}+\dfrac{x-2}{\left(x-2\right)\left(x+2\right)}-\dfrac{2\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}\right):\left(\dfrac{x+2}{x+2}-\dfrac{x}{x+2}\right)\)
\(A=\left(\dfrac{x+x-2-2x-4}{\left(x-2\right)\left(x+2\right)}\right):\left(\dfrac{x+2-x}{x+2}\right)\)
\(A=\dfrac{-6}{\left(x-2\right)\left(x+2\right)}:\dfrac{2}{x+2}\)
\(A=\dfrac{-6}{\left(x-2\right)\left(x+2\right)}.\dfrac{x+2}{2}\)
\(A=\dfrac{-3}{x-2}\)
\(b,A=\dfrac{-3}{x-2}\left(ĐKXĐ:x\ne\pm2\right)\)
Với \(x=-4\left(tmĐKXĐ\right)\)
\(\Rightarrow A=\dfrac{-3}{-4-2}=\dfrac{-3}{-6}=\dfrac{1}{2}\)
\(c,A=\dfrac{-3}{x-2}\left(ĐKXĐ:x\ne\pm2\right)\)
\(A=\dfrac{1}{2}\)
\(\dfrac{-3}{x-2}=\dfrac{1}{2}\)
\(\Rightarrow x-2=-3.2\)
\(\Leftrightarrow x-2=-6\)
\(\Leftrightarrow x=-4\left(tm\right)\)
\(d,A=\dfrac{-3}{x-2}\left(ĐKXĐ:x\ne\pm2\right)\)
\(MàA\in Z\)
\(\Rightarrow x-2\inƯ\left(-3\right)=\left\{\pm1;\pm3\right\}\)
Xét bảng:
| \(x-2\) | \(1\) | \(-1\) | \(3\) | \(-3\) |
| \(x\) | \(3\) | \(1\) | \(5\) | \(-1\) |
Vậy \(x=\left\{;1;5;-1\right\}⇒A\in Z\)