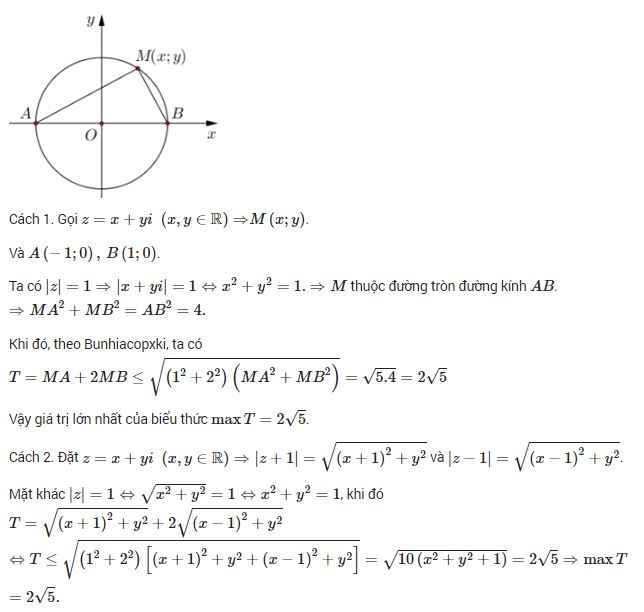
1. Cho số phức z thỏa mãn hệ thức | z-1+i | = | z-2-3i |. Tìm giá trị nhỏ nhất của biểu thức P = | z+2+i | + | z-3+2i |
2. Cho số phức z thỏa mãn hệ thức | z-i | = 2. Biết rằng | z | lớn nhất. Tìm phần ảo của z
3. Cho số phức z thỏa \(\overline{z}=\left(i+\sqrt{2}\right)^2\left(1-\sqrt{2}i\right)\). Tìm phần ảo của số phức z
4. Cho 2 số phức z = m + 3i, z' = 2 - (m + 1)i. Tìm giá trị thực của m để z.z' là số thực
5. Cho 3 điểm A, B, M lần lượt biểu diễn các số phức -4, 4i, x + 3i. Với giá trị thực nào của x thì A, B, M thẳng hàng?
6. Cho 2 số phức \(z_1=1+2i\), \(z_2=2-3i\). Xác định phần ảo của số phức \(3z_1-2z_2\)
7. Nếu mô đun số phức z bằng m thì mô đun của số phức \(\left(1-i\right)^2z\) bằng?
8. Trong tất cả các số phức z thỏa mãn hệ thức | z-1+3i | = 3. Tìm min | z-1-i |
9. Trong mặt phẳng phức tìm điểm biểu diễn số phức z = \(\frac{i^{2017}}{3+4i}\)
10. Trong mặt phẳng phức với hệ trục tọa độ Oxy, điểm biểu diễn của các số phức z = 3 + bi với b \(\in\) R luôn nằm trên đường có phương trình là: A. y = x B. x = 3 C. y = x + 3 D. y = 3
11. Cho 2 số phức \(z_1=1+2i\), \(z_2=2-3i\). Tổng hai số phức là?
12. Cho số phức z = 2 + 5i. Tìm số phức \(w=iz+\overline{z}\)
13. Ký hiệu \(z_0\) là nghiệm phức có phần ảo âm của phương trình \(z^2+z+1=0\). Tìm trên mặt phẳng tọa độ điểm nào dưới đây là điểm biểu diễn số phức \(w=\frac{i}{z_0}\): A. \(M\left(-\frac{\sqrt{3}}{2};-\frac{1}{2}\right)\) B. \(M\left(-\frac{\sqrt{3}}{2};\frac{1}{2}\right)\) C. \(M\left(\frac{\sqrt{3}}{2};-\frac{1}{2}\right)\) D. \(M\left(-\frac{1}{2};-\frac{\sqrt{3}}{2}\right)\)
14. Cho số phức z thỏa mãn hệ thức | z+7-5i | = | z-1-11i |. Biết rằng số phức z = x + yi thỏa mãn \(\left|z-2-8i\right|^2+\left|z-6-6i\right|^2\) đạt giá trị nhỏ nhất. Giá trị của biểu thức \(p=x^2-y^2\)?
15. Gọi \(z_0\) là nghiệm phức có phần ảo âm của phương trình \(2z^2-6z+5=0\). Điểm nào sau đây biểu diễn số phức \(iz_0\): A. \(M\left(\frac{3}{2};\frac{1}{2}\right)\) B. \(M\left(\frac{3}{2};-\frac{1}{2}\right)\) C. \(M\left(-\frac{1}{2};\frac{3}{2}\right)\) D. \(M\left(\frac{1}{2};\frac{3}{2}\right)\)
16. Tính mô đun của số phức \(w=z^2+i\overline{z}\) biết z thỏa mãn \(\left(1+2i\right)z+\left(2+3i\right)\overline{z}=6+2i\)
17. Trong mặt phẳng phức, cho 3 điểm A, B, C lần lượt biểu diễn 3 số phức \(z_1=1+i\), \(z_2=\left(1+i\right)^2\), \(z_3=a-i\left(a\in R\right)\). Để tam giác ABC vuông tại B thì A bằng? A. -3 B. 3 C. -4 D. -2
18. Cho số phức z thỏa mãn (1+2i)z = 3+i. Tính giá trị biểu thức \(\left|z\right|^4-\left|z\right|^2+1\)
19. Cho số phức z = a + (a-1)i (a\(\in R\)). Giá trị thực nào của a để | z | = 1 ?
20. Cho số phức z thoả mãn hệ thức | z+5-i | = | z+1-7i |. Tìm giá trị lớn nhất của biểu thức P = | |z-4-i| - |z-2-4i| |
21. Trong các số phức z = a + bi thỏa mãn | z-1+2i | =1, biết rằng | z+3-i | đạt giá trị nhỏ nhất. Tính \(p=\frac{a}{b}\)
22. Gọi A, B, C lần lượt là các điểm biểu diễn các số phức \(z_1=-1+3i\), \(z_2=-3-2i\), \(z_3=4+i\). Chọn kết luận đúng nhất: A. Tam giác ABC cân B. Tam giác ABC đều C. Tam giác ABC vuông D. Tam giác ABC vuông cân
23. Cho số phức z = 5-3i. Tính \(1+\overline{z}+\left(\overline{z}\right)^2\)
24. Cho \(f\left(z\right)=z^3-3z^2+z-1\) với z là số phức. Tính \(f\left(z_0\right)-f\left(\overline{z_0}\right)\) biết \(z_0=1-2i\)
25. Cho số phức z thỏa mãn iz + 2 - i = 0. Khoảng cách từ điểm biểu diễn của z trên mặt phẳng tọa độ Oxy đến điểm M (3;-4) là: A. \(\sqrt{13}\) B. \(2\sqrt{2}\) C. \(2\sqrt{5}\) D. \(2\sqrt{10}\)