Question

Answer 1

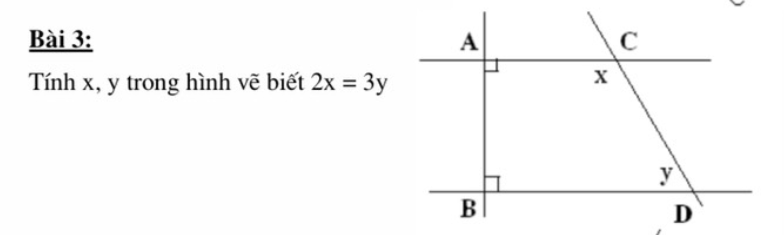
Ta có: AC⊥AB, BD⊥AB

=> AC//BD => \(x+y=180^0\)(trong cùng phía)

Ta có: 

\(2x=3y\Rightarrow\dfrac{x}{3}=\dfrac{y}{2}\)

Áp dụng t/c dtsbn:

\(\dfrac{x}{3}=\dfrac{y}{2}=\dfrac{x+y}{3+2}=\dfrac{180}{5}=36\)

\(\Rightarrow\left\{{}\begin{matrix}x=36.3=108^0\\y=36.2=72^0\end{matrix}\right.\)

Answer 2

Ta có: \(x+y=180^0\) (2 góc trong cùng phía)

Mà \(2x=3y\)

\(\Rightarrow x=108^0\)

\(\Rightarrow y=72^0\)

Answer 3

Ta có: \(x+y=180\) (2 góc phụ nhau)

\(\Leftrightarrow2x+2y=360\\ \Leftrightarrow3y+2y=360\\ \Leftrightarrow5y=360\\ \Leftrightarrow y=72\)

Thay y=72 vào 2x=3y ta được

\(2x=3.72\\ \Leftrightarrow x=108\)