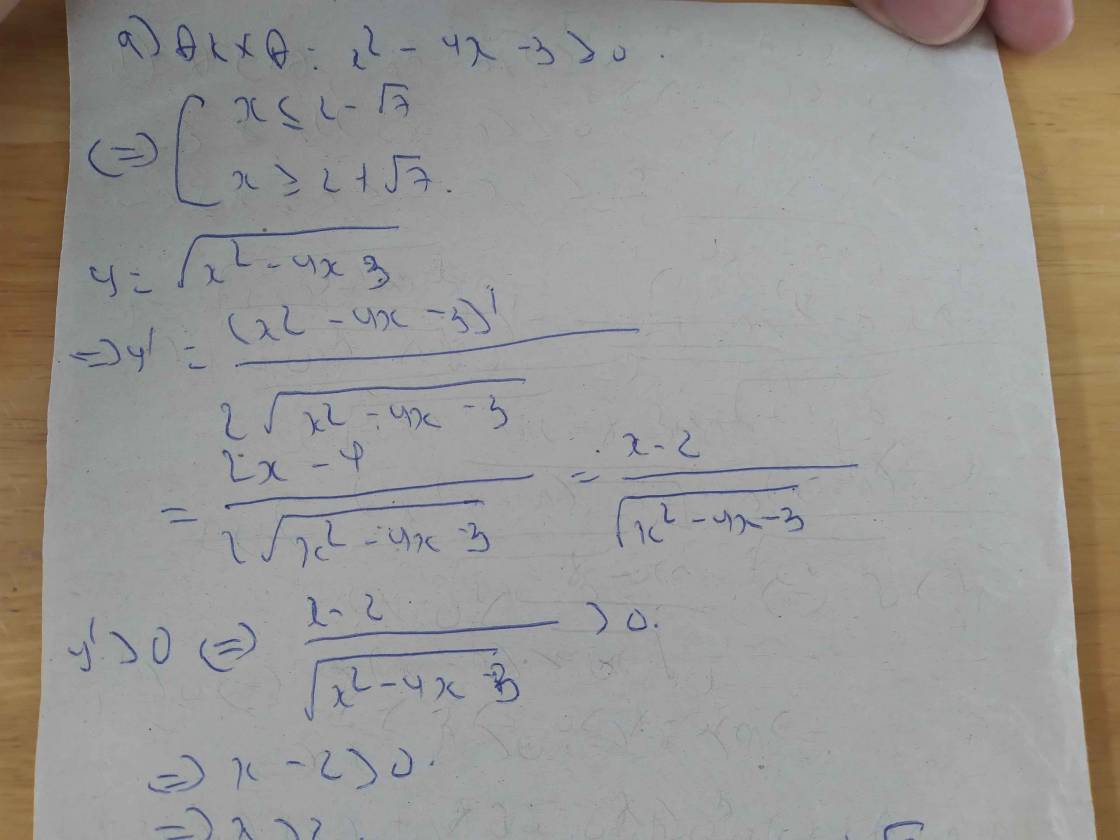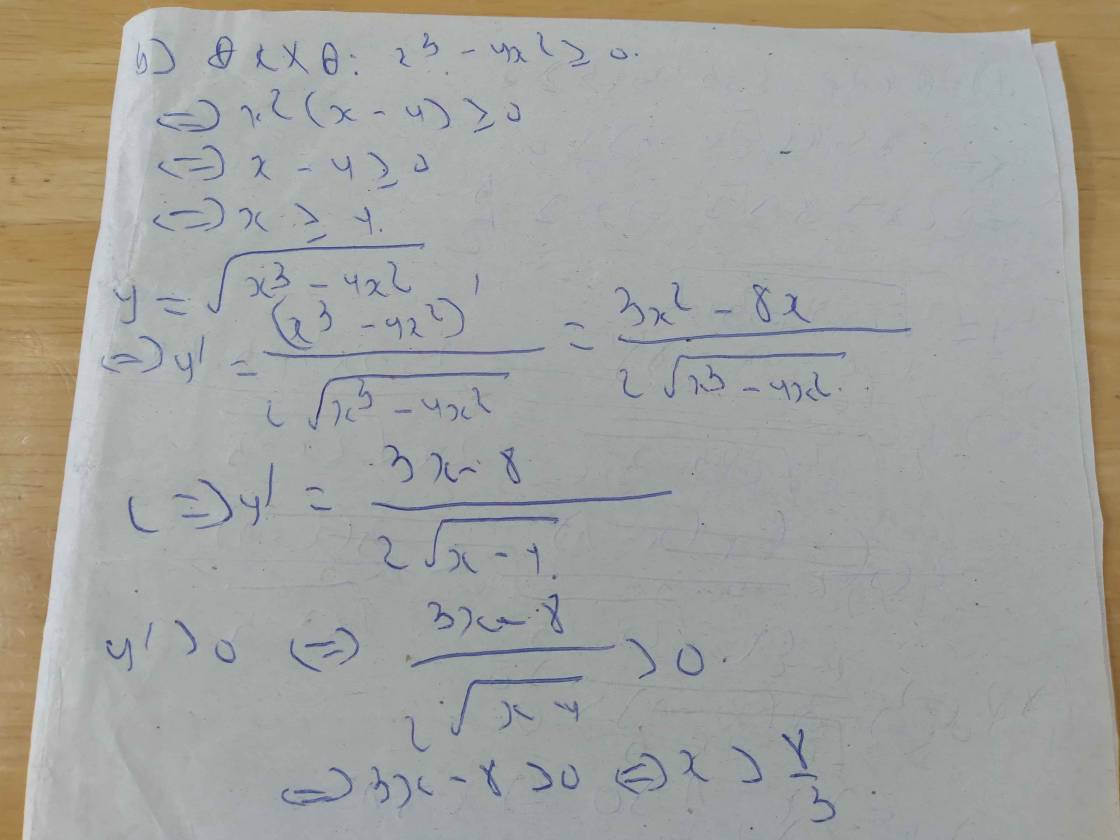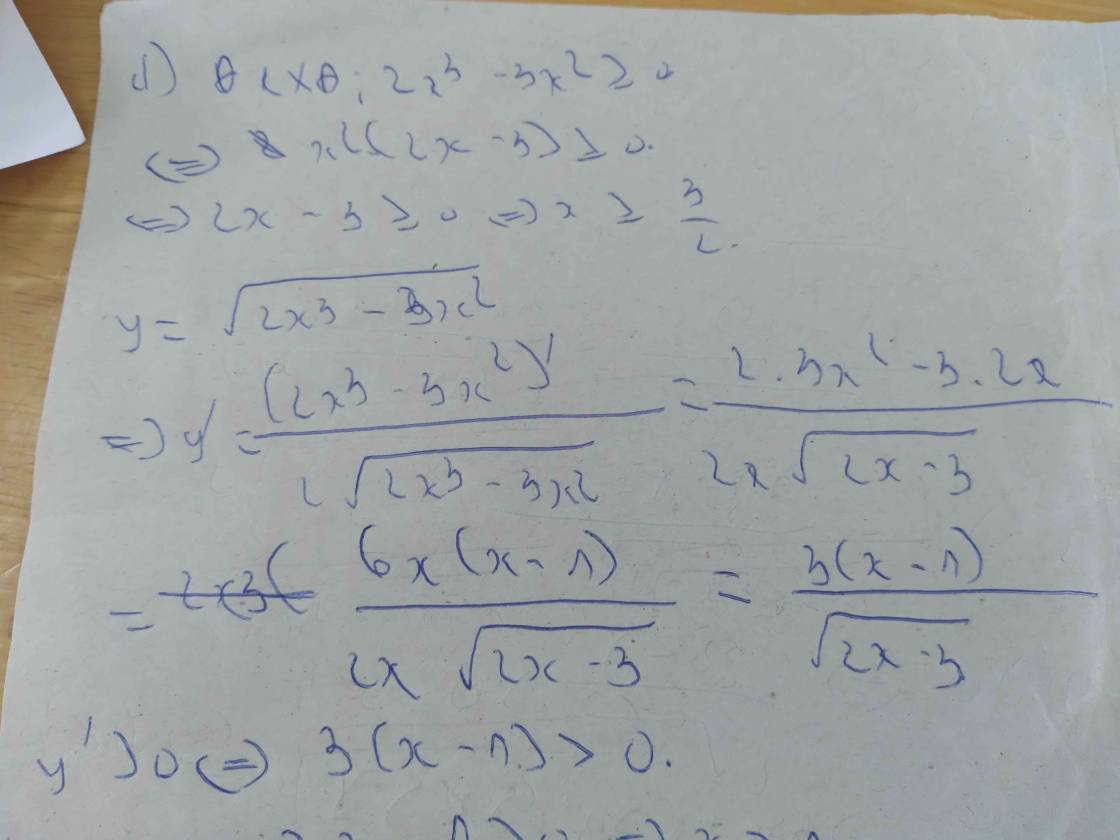\(\left(4x^2-3x-7\right)\left(2x+3\right)\)LÀm tính nhân

Những câu hỏi liên quan
Tính:
\(a)\left(-2x^2\right)\cdot\left(3x-4x^3+7-x^2\right)\)
\(b)\left(x+3\right)\cdot\left(2x^2-3x-5\right)\)
\(c)\left(-6x^5+7x^4-6x^3\right):3x^3\)
\(d)\left(9x^2-4\right):\left(3x+2\right)\)
\(e)\left(2x^4-13x^3+15x^2+11x-3\right):\left(x^2-4x-3\right)\)
a: \(=-2x^2\cdot3x+2x^2\cdot4X^3-2x^2\cdot7+2x^2\cdot x^2\)
\(=8x^5+2x^4-6x^3-14x^2\)
b: \(=2x^3-3x^2-5x+6x^2-9x-15\)
\(=2x^3+3x^2-14x-15\)
c: \(=\dfrac{-6x^5}{3x^3}+\dfrac{7x^4}{3x^3}-\dfrac{6x^3}{3x^3}=-2x^2+\dfrac{7}{3}x-2\)
d: \(=\dfrac{\left(3x-2\right)\left(3x+2\right)}{3x+2}=3x-2\)
e: \(=\dfrac{2x^4-8x^3-6x^2-5x^3+20x^2+15x+x^2-4x-3}{x^2-4x-3}\)
=2x^2-5x+1
Đúng 2
Bình luận (0)
rút gọn biểu thức
\(\left(x^2-1\right)\left(x+2\right)-\left(x-4\right)\left(x^2+4x+16\right)\)
làm tính nhân
\(2x\left(3x-2\right)^2\)
\(\left(x-3\right)\left(x^2-3x+9\right)\)
\(\left(x^2-1\right)\left(x+2\right)-\left(x-4\right)\left(x^2+4x+16\right)\)
\(=x^3+2x^2-x-2-\left(x^3-4^3\right)\)
\(=x^3+2x^2-x-2-x^3+64\)
\(=2x^2-x+62\)
\(2x\left(3x-2\right)^2\)
\(=2x\left(9x^2-12x+4\right)\)
\(=18x^3-24x^2+8x\)
\(\left(x-3\right)\left(x^2-3x+9\right)\)
\(=x^3-3x^2+9x-3x^2+9x-27\)
\(=x^3-3x^2+18x-27\)
Đúng 0
Bình luận (0)
\(\left(x^2-1\right)\left(x+2\right)-\left(x-4\right)\left(x^2+4x+16\right)\)
\(=\left(x^2-1^2\right)\left(x+2\right)-x^3-4^3\)
\(=\left(x+1\right)\left(x-1\right)\left(x+2\right)-x^3-64\)
Đúng 0
Bình luận (0)
TìmATRONG mỗi phân thức PHÂN THỨC SAUfrac{4x^2-3x-7}{A}frac{4x-7}{2x+3}.giải. Ta có : left(4x^2-3x-7right)left(2x+3right)A.left(4x-7right)Hayleft(4x^2-7x+4x-7right)left(2x+3right)A.left(4x-7right).Hayleft(4x-7right)left(x+1right)left(2x+3right)A.left(4x-7right).VậyAleft(x+1right)left(2x+3right)2x^2+5x+3.Cô ơi, ở dòng hay thứ 2, chỗ : left(x+1right)left(2x+3right)từ đâu có vậy cô ? (cp6 làm, phân tích chi tiết giúp em nhe cô). Em cám ơn cô. :)
Đọc tiếp
\(Tìm\)\(A\)TRONG mỗi phân thức PHÂN THỨC SAU
\(\frac{4x^2-3x-7}{A}=\frac{4x-7}{2x+3}.\)
giải. Ta có : \(\left(4x^2-3x-7\right)\left(2x+3\right)=A.\left(4x-7\right)\)
\(Hay\)\(\left(4x^2-7x+4x-7\right)\left(2x+3\right)=A.\left(4x-7\right).\)
\(Hay\)\(\left(4x-7\right)\left(x+1\right)\left(2x+3\right)=A.\left(4x-7\right).\)
\(Vậy\)\(A=\left(x+1\right)\left(2x+3\right)=2x^2+5x+3.\)
Cô ơi, ở dòng hay thứ 2, chỗ : \(\left(x+1\right)\left(2x+3\right)\)từ đâu có vậy cô ? (cp6 làm, phân tích chi tiết giúp em nhe cô). Em cám ơn cô. :)
này như thế này phải không
(4x2+4x-7x-7)(2x+3)= 4x(x+1)-7(x+1)= (4x-7)(x+1)
Đúng 0
Bình luận (0)
Tìm số nguyên x
1/ 17x+3left(-16x-37right)2x+43-4x
2/ -2x-3left(x-17right)34-2left(-x+25right)
3/ left{-3x+2left[45-x-3left(3x+7right)-2xright]+4xright}55-103-57:left[-2left(2x-1right)^2-left(-9right)^0right]-106
4/ -2x+3left{12-2left[3x-left(20+2xright)-4xright]+1right}45
5/ 3x-32-5+1
6/ 15+4x 2x-145
7/ -3left(2x+5right)-16 -4left(3-2xright)
8/ -2x+15 3x-7 19-x
Đọc tiếp
Tìm số nguyên x
1/ \(17x+3\left(-16x-37\right)=2x+43-4x\)
2/ \(-2x-3\left(x-17\right)=34-2\left(-x+25\right)\)
3/ \(\left\{-3x+2\left[45-x-3\left(3x+7\right)-2x\right]+4x\right\}=55-103-57:\left[-2\left(2x-1\right)^2-\left(-9\right)^0\right]=-106\)
4/ \(-2x+3\left\{12-2\left[3x-\left(20+2x\right)-4x\right]+1\right\}=45\)
5/ \(3x-32>-5+1\)
6/ \(15+4x< 2x-145\)
7/ \(-3\left(2x+5\right)-16< -4\left(3-2x\right)\)
8/ \(-2x+15< 3x-7< 19-x\)
bài tập tết nâng cao phải ko
mk cũng có nhưng chưa làm dc
Đúng 0
Bình luận (0)
a)làm tính nhân: (x+2)\(\left(x^2+3x+1\right)\)
b)Làm tính chia: \(\left(2x^3+10x^2+9x+4\right):\left(x+4\right)\)
a) (x + 2)(x2 + 3x + 1)
= x.x2 + x.3x + x.1 + 2.x2 + 2.3x + 2.1
= x3 + 3x2 + x + 2x2 + 6x + 2
= x3 + 5x2 + 7x + 2
b) (2x3 + 10x2 + 9x + 4) : (x + 4)
= (2x3 + 8x2 + 2x2 + 8x + x + 4) : (x + 4)
= [(2x3 + 8x2) + (2x2 + 8x) + (x + 4)] : (x + 4)
= [2x2(x + 4) + 2x(x + 4) + (x + 4)] : (x + 4)
= (x + 4)(2x2 + 2x + 1) : (x + 4)
= 2x2 + 2x + 1
Đúng 1
Bình luận (0)
Bằng cách phân tích vế trái thành nhân tử, giải các PT sau:
d) \(x\left(2x-7\right)-4x+14=0\)
e) \(\left(2x-5\right)^2-\left(x+2\right)^2=0\)
f) \(x^2-x-\left(3x-3\right)=0\)
d) \(PT\Leftrightarrow x\left(2x-7\right)-4\left(x-7\right)=0\)
\(\Leftrightarrow\left(2x-7\right)\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-7=0\\x-4=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=4\end{matrix}\right.\)
Vậy: \(S=\left\{\dfrac{7}{2};4\right\}\)
e) \(PT\Leftrightarrow\left(2x-5-x-2\right)\left(2x-5+x+2\right)=0\)
\(\Leftrightarrow\left(x-7\right)\left(3x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-7=0\\3x-3=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=7\\x=1\end{matrix}\right.\)
Vậy: \(S=\left\{7;1\right\}\)
f) \(PT\Leftrightarrow x\left(x-1\right)-3\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x-3=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=3\end{matrix}\right.\)
Vậy: \(S=\left\{1;3\right\}\)
Đúng 2
Bình luận (0)
\(d,x\left(2x-7\right)-4x+14=0\)
\(x\left(2x-7\right)-2\left(2x-7\right)=0\)
\(\left(x-2\right)\left(2x-7\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=\dfrac{7}{2}\end{matrix}\right.\)
Đúng 2
Bình luận (0)
d: =>(2x-7)(x-2)=0
=>x=7/2 hoặc x=2
e: =>(2x-5-x-2)(2x-5+x+2)=0
=>(x-7)(3x-3)=0
=>x=7 hoặc x=1
f: =>x(x-1)-3(x-1)=0
=>(x-1)(x-3)=0
=>x=1 hoặc x=3
Đúng 1
Bình luận (0)
Xem thêm câu trả lời
Phân tích các đa thức sau thành nhân tử:
\(A=4x^2+6x\). \(B=\left(2x+3\right)^2-x\left(2x+3\right)\). \(C=\left(9x^2-1\right)-\left(3x-1\right)^2\).
\(D=x^3-16x\). \(E=4x^2-25y^2\). \(G=\left(2x+3\right)^2-\left(2x-3\right)^2\).
\(A=4x^2+6x=2x\left(2x+3\right)\)
\(B=\left(2x+3\right)^2-x\left(2x+3\right)=\left(2x+3\right)\left(2x+3-x\right)=\left(2x+3\right)\left(x+3\right)\)
\(C=\left(9x^2-1\right)-\left(3x-1\right)^2=\left(3x-1\right)\left(3x+1\right)-\left(3x-1\right)^2=\left(3x-1\right)\left(3x+1-3x+1\right)=2\left(3x+1\right)\)
\(D=x^3-16x=x\left(x^2-16\right)=x\left(x-4\right)\left(x+4\right)\)
\(E=4x^2-25y^2=\left(2x-5y\right)\left(2x+5y\right)\)
\(G=\left(2x+3\right)^2-\left(2x-3\right)^2=\left(2x+3-2x+3\right)\left(2x+3+3x-3\right)=6.4x=24x\)
Đúng 2
Bình luận (0)
\(A=2x\left(2x+3\right)\\ B=\left(2x+3\right)\left(2x+3-x\right)=\left(2x+3\right)\left(x+3\right)\\ C=\left(3x-1\right)\left(3x+1\right)-\left(3x-1\right)^2\\ =\left(3x-1\right)\left(3x+1-3x+1\right)\\ =2\left(3x-1\right)\\ D=x\left(x^2-16\right)=x\left(x-4\right)\left(x+4\right)\\ E=\left(2x-5y\right)\left(2x+5y\right)\\ G=\left(2x+3-2x+3\right)\left(2x+3+2x-3\right)\\ =24x\)
Đúng 2
Bình luận (0)
xét tính đồng biến nghịch biến
a) \(y=\sqrt{x^2-4x-3}\)
b) \(y=\sqrt{x^3-4x^2}\)
c) \(y=\left(2x+3\right)^{12}\left(6-5x\right)^9\left(x-7\right)^5\)
d) \(y=\sqrt{2x^3-3x^2}\)
Tìm x, biết :
a, \(\left(3x+2\right).\left(6x-2\right)-\left(9x-2\right).\left(2x+1\right)=24\)
b, \(\left(4x+3\right)\left(3x-2\right)-\left(6x-1\right)\left(2x+3\right)=16\)
c, \(\left(5x-2\right)\left(4x+5\right)+\left(10x-7\right)\left(5-2x\right)=12\)
d, \(6x\left(3-4x\right)+8x\left(3x-2\right)=16\)